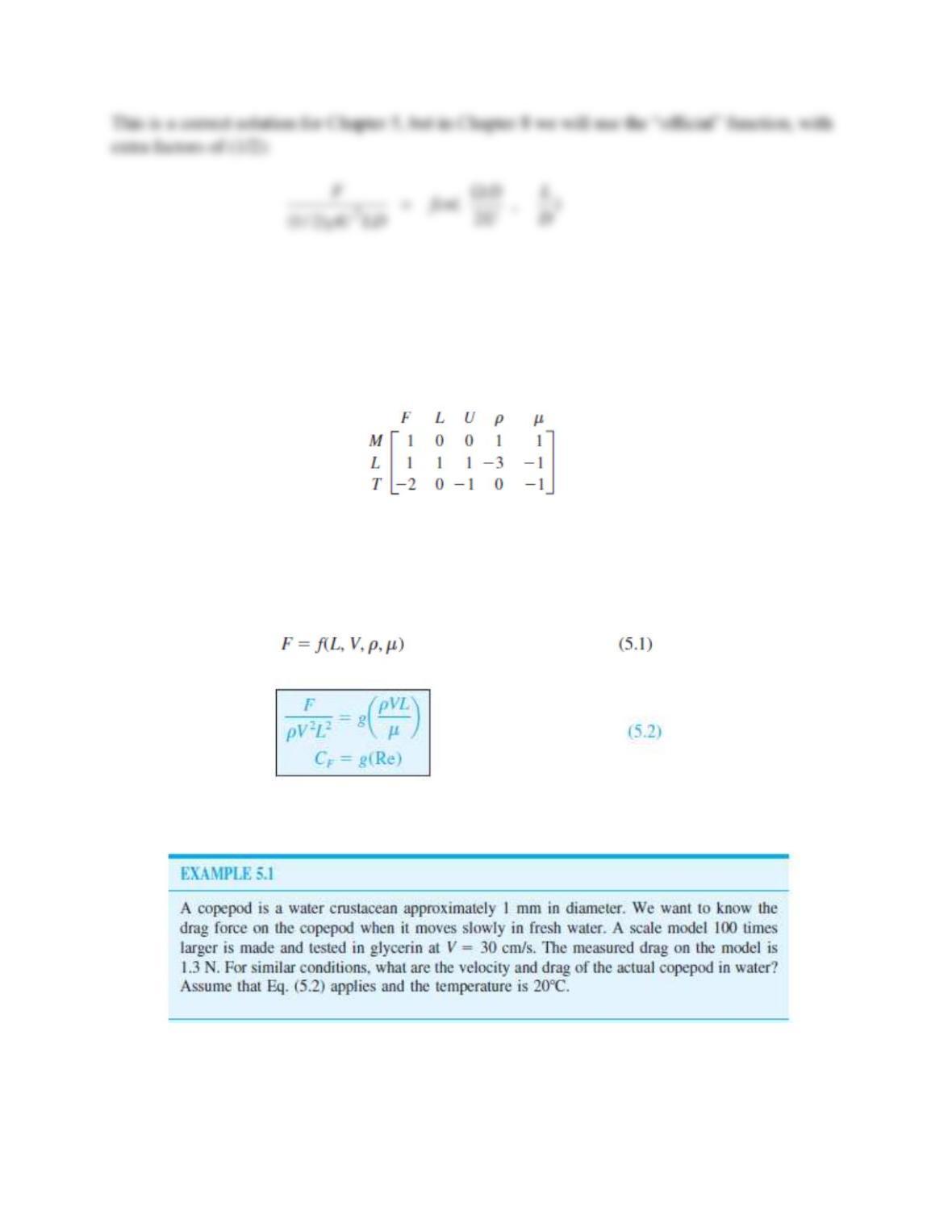
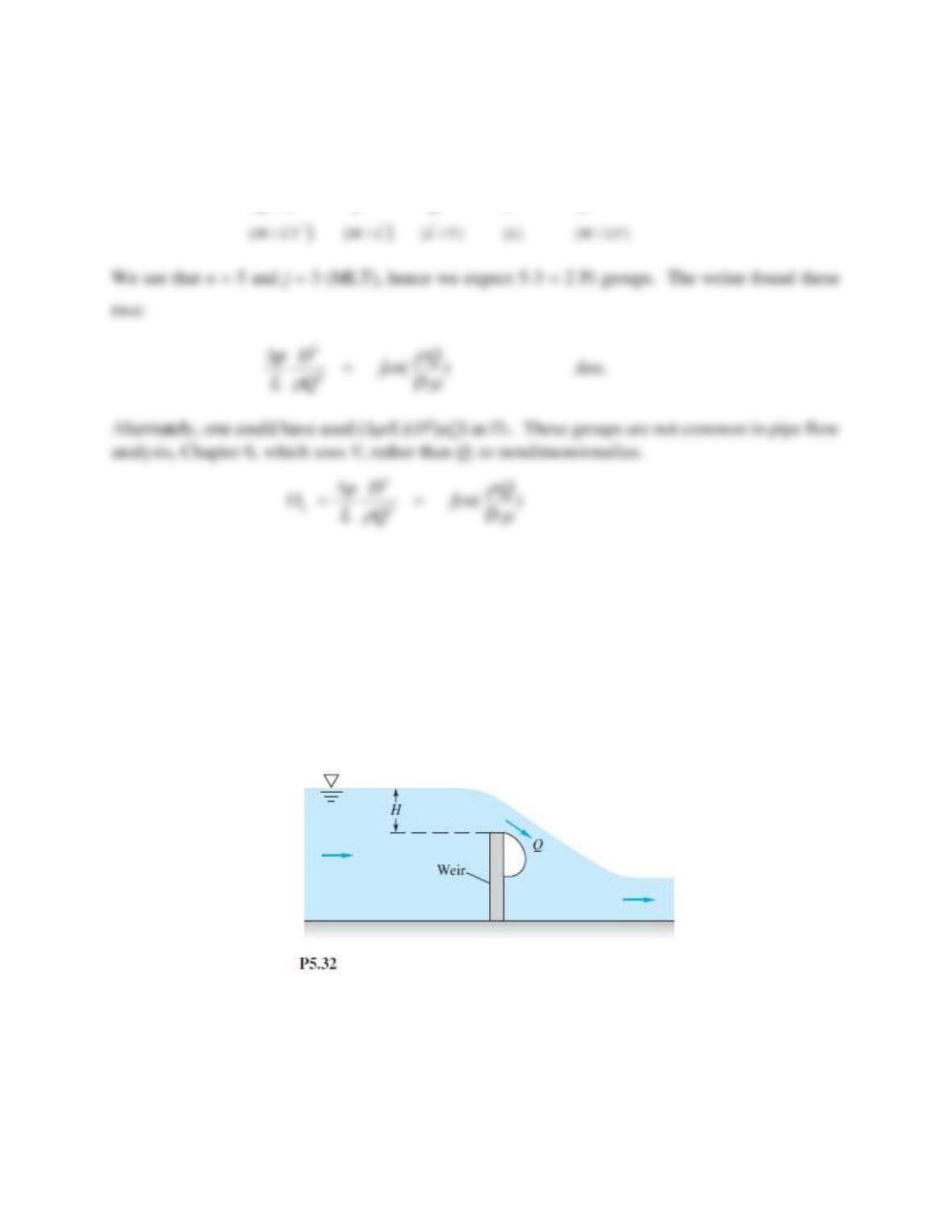
Problem 5.21
In Example 5.1 we used the pi theorem to develop Eq. (5.2) from Eq. (5.1). Instead of merely listing
the primary dimensions of each variable, some workers list the powers of each primary dimension
for each variable in an array:
This array of exponents is called the dimensional matrix for the given function. Show that the rank
of this matrix (the size of the largest nonzero determinant) is equal to j = n – k, the desired reduction
between original variables and the pi groups. This is a general property of dimensional matrices, as
noted by Buckingham [1].
Solution 5.21
Problem 5.22
As will be discussed in Chapter 11, the power P developed by a wind turbine is a function of
diameter D, air density ρ, wind speed V, and rotation rate ω. Viscosity effects are negligible.
Rewrite this relationship in dimensionless form.
Solution 5.22
Write the function and the dimensions of the variables:
Problem 5.23
The period T of vibration of a beam is a function of its length L, area moment of inertia I, modulus
of elasticity E, density
, and Poisson’s ratio
. Rewrite this relation in dimensionless form. What
further reduction can we make if E and I can occur only in the product form EI?
Hint: Take L , ρ , and E as repeating variables.
Solution 5.23
Establish the variables and their dimensions:
L=
Problem 5.24
The lift force F on a missile is a function of its length L, velocity V, diameter D, angle of attack
density
, viscosity
, and speed of sound a of the air. Write out the dimensional matrix of this
function and determine its rank. (See Prob. 5.21 for an explanation of this concept.) Rewrite the
function in terms of pi groups.
Solution 5.24
Establish the variables and their dimensions:
Problem 5.25
The thrust F of a propeller is generally thought to be a function of its diameter D and angular
velocity
, the forward speed V, and the density
and viscosity
of the fluid. Rewrite this
relationship as a dimensionless function.
Solution 5.25
Write out the function with the various dimensions underneath:
Problem 5.26
A pendulum has an oscillation period T which is assumed to depend upon its length L, bob mass
m, angle of swing
, and the acceleration of gravity. A pendulum 1 m long, with a bob mass of
200 g, is tested on earth and found to have a period of 2.04 s when swinging at 20. (a) What is its
period when it swings at 45? A similarly constructed pendulum, with L = 30 cm and m = 100 g,
is to swing on the moon (g = 1.62 m/s2) at
= 20. (b) What will be its period?
Solution 5.26
First establish the variables and their dimensions so that we can do the numbers:
T = fcn( L , m , g ,
)
{T} {L} {M} {L/T2} {1}
Problem 5.27
In studying sand transport by ocean waves, A. Shields in 1936 postulated that the bottom threshold
wave-induced bottom shear stress
required to move particles depends upon gravity g, particle size
d and density
p, and water density
and viscosity
. Find suitable dimensionless groups of this
problem, which resulted in the celebrated Shields sand transport diagram.
Solution 5.27
There are six variables (
, g, d,
p,
,
) and three dimensions (M, L, T), hence we expect
Problem 5.28
A simply supported beam of diameter D, length L, and modulus of elasticity E is subjected to a fluid
crossflow of velocity V, density
, and viscosity
. Its center deflection
is assumed to be a
function of all these variables. (a) Rewrite this proposed function in dimensionless form. (b) Suppose
it is known that
is independent of
, inversely proportional to E, and dependent only upon
V
2, not
and V separately. Simplify the dimensionless function accordingly. Hint: Take L,
, and V as repeating
variables.
Solution 5.28
Establish the variables and their dimensions:
= fcn(
, D , L , E , V ,
)
Problem 5.29
When fluid in a pipe is accelerated linearly from rest, it begins as laminar flow and then undergoes
transition to turbulence at a time ttr which depends upon the pipe diameter D, fluid acceleration a,
density
, and viscosity
. Arrange this into a dimensionless relation between ttr and D.
Solution 5.29
Establish the variables and their dimensions:
Problem 5.30
When a large tank of high-pressure gas discharges through a nozzle, the exit mass flow
m
is a
function of tank pressure po and temperature To, gas constant R, specific heat cp, and nozzle
diameter D. Rewrite this as a dimensionless function. Check to see if you can use (po, To , R, D)
as repeating variables.
Solution 5.30
Using Table 5.1, write out the dimensions of the six variables:
Problem 5.31
The pressure drop per unit length in horizontal pipe flow, Δp/L, depends on the fluid density ρ,
viscosity μ, diameter D, and volume flow rate Q. Rewrite this function in terms of pi groups.
Solution 5.31
First write out the dimension s of the variables:
/
p L Q D
Problem 5.32
A weir is an obstruction in a channel flow which can be calibrated to measure the flow rate, as in
Fig. P5.32. The volume flow Q varies with gravity g, weir width b into the paper, and upstream
water height H above the weir crest. If it is known that Q is proportional to b, use the pi theorem to
find a unique functional relationship Q(g, b, H).
Solution 5.32
Establish the variables and their dimensions:
Q = fcn( g , b , H )
Problem 5.33
A spar buoy (see Prob. 2.113) has a period T of vertical (heave) oscillation that depends on the
waterline cross-sectional area A, buoy mass m, and fluid specific weight
. How does the period
change due to doubling of (a) the mass and (b) the area? Instrument buoys should have long periods
to avoid wave resonance. Sketch a possible long-period buoy design.
Solution 5.33
Establish the variables and their dimensions:
Problem 5.34
To good approximation, the thermal conductivity k of a gas (see Ref. 21 of Chap. 1) depends only
on the density
, mean free path
,
gas constant R, and absolute temperature T. For air at 20C and
1 atm, k 0.026 W/mK and 6.5E−8 m. Use this information to determine k for hydrogen at
20C and 1 atm if 1.2E−7 m.
Solution 5.34
First establish the variables and their dimensions and then form a pi group:
Problem 5.35
The torque M required to turn the cone-plate viscometer in Fig. P5.35 depends upon the radius R,
rotation rate , fluid viscosity
, and cone angle
. Rewrite this relation in dimensionless form. How
does the relation simplify if it is known that M is proportional to
?
Solution 5.35
Establish the variables and their dimensions:
Problem 5.36
The rate of heat loss
Q
loss through a window or wall is a function of the temperature difference
between inside and outside ΔT , the window surface area A , and the R value of the window,
which has units of (ft2 · h · °F)/Btu. (a) Using the Buckingham Pi Theorem, find an expression
for rate of heat loss as a function of the other three parameters in the problem. (b) If the
temperature difference ΔT doubles, by what factor does the rate of heat loss increase?
Solution 5.36
First figure out the dimensions of R: {R} = {T3/M}. Then note that n = 4 variables and
Problem 5.37
The volume flow Q through an orifice plate is a function of pipe diameter D, pressure drop p
across the orifice, fluid density
and viscosity
, and orifice diameter d. Using D,
, and p as
repeating variables, express this relationship in dimensionless form.
Solution 5.37
There are 6 variables and 3 primary dimensions (MLT), and we already know that j = 3,
because the problem thoughtfully gave the repeating variables. Use the pi theorem to find the
three pi’s:
Problem 5.38
The size d of droplets produced by a liquid spray nozzle is thought to depend upon the nozzle
diameter D, jet velocity U, and the properties of the liquid
,
, and Y. Rewrite this relation in
dimensionless form. Hint: Take D,
, and U as repeating variables.
Solution 5.38
Establish the variables and their dimensions:
Problem 5.39
The volume flow Q over a certain dam is a function of dam width b, gravity g, and the upstream
water depth H above the dam crest. It is known that Q is proportional to b. If b = 120 ft and
H = 15 inches, the flow rate is 600 ft3/s. What will be the flow rate if H = 3 ft?
Solution 5.39
Work this problem in BG units. Given that
Qb
, use dimensional analysis:
Problem 5.40
The time td to drain a liquid from a hole in the bottom of a tank is a function of the hole diameter
d, the initial fluid volume o, the initial liquid depth ho, and the density
and viscosity
of the
fluid. Rewrite this relation as a dimensionless function, using Ipsen’s method.
Solution 5.40
As asked, use Ipsen’s method. Write out the function with the dimensions beneath:
Problem 5.41
A certain axial-flow turbine has an output torque M which is proportional to the volume flow rate Q
and also depends upon the density
, rotor diameter D, and rotation rate . How does the torque
change due to a doubling of (a) D and (b) ?
Solution 5.41
List the variables and their dimensions, one of which can be MQ, since M is stated to be
proportional to Q:
Problem 5.42
When disturbed, a floating buoy will bob up and down at frequency f. Assume that this
frequency varies with buoy mass m and waterline diameter d and with the specific weight
of
the liquid. (a) Express this as a dimensionless function. (b) If d and
are constant and the buoy
mass is halved, how will the frequency change?
Solution 5.42
The proposed function is f = fcn( m, d,
). Write out their dimensions:
Problem 5.43
Nondimensionalize the thermal energy equation (4.75) and its boundary conditions (4.62), (4.63), and
(4.70) by defining dimensionless temperature
o
T* T/T=
, where To is the inlet temperature, assumed
constant. Use other dimensionless variables as needed from Eqs. (5.23). Isolate all dimensionless
parameters you find, and relate them to the list given in Table 5.2.
See Table 5.2 on the next page (Dimensionless Groups in Fluid Mechanics)
Solution 5.43
Recall the previously defined variables in addition to
T*
:
u x Ut v or w y or z
u* ; x* ; t* ; similarly, v* or w* ; y* or z*
U L L U L
= = = = =
Problem 5.44
The differential energy equation for incompressible two–dimensional flow through a “Darcy–type”
porous medium is approximately
2
20
pp
p T p T T
c c k
x x y y y
+ + =
where
is the permeability of the porous medium. All other symbols have their usual meanings.
(a) What are the appropriate dimensions for
? (b) Nondimensionalize this equation, using
(L, U,
, To) as scaling constants, and discuss any dimensionless parameters which arise.
Solution 5.44
(a) The only way to establish {
} is by comparing two terms in the PDE:
Problem 5.45
A model differential equation, for chemical reaction dynamics in a plug reactor, is as follows:
2
2
C C C
u kC
xt
x
= − −D
where u is the velocity, is a diffusion coefficient, k is a reaction rate, x is distance along the
reactor, and C is the (dimensionless) concentration of a given chemical in the reactor.
(a) Determine the appropriate dimensions of and k. (b) Using a characteristic length scale L and
average velocity V as parameters, rewrite this equation in dimensionless form and comment on any
pi groups appearing.
Solution 5.45
(a) Since all terms in the equation contain C, we establish the dimensions of k and by
comparing {k} and {
2/
x2} to {u
/
x}:
Problem 5.46
If a vertical wall at temperature Tw is surrounded by a fluid at temperature To, a natural
convection boundary layer flow will form. For laminar flow, the momentum equation is
2
2
( ) ( )
o
u u u
u v T T g
xy y
+ = − +
to be solved, along with continuity and energy, for (u, v, T) with appropriate boundary
conditions. The quantity
is the thermal expansion coefficient of the fluid. Use
, g, L, and
(Tw – To) to nondimensionalize this equation. Note that there is no “stream” velocity in this type
of flow.
Solution 5.46
For the given constants used to define dimensionless variables, there is only one pairing which
will give a velocity unit: (gL)1/2. Here are the writer’s dimensionless variables:
Problem 5.47
The differential equation for small-amplitude vibrations y(x, t) of a simple beam is given by
24
24
0
yy
A EI
tx
+=
where
= beam material density
A = cross-sectional area
I = area moment of inertia
E = Young’s modulus