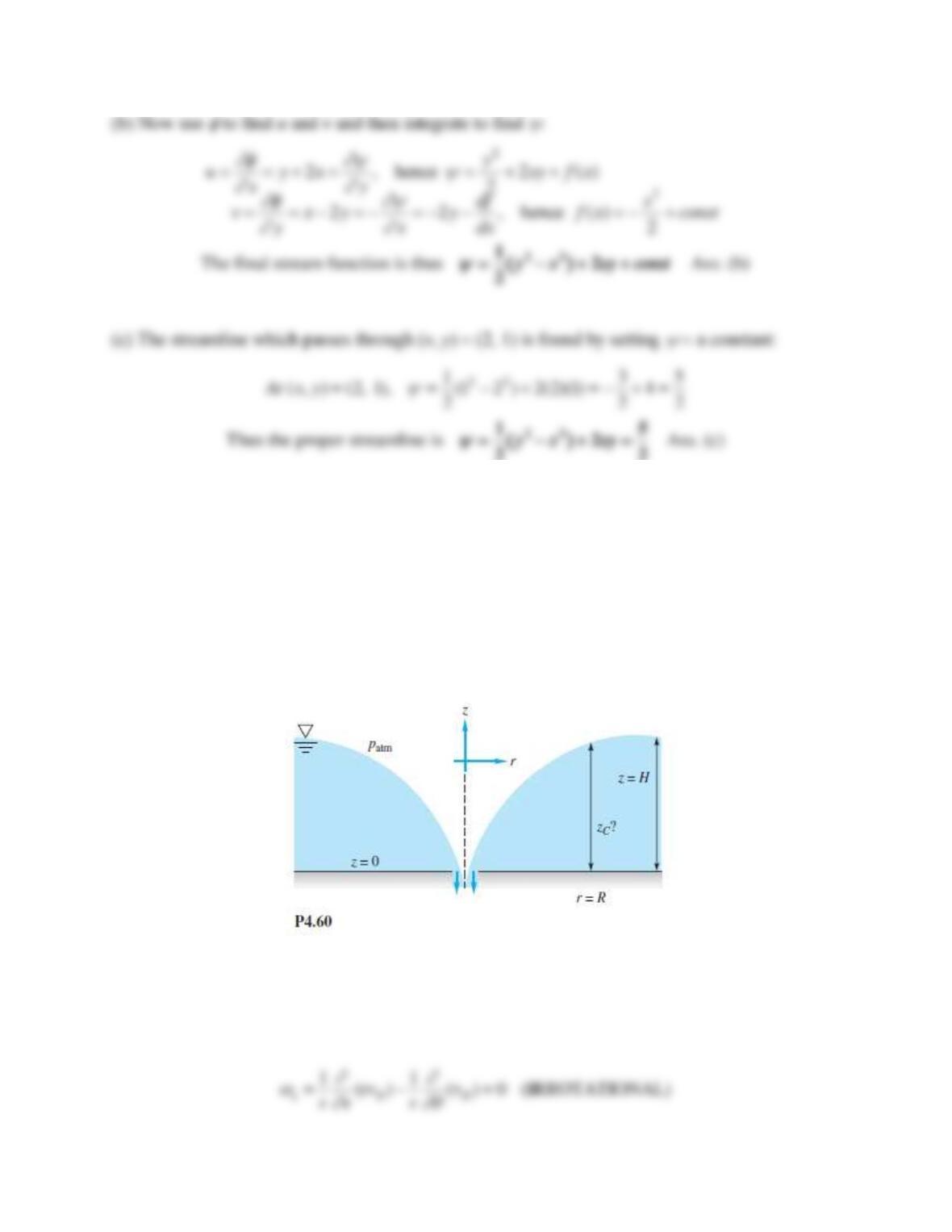
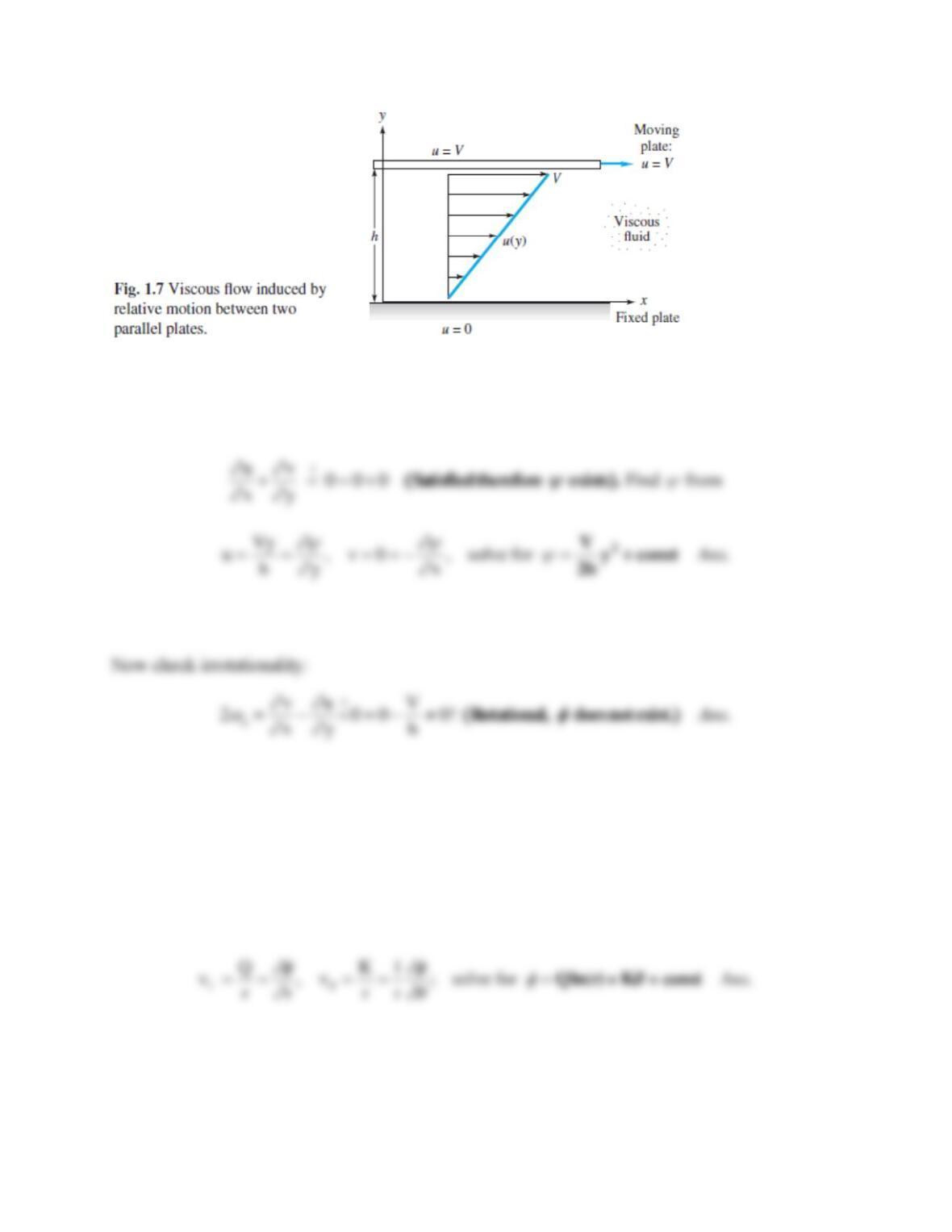
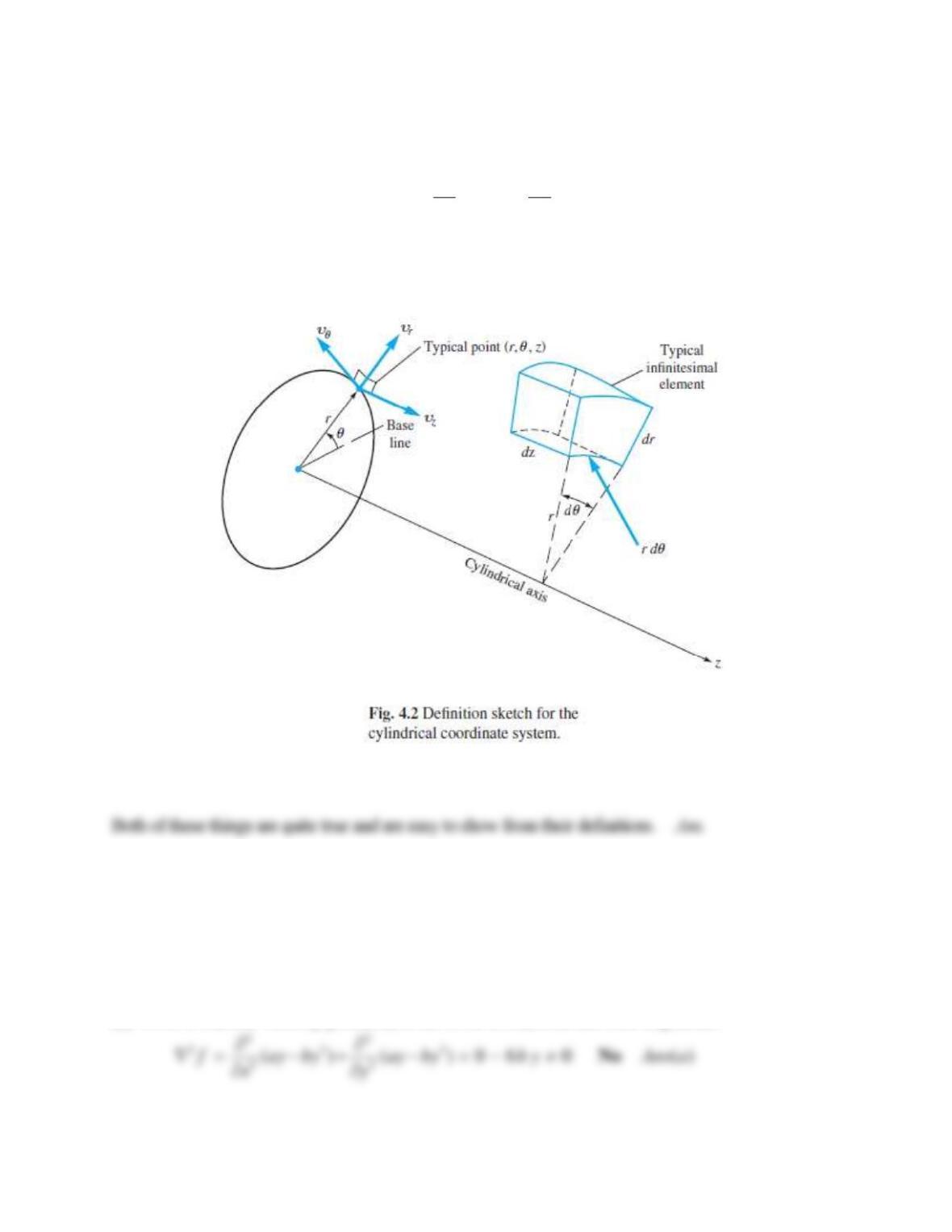
Problem 4.42
Suppose we wish to analyze the rotating, partly full cylinder of Fig. 2.23 as a spin-up problem,
starting from rest and continuing until solid-body rotation is achieved. What are the appropriate
boundary and initial conditions for this problem?
Solution 4.42
Let V = V(r, z, t). The initial condition is: V(r, z, 0) = 0. The boundary conditions are
Problem 4.43
For the draining liquid film of Fig. P4.36, what are the appropriate boundary conditions (a) at the
bottom y = 0 and (b) at the surface y = h?
Solution 4.43
The physically realistic conditions at the upper and lower surfaces are:
Problem 4.44
Suppose that we wish to analyze the sudden pipe expansion flow of Fig. P3.59, using the full
continuity and Navier- Stokes equations. What are the proper boundary conditions to handle this
problem?
Solution 4.44
First, at all walls, one would impose the no-slip condition: ur = uz = 0 at all solid surfaces: at
Problem 4.45
For the sluice gate problem of Example 3.10, list all the boundary conditions needed to solve this
flow exactly by, say, computational fluid dynamics.
Solution 4.45
There are four different kinds of boundary conditions needed, as labeled.
Problem 4.46
Fluid from a large reservoir at temperature T0 flows into a circular pipe of radius R . The pipe
walls are wound with an electric resistance coil that delivers heat to the fluid at a rate qw (energy
per unit wall area). If we wish to analyze this problem by using the full continuity, Navier-
Stokes, and energy equations, what are the proper boundary conditions for the analysis?
Solution 4.46
Letting z = 0 be the pipe entrance, we can state inlet conditions: typically uz(r, 0) = U (a uniform
Problem 4.47
A two-dimensional incompressible flow is given by the velocity field V = 3yi + 2xj in arbitrary
units. Does this flow satisfy continuity? If so, fi nd the stream function ψ(x , y) and plot a few
streamlines, with arrows.
Solution 4.47
With u = 3y and v = 2x, we may check
u/
x +
v/
y = 0 + 0 = 0, OK. Find the streamlines
Problem 4.48
Consider the following two-dimensional incompressible flow, which clearly satisfies continuity:
u = U0 = constant, υ 5 V0 = constant
Find the stream function ψ (r , θ) of this flow using polar coordinates.
Solution 4.48
In cartesian coordinates the stream function is quite easy:
u =
/
y = Uo and v = –
/
x = Vo or:
= Uoy – Vox + constant
Problem 4.49
Investigate the stream function
= K(x2 – y2), K = constant. Plot the streamlines in the full xy
plane, find any stagnation points, and interpret what the flow could represent.
Solution 4.49
The velocities are given by
Problem 4.50
In 1851, George Stokes (of Navier-Stokes fame) solved the problem of steady incompressible
low-Reynolds-number flow past a sphere, using spherical polar coordinates (r,
) [Ref. 5,
page 168]. In these coordinates, the equation of continuity is
2
( sin ) ( sin ) 0
r
r v r v
r
+=
(a) Does a stream function exist for these coordinates? (b) If so, find its form.
Solution 4.50
Two velocity components and two continuity terms. Yes,
exists! Ans.(a)
Problem 4.51
The velocity profile for incompressible pressure-driven laminar flow between parallel plates (see
Fig. 4.12b) has the form u = C(h2 – y2), where C is a constant. (a) Determine if a stream function
exists. (b) If so, determine a formula for the stream function.
Solution 4.51
(a) A stream function exists, for a single velocity component u, if u/x = 0, which it certainly
Problem 4.52
A two-dimensional, incompressible, frictionless fluid is guided by wedge-shaped walls into a
small slot at the origin, as in Fig. P4.52. The width into the paper is b, and the volume flow rate is
Q. At any given distance r from the slot, the flow is radial inward, with constant velocity. Find
an expression for the polar-coordinate stream function of this flow.
Solution 4.52
We can find velocity from continuity:
Problem 4.53
For the fully developed laminar-pipe-flow solution of Eq. (4.137), find the axisymmetric stream
function
(r, z). Use this result to determine the average velocity V = Q/A in the pipe as a ratio of
umax.
Solution 4.53
The given velocity distribution, vz = umax(1 – r2/R2), vr = 0, satisfies continuity, so a stream
function does exist and is found as follows:
1-2 2 1 0-R max max max
Problem 4.54
An incompressible stream function is defined by
23
2
( , ) (3 )
U
x y x y y
L
=−
where U and L are (positive) constants. Where in this chapter are the streamlines of this flow
plotted? Use this stream function to find the volume flow Q passing through the
rectangular surface whose corners are defined by (x, y, z) = (2L, 0, 0), (2L, 0, b), (0, L, b), and
(0, L, 0). Show the direction of Q.
Solution 4.54
This flow, with velocities u =
/
y = 3U/L2(x2 – y2), and
Problem 4.55
The proposed flow in Prob. P4.19 does indeed satisfy the incompressible equation of
continuity. Determine the polar-coordinate stream function of this flow.
Solution 4.55
The plane polar-coordinate stream function is defined by Eq. (4.101):
Problem 4.56
Investigate the velocity potential
= Kxy, K = constant. Sketch the potential lines in the full xy
plane, find any stagnation points, and sketch in by eye the orthogonal streamlines. What could
the flow represent?
Solution 4.56
The potential lines,
= constant, are hyperbolas, as shown. The streamlines, sketched in as
Problem 4.57
A two-dimensional incompressible flow field is defined by the velocity components
22
x y y
u V v V
L L L
= − = −
where V and L are constants. If they exist, find the stream function and velocity potential.
Solution 4.57
First check continuity and irrotationality:
Problem 4.58
Show that the incompressible velocity potential in plane polar coordinates
(r,
) is such that
1
rrr
==
Finally show that
as defined here satisfies Laplace’s equation in polar coordinates for
incompressible flow.
Solution 4.58
Both of these things are quite true and easy to show from the definition of the gradient vector in
Problem 4.59
Consider the two-dimensional incompressible velocity potential
= xy + x2 – y2. (a) Is it true
that 2
= 0, and, if so, what does this mean? (b) If it exists, find the stream function
(x, y) of
this flow. (c) Find the equation of the streamline which passes through (x, y) = (2, 1).
Solution 4.59
(a) First check that 2
= 0, which means that incompressible continuity is satisfied.
Problem 4.60
Liquid drains from a small hole in a tank, as shown in Fig. P4.60, such that the velocity field set
up is given by
r 0,
z 0,
= KR2/r, where z = H is the depth of the water far from the hole. Is
this flow pattern rotational or irrotational? Find the depth zc of the water at the radius r = R.
Solution 4.60
From Appendix D, the angular velocity is
Problem 4.61
An incompressible stream function is given by
sin .a b r
=+
(a) Does this flow have a
velocity potential? (b) If so, find it.
Solution 4.61
(a) Find the (polar coordinate) velocities and see if the angular velocity is zero:
Problem 4.62
Show that the linear Couette flow between plates in Fig. 1.7 has a stream function but no
velocity potential. Why is this so?
Solution 4.62
Given u = Vy/h, v = 0, check continuity:
Problem 4.63
Find the two-dimensional velocity potential
(r,
) for the polar-coordinate flow pattern
r = Q/r,
= K/r, where Q and K are constants.
Solution 4.63
Relate these velocity components to the polar-coordinate definition of
:
Problem 4.64
Show that the velocity potential
(r, z) in axisymmetric cylindrical coordinates (see Fig. 4.2 of
the text) is defined by the such that:
rz
rz
==
Further show that for incompressible flow this potential satisfies Laplace’s equation in (r, z)
coordinates.
Solution 4.64
Problem 4.65
Consider the function f = ay – by3. (a) Could this represent a realistic incompressible
velocity potential? Extra credit: (b) Could it represent a stream function?
Solution 4.65
(a) To be a realistic velocity potential, it has to be irrotational and thus Laplacian:
Problem 4.66
A plane polar-coordinate velocity potential is defined by
cos const
KK
r
==
Find the stream function for this flow, sketch some streamlines and potential lines, and interpret
the flow pattern.
Solution 4.66
Evaluate the velocities and thence find the stream function:
Problem 4.67
A stream function for a plane, irrotational, polar-coordinate flow is
ln and constC K r C K
= − =
Find the velocity potential for this flow. Sketch some streamlines and potential lines, and
interpret the flow pattern.
Solution 4.67
If this problem is given early enough (before Section 4.10 of the text), the students will discover
Problem 4.68
For the velocity distribution of Prob. P4.4, (a) determine if a velocity potential exists and, if it
does, (b) find an expression for
(x,y) and sketch the potential line which passes through the
point (x, y) = (L/2, L/2).
Solution 4.68
Recall the given flow, u = Uo(1+x/L) and v =
−
Uo(y/L). (a) Calculate if the flow is irrotational.
Problem 4.69
A steady, two-dimensional flow has the following polar-coordinate velocity potential:
where C and K are constants. Determine the stream function
(r,
) for this flow. For extra
credit, let C be a velocity scale U, let K = UL, and sketch what the flow might represent.
rKrC lncos +=
Solution 4.69
Write out the
and
expressions for polar-coordinate velocities:
Problem 4.70
A CFD model of steady two-dimensional incompressible flow has printed out the values of
stream function
(x, y), in m2/s, at each of the four corners of a small 10cm-by-10cm cell, as
shown in Fig. P4.70. Use these numbers to estimate the resultant velocity in the center of the
cell and its angle
with respect to the x axis.
1
cos , sin ( )
r
K
v C C r K f r
r r r
= = + = = + +
hence
Solution 4.70
Quick analysis: the
values are higher on the top than the bottom, therefore u is to the right.
The
values are higher on the right than the left, therefore v is down. There are several ways to