Problem 7.66 In Example 7.9, determine the ycoor-
dinate of the centroid of the line.
x
y
y ⫽ x2
(1, 1)
L
(1, 1)
dy
x
y
dL
Solution: The expression derived in Example 7.9 for the element
dL of the line in terms of xis
dL D1C4x2dx
The ycoordinate of the centroid is
yDL
ydL
L
0
x21C4x2dx
1
Problem 7.67 Determine the coordinates of the cen-
troid of the line.
y
y ⫽x2
Solution:
xds
x1Cdy
dx
x1C⊲2x⊳2dx
Problem 7.68 Determine the xcoordinate of the
centroid of the line.
y
Solution: The length: Noting that dy
dx D⊲x 1⊳1/2, the element
of length is
Problem 7.69 Determine the xcoordinate of the
centroid of the line.
y
Solution: The length: Noting that dy
dx Dx1/2the element of
length is
Problem 7.70 Use the method described in Exam-
ple 7.10 to determine the centroid of the circular arc.
y
a
Solution: The length of the differential line element of the circular
arc is dL DRd. The coordinates of the centroid are
xdL
⊲R cos ⊳ Rd
552
Problem 7.71 In Active Example 7.11, suppose that
the cylinder is hollow with inner radius R/2 as shown.
If the dimensions RD6 in, hD12 in, and bD10 in,
what is the xcoordinate of the centroid of the volume?
y
z
hR
Solution: Let the cone be volume 1, let the solid cylinder be
the xcoordinates of the their centroids are
Problem 7.72 Use the procedure described in Exam-
ple 7.12 to determine the xcomponent of the centroid
of the volume.
25 mm
yy
Solution: Let the rectangular part without the cutout be volume 1,
Problem 7.73 Determine the centroids of the volumes. y
z
R
Solution: The object will be divided into a cone and a hemisphere.
Using tables we have in the xdirection
3
4⊲4R⊳ 1
3R2[4R]C4RC3R
82R3
3
Problem 7.74 Determine the centroids of the volumes. y
200 mm
Solution: We have a hemisphere and a hemispherical hole. From
symmetry yDzD0
Problem 7.75 Determine the centroids of the volumes.
y
z
Solution: This is a composite shape. Let us consider a solid
cylinder and then subtract the cone. Use information from the appendix
Volume Volume (mm3)x x (mm)
554
Problem 7.76 Determine the centroids of the volumes.
y
x
120 mm 25 mm
75 mm
20 mm
25 mm
3R2D/20
3D/2
4r2D0⊲H Ch⊳ D/2
where RDW/2. For the composite,
(L)(W)
120 mm
100 mm
z
y
1
7.76 (Continued)
Substituting into the formulas for the composite, we get
(D)
z
x
y
2
4
r = 20 mm
Problem 7.77 Determine the centroids of the volumes.
y
z
5 in
1 in1.75 in
4 in
556
Problem 7.78 Determine the centroids of the volumes.
x
z
y
180
mm 180
mm
30 mm
60 mm
3r2L
23(L/2)/4
(mm3) (mm)
Cylinder 5.089 ð10590
Cone 1 1.357 ð106270
Cone 2 1.696 ð105135
LD360 mm
y
Cylinder 60 mm
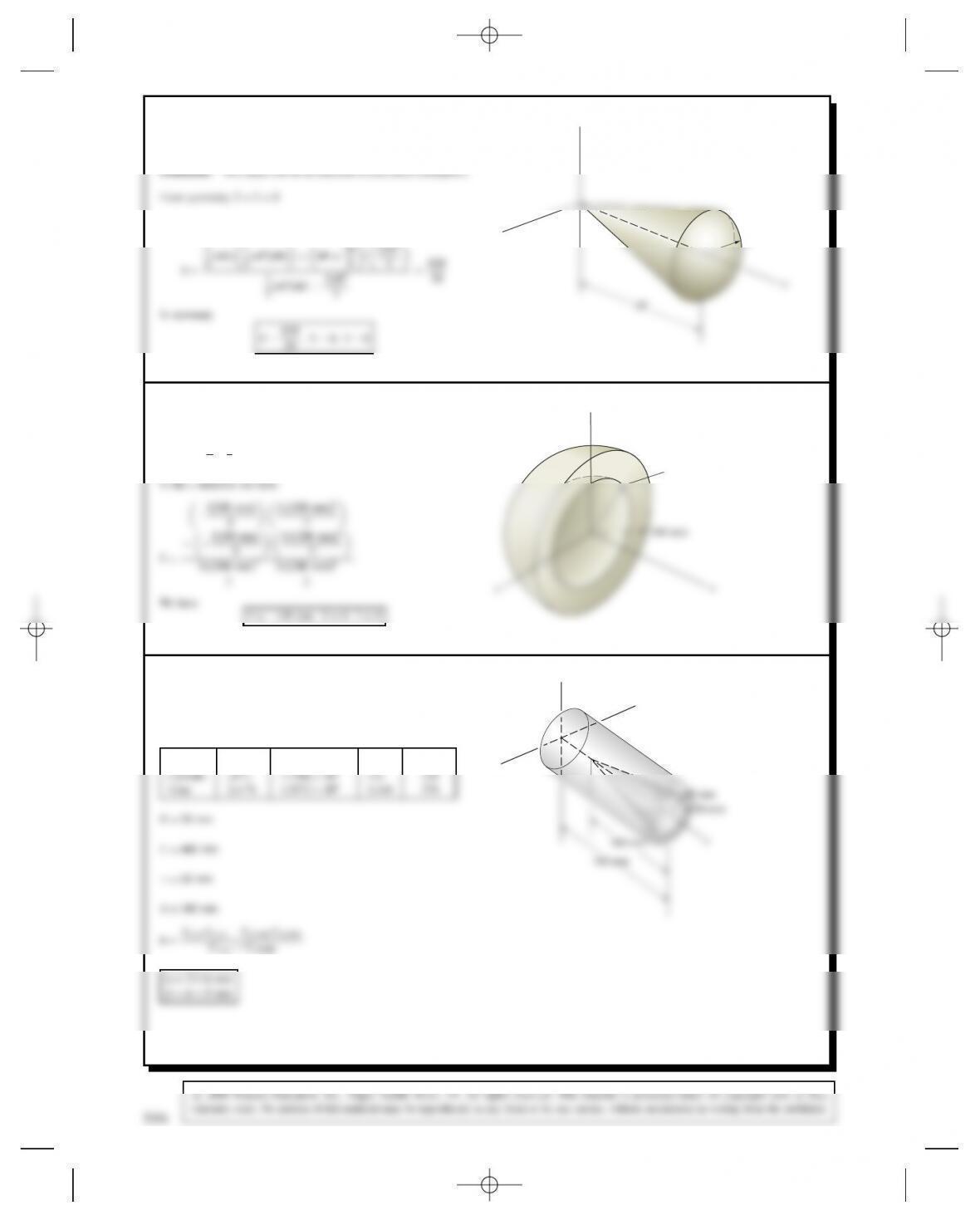
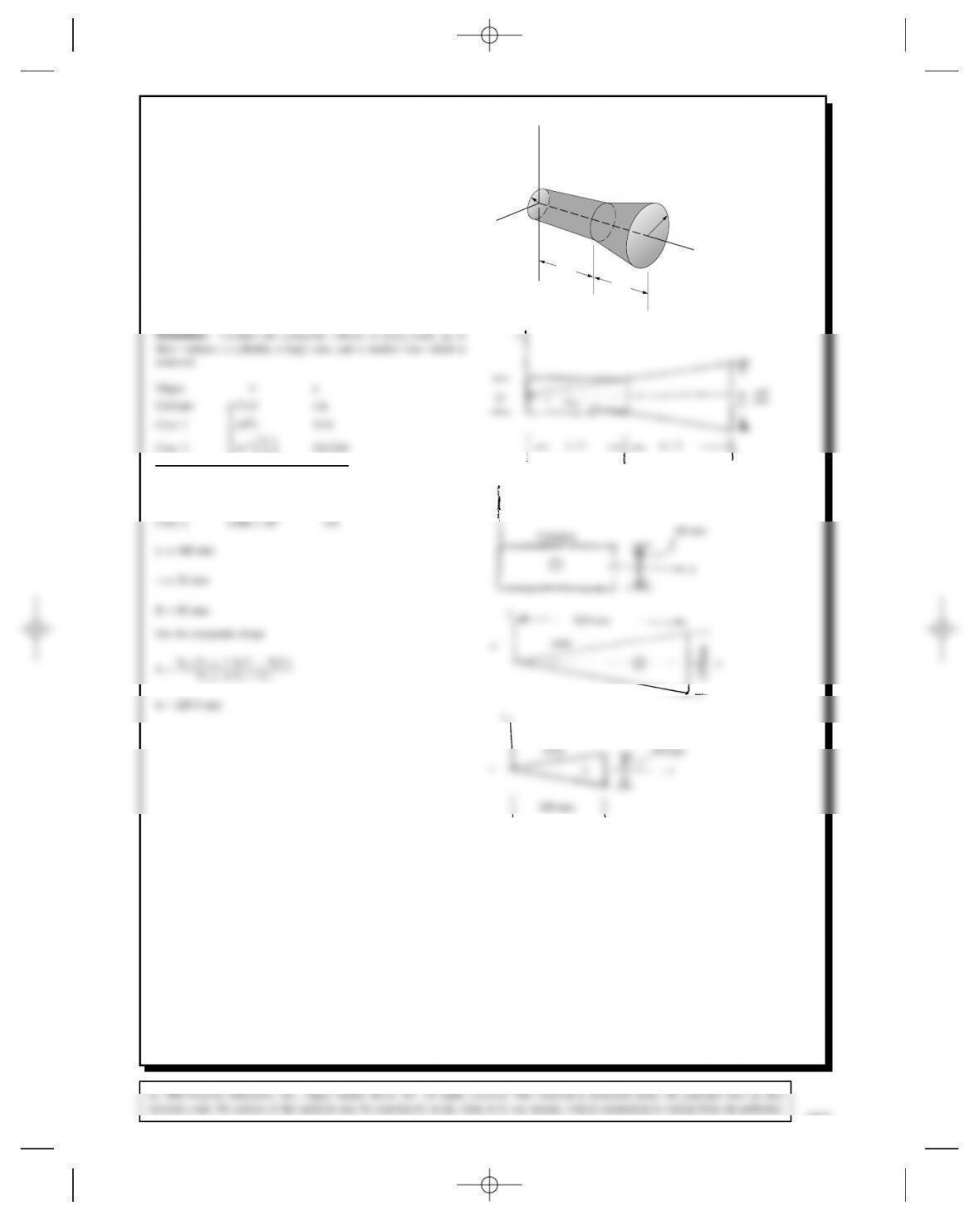
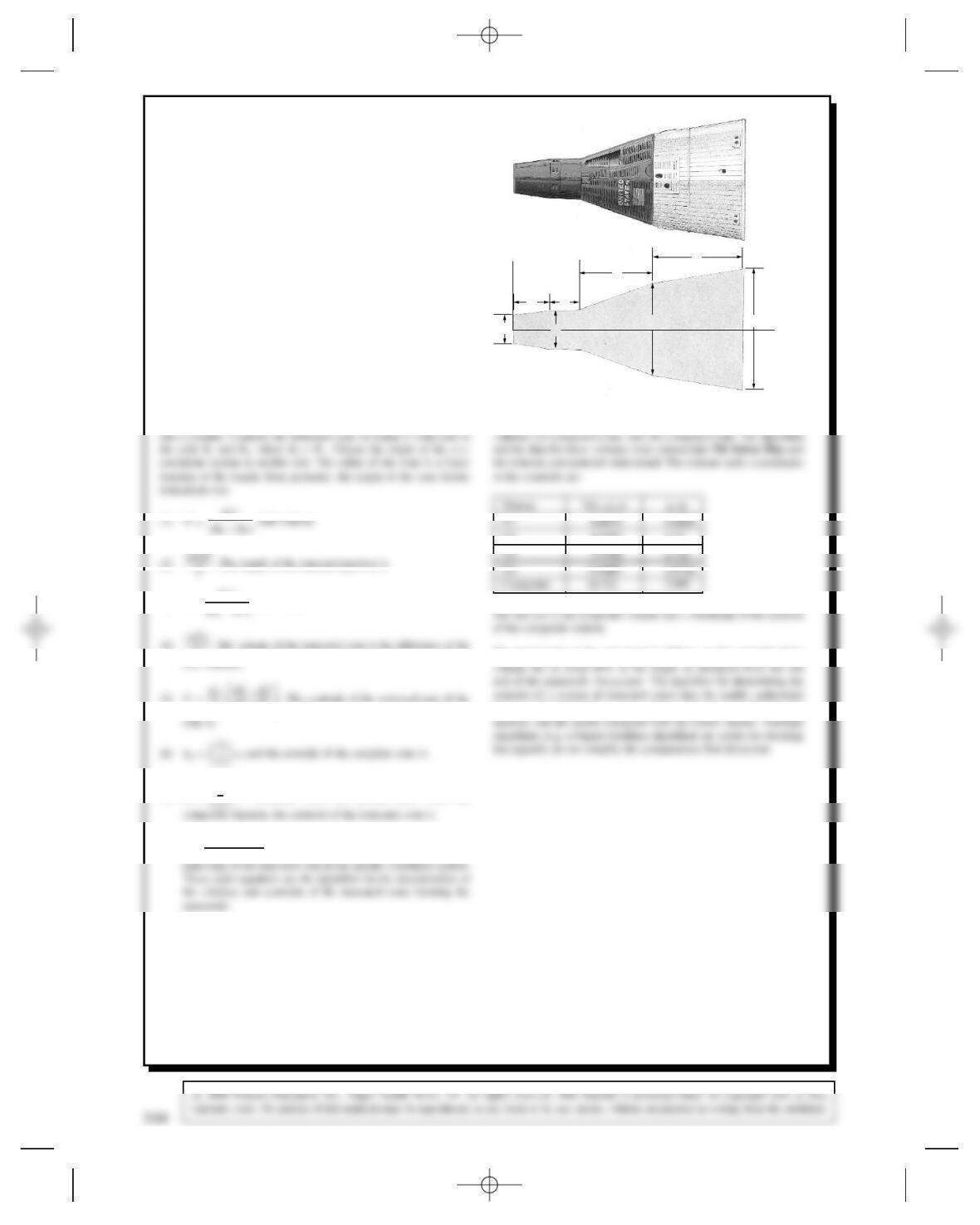
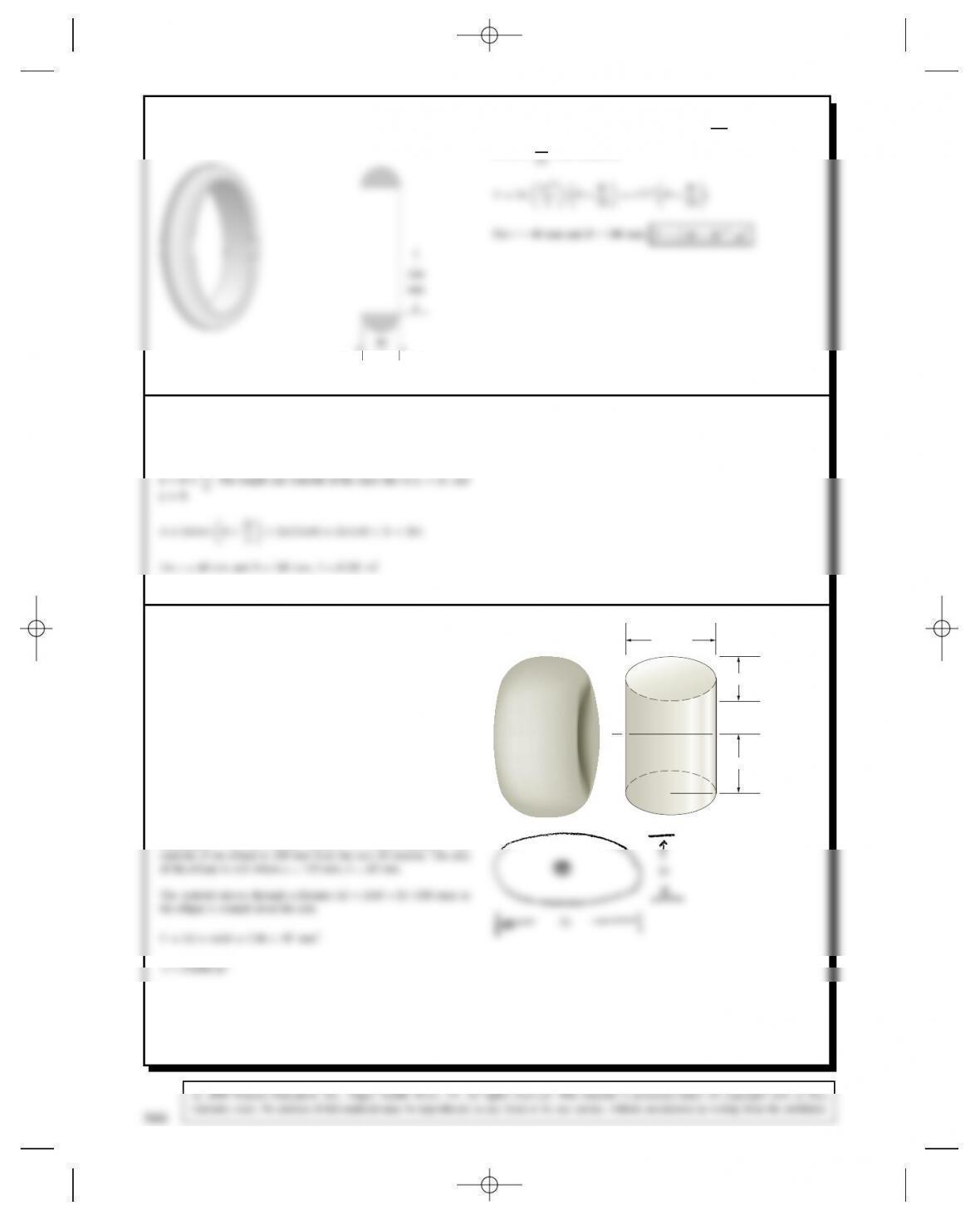
Problem 7.79 The dimensions of the Gemini space-
craft (in meters) are aD0.70, bD0.88, cD0.74,
dD0.98, eD1.82, fD2.20, gD2.24, and hD2.98.
Determine the centroid of its volume.
y
x
d
a
b c
e
g
fh
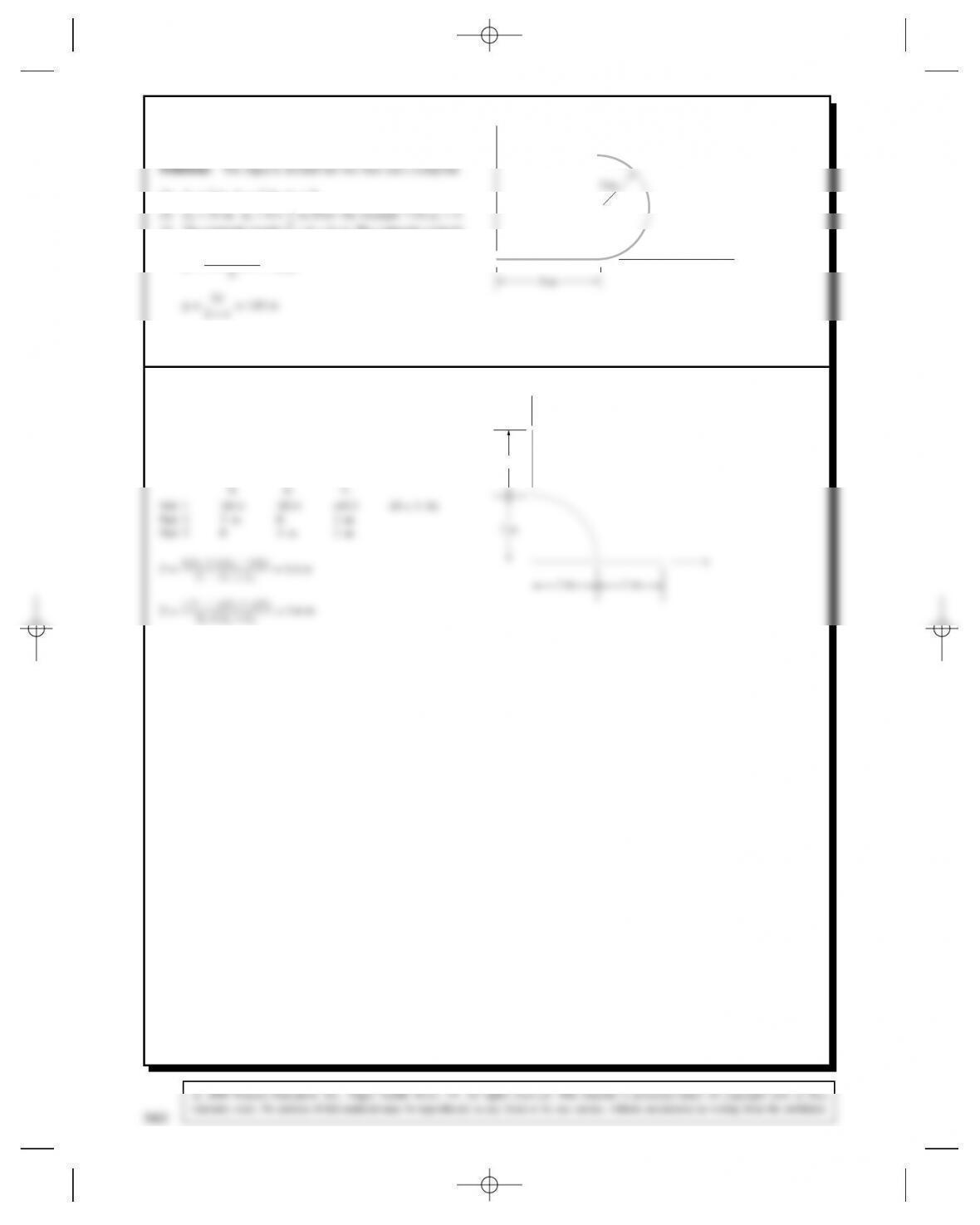
Solution: The spacecraft volume consists of three truncated cones
(4) R2
3. The volume of the truncated cone is the difference of the
two volumes,
(5) VDL
3R3
R2R1. The centroid of the removed part of the
Beginning from the left, the volumes are (1) a truncated cone, (2) a
V2 0.5582 1.25
The total length of the spacecraft is 5.68 m, so the centroid of the
if it is implemented for a cone of known dimensions divided into
558
Problem 7.80 Two views of a machine element are
shown. Determine the centroid of its volume.
y
24 mm
8 mm
50 mm
y
Solution: We divide the volume into six parts as shown. Parts 3
and 6 are the “holes”, which each have a radius of 8 mm. The volumes
V5D1
2⊲18⊳2⊲20⊳D10,179 mm3,
V6D⊲8⊳2⊲20⊳D4021 mm3.
The coordinates of the centroids are
z2D0,
x3D25 mm,
y5D18 mm,
y
2
z5D24 C16 C4⊲18⊳
3D47.6mm,
x6D10 mm,
36.63 mm and zD3.52 mm
Problem 7.81 In Example 7.13, suppose that the
circular arc is replaced by a straight line as shown.
Determine the centroid of the three-segment line.
y
(0, 0, 2) m
(0, 2, 0) m
Problem 7.82 Determine the centroids of the lines.
y
x
Solution: The object is divided into two lines and a composite.
(1) L1D6m,x1D3m,y1D0.
(3) The composite length: LD6C3m. The composite centroid:
xDL1x1CL2x2
Problem 7.83 Determine the centroids of the lines. y
2 m
Solution: Break the composite line into three parts (the quarter
circle and two straight line segments) (see Appendix B).
560
Problem 7.84 The semicircular part of the line lies in
the x–zplane. Determine the centroid of the line.
100 mm
120 mm
160 mm
x
y
z
L3 188.7 80 50 0
Composite 665.7 65.9 21.7 68.0
Problem 7.85 Determine the centroid of the line. y
200 mm
Solution: Break into a straight line and an arc.
1
2⊲200 mm tan 60°⊳2cos 30°C2/3
Problem 7.86 Use the method described in Active
Example 7.14 to determine the area of the curved part
of the surface of the truncated cone.
x
z
y
R
h
Problem 7.87 Use the second Pappus–Guldinus theo-
rem to determine the volume of the truncated cone.
Solution: Work with the trapezoidal area
AD⊲R/2⊳⊲h/2⊳C⊲1/2⊳⊲R/2⊳⊲h/2⊳D3Rh
VD2yA D27R
18 3Rh
8D7R2h
24
VD7R2h
24
R
Problem 7.88 The area of the shaded semicircle is
1
2R2. The volume of a sphere is 4
3R3. Extend the
approach described in Example 7.15 to the second
y
yS
562
Problem 7.89 Use the second Pappus–Guldinus
theorem to determine the volume generated by revolving
the curve about the yaxis.
Solution: The x coordinate of the centroid:. The element of area
is the vertical strip of height ⊲1y⊳ and width dx. Thus
AD1
⊲1y⊳ dx D1
⊲1x2⊳dx.
Problem 7.90 The length of the curve is LD1.479,
and the area generated by rotating it about the xaxis
Problem 7.91 Use the first Pappus–Guldinus theorem
LD1.479. The elementary length of the curve is
dL D1Cdy
dx 2
dx.
Noting dy
Problem 7.92 A nozzle for a large rocket engine is
designed by revolving the function yD2
3⊲x 1⊳3/2about
the yaxis. Use the first Pappus–Guldinus theorem to
determine the surface area of the nozzle.
y
Problem 7.93 The coordinates of the centroid of the
line are xD332 mm and yD118 mm. Use the first
Pappus-Guldinus theorem to determine the area of the
surface of revolution obtained by revolving the line about
the xaxis.
y
x
200 mm
60⬚
564
Problem 7.94 The coordinates of the centroid of the
area between the xaxis and the line in Problem 7.93 are
xD355 mm and yD78.4 mm. Use the second Pappus-
Guldinus theorem to determine the volume obtained by
revolving the area about the xaxis.
Problem 7.95 The volume of revolution contains a
hole of radius R.
(a) Use integration to determine its volume.
(b) Use the second Pappus–Guldinus theorem to deter-
R
R + a
Problem 7.96 Determine the volume of the volume of
revolution.
mm
Solution: The area of the semicircle is ADr2
2. The centroid is
yDRC4r
Problem 7.97 Determine the surface area of the
volume of revolution in Problem 7.96.
Solution: The length and centroid of the semicircle is LoDr,
Problem 7.98 The volume of revolution has an
elliptical cross section. Determine its volume.
180 mm
130 mm
230 mm
Solution: Use the second theorem of Pappus-Guldinus. The
566
Problem 7.99 Suppose that the bar in Active
Example 7.16 is replace with this 100-kg homogeneous
bar. (a) What is the xcoordinate of the bar’s center of
mass? (b) Determine the reactions at Aand B.
1 m
0.5 m
1 m
A
B
x
y
Solution:
(b) The equilibrium equations are
Problem 7.100 The mass of the homogeneous flat
plate is 50 kg. Determine the reactions at the supports A
and B.
AB
200 mm
100 mm
400 mm
Rectangle 3.2ð105400
Circle 3.14 ð104600
Triangle 1.2ð1051000
Composite 4.09 ð105561
568
Problem 7.101 The suspended sign is a homogeneous
flat plate that has a mass of 130 kg. Determine the axial
forces in members AD and CE. (Notice that the yaxis
is positive downward.) 1 m
2 m 4 m
CA
DB
x
E
5.3333 D2.25 ft.
The equilibrium conditions: The angle of the member CE is
Problem 7.102 The bar has a mass of 80 kg. What are
the reactions at Aand B?
2 m
2 m
A
B
Solution: Break the bar into two parts and find the masses and
centers of masses of the two parts. The length of the bar is
X1
y
m1g
570