Unlock document.
This document is partially blurred.
Unlock all pages and 1 million more documents.
Get Access
*12–152.
A particle Ptravels along an elliptical spiral path such
that
its position vector ris defined by
where tis in
seconds
and the arguments for the sine and cosine are given
in
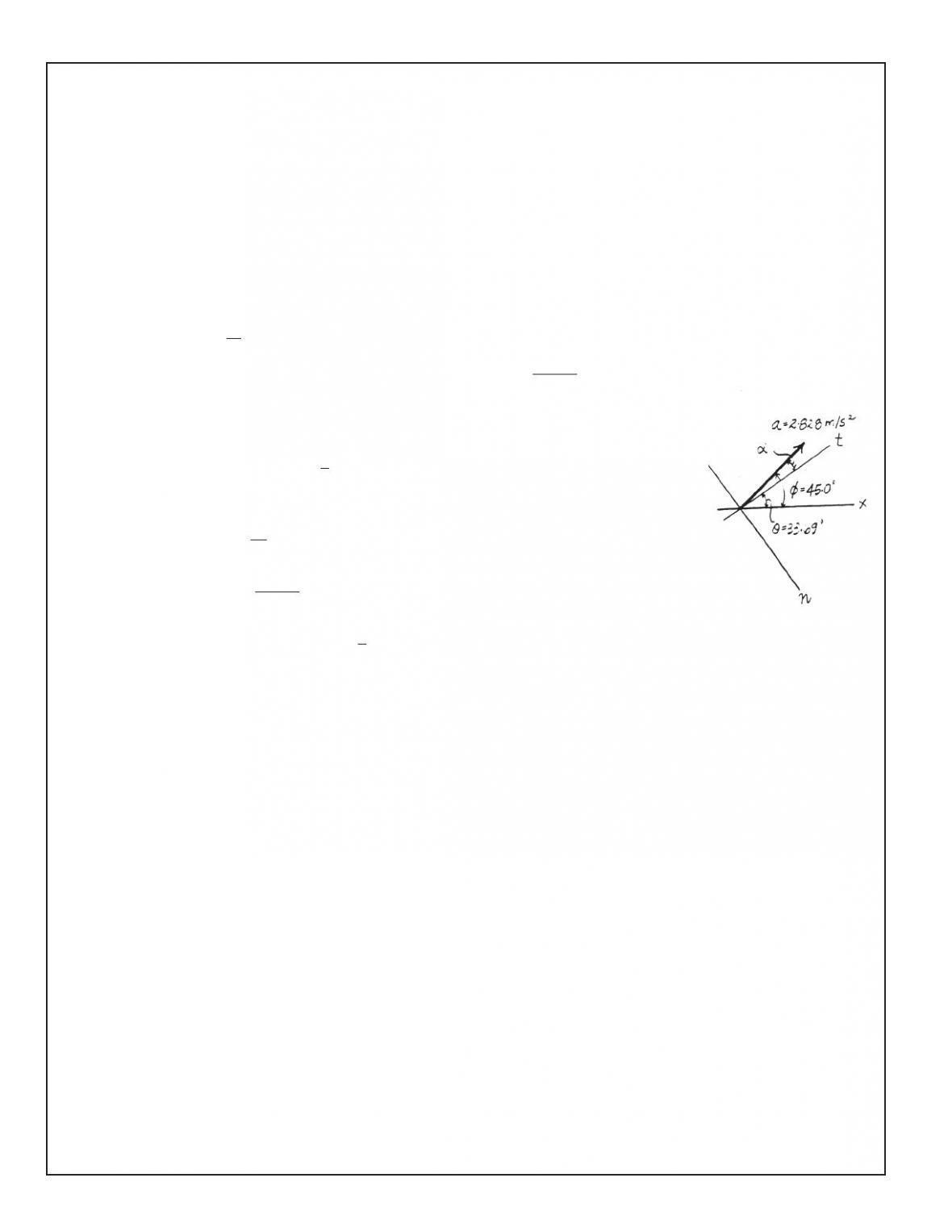
radians.When determine the coordinate
direction
angles and which the binormal axis to the
osculating plane makes with the
x, y, and zaxes. Hint: Solve
for
the velocity and acceleration of the particle in
terms of their
i,j,kcomponents.The binormal is parallel to
Why?vP*aP.
aP
vP
g,b,a,
t=8s,
r=52 cos10.1t2i+1.5 sin10.1t2j+12t2k6m,
SOLUTION
#=-0.2 sin (0.1t)i+0.15 cos (0.1t)j+2k
rP=2 cos (0.1t)i+1.5 sin (0.1t)j+2tk
z
y
r
P
12–153.
The motion of a particle is defined by the equations
and , where t is in seconds.
Determine the normal and tangential components of the
particle’s velocity and acceleration when .t=2s
y=(t2)mx=(2t+t2)m
12–154.
If the speed of the crate at A is 15 ft s, which is increasing at
a rate determine the magnitude of the
acceleration of the crate at this instant.
v
#=3 ft>s2,
>
SOLUTION
Thus,
Acceleration:
The magnitude of the crate’s acceleration at Ais
Ans.a
=2
a
t
2
+
a
n
2
=2
32
+
6.8562
=
7.48 ft
>
s2
an=v2
r=152
32.82 =6.856 ft>s2
at=v
#=3ft>s2
r=
B
1+ady
dx b2
R
3>2
`
d2y
dx2
`
=
B
1+
¢
1
8x
≤
2
R
3>2
`
1
8
`
4x=10 ft
=32.82 ft
d2y
dx2=1
8
dy
dx =1
8 x
y
x
A
y fi x2
1
16
12–155.
SOLUTION
Ans.a=a2
r+a2
u=(-11.6135)2+(-8.3776)2=14.3 in. s2
au=ru
$
+2r
#u
#
=4(-2.0944) +0=-8.3776 in.>s2
ar=r
$-ru
#2=0-4(-1.7039)2=-11.6135 in.>s2
r=4r
#=0r
$=0
u
$
=d2u
dt2=-4 cos 2t2t=0.5099 s
=-2.0944 rad>s2
u
#
=du
dt =-2 sin 2t2t=0.5099 s
=-1.7039 rad>s
p
A particle is moving along a circular path having a radius
of4in. such that its position as a function of time is given
by where is in radians and tis in seconds.
Determine the magnitude of the acceleration of the particle
when u=30°.
uu =cos 2t,
*12–156.
For a short time a rocket travels up and to the right at a
constant speed of 800 m
>
s along the parabolic path
y
=
600
-
35x2. Determine the radial and transverse
components of velocity of the rocket at the instant
u
= 60°,
where
u
is measured counterclockwise from the x axis.
12–157.
A particle moves along a path defined by polar coordinates
r = (2et) ft and
u
= (8t2) rad, where t is in seconds. Determine
the components of its velocity and acceleration when t = 1 s.
12–158.
SOLUTION
Ans
.
Ans
.
a=2a2
Pl +a2
Pr =2(0.001 22)2+(43 200)2=43.2(103)ft>s2
aPr =
v2
Pr
r=(360)2
3=43 200 ft>s2
v=2v2
Pl +v2
Pr =2(293.3)2+(360)2=464 ft>s
vPr =120(3) =360 ft>s
aPl =
¢
3mi
h2
≤¢
5280 ft
1mi
≤¢
1h
3600 s
≤
2
=0.001 22 ft>s2
vPl =
¢
200 mi
h
≤¢
5280 ft
1mi
≤¢
1h
3600 s
≤
=293.3 ft>s
An airplane is flying in a straight line with a velocity of
200
and an acceleration of .If the propeller
has
a diameter of 6 ft and is rotating at an angular rate of
120
,determine the magnitudes of velocity and
acceleration
of a particle located on the tip of the
propeller
.
rad>s
3mi>h2
mi>h
12–159.
The small washer is sliding down the cord OA. When it is at
the midpoint, its speed is 28 m
>
s and its acceleration is
7 m
>
s2. Express the velocity and acceleration of the washer
at this point in terms of its cylindrical components.
7b
6 m
O
z
y
A
*12–160.
A radar gun at rotates with the angular velocity of
and angular acceleration of ,
at the instant ,as it follows the motion of the car
traveling along the circular road having a radius of
. Determine the magnitudes of velocity and
acceleration of the car at this instant.
r=200 m
u=45°
u
$
=0.025 rad>s2
u
#
=0.1 rad>s
O
SOLUTION
Velocity:
Thus, the magnitude of the car’s velocity is
Ans.
Acceleration:
Thus, the magnitude of the car’s acceleration is
Ans.a
=2
a
r
2
+
a
u
2
=2
(
-
2)2
+
52
=
5.39 m
>
s2
au=r
$
u+2r
#u
#
=200(0.025) +0=5 m>s2
ar=r
#-ru2
#
=0-200(0.12)=-2 m>s2
v=2vr2+vu2=202+202=20 m>s
vu=ru
#
=200(0.1) =20 m>s
vr=r
#=0
r
r fi 200 m
O
u
170
12–161.
SOLUTION
Ans.
Ans.
Ans.
Ans.au=ru
$
+2r
#u
#
=2 cos t102+21-2 sin t2
a
1
2b
=-2 sin t
ar=r
#
=-2 cos t-(2cos ) ta1
2b2
=-
2cos t
vu=ru
#
=(2 cos ) ta1
2b=cos t
vr=r
#=-2 sin t
u=t
2u
#
=1
2u
$
=0
r=2 cos tr
#=-2 sin tr
$=-2 cos t
If a particle moves along a path such that
and where tis in seconds, plot the path
and determine the particle’s radial and transverse
components of velocity and acceleration.
r=f1u2
u=1t>22rad,
r=
1
2 cos t
2
ft
Ans:
vr=-2
sin
t
vu=
cos
t
ar=-
5
2
cos
t
au=-2
sin
t
171
12–162.
If a particle moves along a path such that r = (eat) m and
u
= t,
where t is in seconds, plot the path r = f(
u
), and determine the
particle’s radial and transverse components of velocity and
acceleration.
SOLUTION
r=eat
r
#
=aeat
r
$
=a2eet
u=t
u
#
=1
u
$
=0
vr=
#
r=aeat
Ans.
v
a=
r
#
u
=
e
at
(1)
=
e
at
Ans.
ar=
$
r-r
#
u
2=a2eat -eat(1)2=eat(a2-1)
Ans.
a
u=
r
$
u
+
2
#
r
#
u
=
e
at
(0)
+
2(ae
at
)(1)
=
2ae
at
Ans.
Ans:
vr=aeat
vu=eat
ar
=
e
at
(
a
2-
1
)
au=2aeat
12–163.
The car travels along the circular curve having a radius
.At the instant shown, its angular rate of rotation
is , which is decreasing at the rate
. Determine the radial and transverse
components of the car’s velocity and acceleration at this
instant and sketch these components on the curve.
u
$
=-0.008 rad>s2
u
#
=0.025 rad>s
r=400 ft
SOLUTION
Ans.
Ans.
Ans.
ar=r-ru
#2=0-400(0.025)2=-0.25 ft>s2
vu=ru
#
=400(0.025) =10 ft>s
vr=r
#=0
u
#
=0.025 u=-0.008
r=400
r
#=0r
$=0
r400 ft
u
.
$
*12–164.
SOLUTION
r=400 ft
r
#=0r
$=0
The car travels along the circular curve of radius
with
a constant speed of . Determine the angular
rate
of rotation of the radial line rand the magnitude of
the car’
s acceleration.
u
#v=30 ft>s
r
=400 ft
r400 ft
u
.
12–165.
SOLUTION
But,
Substituting and combining terms yields
Ans.a
#=ar
### -3ru
#
2 -3ru
#
u
$bur +a3r
#u
$
+ ru
$#
+3r
$u
#
-ru
#3buu+az
###buz
ur=u
#
uu
u
#
u=-u
#
ur
u
#
z=0
a
$=ar
### -r
#u
#2-2ru
#
u
$bur +ar
$-ru
#2bu
#
r+ar
#u
$
+ ru
$#
+2r
$u
#
+2r
#u
$buu+aru
$
+2r
#u
#bu
#
u+z
### uz+z
$u
#
z
a=ar
$-ru
#
2bur+aru
$
+ 2r
#u
#buu+z
$uz
The time rate of change of acceleration is referred to as the
jerk, which is often used as a means of measuring passenger
discomfort. Calculate this vector, in terms of its
cylindrical components,using Eq. 12–32.
a
#,
12–166.
Thus,
Ans.a=2(-39.196)2+(-28.274)2=48.3 in.>s2
au=ru
$
+2r
#u
#
=6(-4.7124) +0=- 28.274
ar=r
$-ru
#2=0-6(2.5559)2=-39.196
u
$
=-4.7124 rad>s2
u
#
=2.5559 rad>s
t=10.525 s
30°
180°p=sin 3t
At u=30°,
u
$
=-9 sin 3t
u
#
=3 cos 3t
u=sin 3t
r=6 in.,
r
r
A particle is moving along a circular path having a radius of
6 in. such that its position as a function of time is given by
where is in radians, the argument for the sine are
, and tis in seconds. Determine the acceleration of
the particle at The particle starts from rest at
u=0°.
u=30°.
uu =sin 3t,
in radians
12–167.
The slotted link is pinned at O, and as a result of the
constant angular velocity it drives the peg Pfor
a short distance along the spiral guide where
is in radians. Determine the radial and transverse
components of the velocity and acceleration of Pat the
instant u=p>3 rad.
u
r=10.4 u2m,
u
#
=3 rad>s
At
Ans.
Ans.
Ans.
Ans.au=ru
$
+2r
#u
#
=0+2(1.20)(3) =7.20 m>s2
ar=r
$-ru
#2=0-0.4189(3)2=-3.77 m>s2
vu=ru
#
=0.4189(3) =1.26 m>s
v=r
#=1.20 m>s
r
$=0.4(0) =0
r
#=0.4(3) =1.20
u=p
3,r=0.4189
r
$=0.4 u
$
r
r
P
r0.4u
0.5 m
u3 rad/s
·
SOLUTION
r=25(1 -cos
u
)=25(1 -cos 120°)=37.5 ft
r
#
=
25 sin uu
#
=
25 sin 120
°
(2)
=
43.30 ft
>
s
r
$
=
25[cos uu
#2+
sin uu
$
]
=
25[cos 120
°
(2)
2+
sin 120
°
(0.2)]
=-
45.67 ft
>
s
2
vr=r
#
=43.30 ft>s
v
u=
ru
#
=
37.5(2)
=
75 ft
>
s
v
=2
v
r
2
+
v
u
2
=2
43.302
+
752
=
86.6 ft
>
s Ans.
a
r=
r
$-
ru
#2=-
45.67
-
37.5(2)
2=-
195.67 ft
>
s
2
a
u=
ru
$
+
2ru
#
=
37.5(0.2)
+
2(43.30)(2)
=
180.71 ft
>
s
2
a
=2
a
s
2
+
a
u
2
=2
(
-
195.67)2
+
180.71
2
=
266 ft
>
s2 Ans.
*12–168.
For a short time the bucket of the backhoe traces the path
of the cardioid r = 25(1 − cos
u
) ft. Determine themagnitudes
of the velocity and acceleration of the bucket when
u
= 120°
if the boom is rotating with an angular velocity of
u
#
= 2 rad
>
s
and an angular acceleration of
u
$
= 0.2 rad
>
s2 at the instant
shown. u fi 120
r
12–169.
The slotted link is pinned at O, and as a result of the
constant angular velocity it drives the peg Pfor
a short distance along the spiral guide where
is in radians. Determine the velocity and acceleration of
the particle at the instant it leaves the slot in the link, i.e.,
when r=0.5 m.
u
r=10.4 u2m,
u
#
=3 rad>s
0.5 m
r
P
3 rad/sr 0.4u
·
u
12–170.
A part
i
c
l
e moves
i
n t
h
e x–yp
l
ane suc
h
t
h
at
i
ts pos
i
t
i
on
i
s
defined by where tis in seconds.
Determine the radial and transverse components of the
particle’s velocity and acceleration when t=2s.
r=52ti+4t2j6ft,
SOLUTION
f-u=6.9112°
a=8ft>s2
f=tan-1a16
2b=82.875°
v=2(2)2+(16)2=16.1245 ft>s
u=tan-1a16
4b=75.964°
a=8j
v=2i+8tj|t=2=2i+16j
r=2ti+4t2j|t=2=4i+16j
180
12–171.
At the instant shown, the man is twirling a hose over his
head with an angular velocity
u
#
= 2 rad
>
s and an angular
acceleration
u
$
= 3 rad
>
s2. If it is assumed that the hose lies
in a horizontal plane, and water is flowing through it at a
constant rate of 3 m
>
s, determine the magnitudes of the
velocity and acceleration of a water particle as it exits the
open end, r = 1.5 m.
fi 2 rad/s
·
u
fi 3 rad/s2
· ·
u
u
r fi 1.5 m
SOLUTION
r=1.5
r
#
=3
r
$
=0
u
#
=2
u
$
=3
vr=r
#
=3
v
u=
ru
#
=
1.5(2)
=
3
v
=2
(3)2
+
(3)2
=
4.24 m
>
s Ans.
a
r=
r
$-
r(u
#
)
2=
0
-
1.5(2)
2=
6
a
u=
r u
$
+
2r
#
u
#
=
1.5(3)
+
2(3)(2)
=
16.5
a
=2
(6)2
+
(16.5)2
=
17.6 m
>
s2 Ans.
Ans:
v
=4.24 m>s
a
=
17.6 m
>
s
2
