21
Figure S2.20.1
After the enzyme is added the apparent viscosity decreases and is much less sensitive to shear rate, as
determined by the following regression of data (Figure S2.20.2)
0225.0
01.0 −
=
γη
app
. The enzyme
functions by clipping the hyaluronic acid chains decreasing their length. As a result the hyaluronic
acid offers much less resistance to flow.
Figure S2.20.2
2.21. (a) Since the momentum balance is independent of the type of fluid, begin with
Equation 2.7.36 with C2 = 0.
22
23
Note that for r0 = 0, the result for a Newtonian fluid is obtained.
2.22. (a) Since δ = R(1-ε)<<R, the magnitude of the radial position does not vary significantly with a
24
Evaluating the boundary conditions, yields Equation (S2.22.4). Thus, the torque for a power law
fluid is:
Thus, a plot of ln(T) versus ln(ΩR/δ) has a slope equal to n and an intercept equal to ln(2πR2Lm).
2.23. (a) For low rotational speeds, there is only 1 velocity component, vθ. This velocity is a
function of r only. There is no angular variation in velocity or pressure. Thus there is only one shear
stress term, τrθ. Applying a momentum balance, Equation (2.7.57):
(b)
25
26
500 0.01 0.9805
2.25. (a)
τ
rz r=RC
=µdvz
dr r=R
=−µdvz
dy y=
=−µVC
δ
Alternatively, the momentum balance, Equation (2.7.34b), is:
dz =1
r
dr
⎝
⎠
Or after integration and applying the symmetry boundary condition at r = 0.
The more general approach is to consider the fluid in the gap.
(d) From parts (b) and (c)
27
(e) As shown in the table below, there is a minimum in the viscosity at δ/R between 0.2 and 0.3 and
the viscosity increases as δ/R increases.
δ /R
µeff/µ formula 1
µeff/µ formula 2
0.1
3.09
3.43
2.26. (a) From Equation (2.7.36), the shear stress of the flow in a cylindrical tube is:
28
Figure S2.26.1
(d) The wall shear stress (r = R) can be computed from Equation (2.7.36) and is independent of the
29
The enzyme treatment decreased the resistance by 14%. Assuming that the enzyme decreased the
inner radius of the blood vessel by removing the glycocalyx completely, the change is resistance is
due solely to a change in the effective radius of the blood vessel. Thus:
Taking the one-fourth root yields an increase in radius of Renzyme = 1.038R. Assuming that the radius
after enzyme treat is 14.5 µm, then the glycocalyx thickness is 0.038*14.5 µm = 0.551 µm.
30
Solution to Problems in Chapter 3. Section 3.8
3.2. For flow in a cylindrical tube, the friction factor is defined as:
Rearranging Equation (2.7.44) yields a relation between the pressure drop and average velocity:
Substituting Equation (S3.2.4) for Δp in equation (S3.2.1) yields:
3.3. The coordinate system originates at the centerline as shown in Figure 3.19. The boundary
The solution to this problem parallels the case of flow through a cylindrical tube through integration
The stress is not known at any point in the fluid so the boundary condition cannot be evaluated at this
point. Substituting for a Newtonian fluid:
Integrating this expression yields an expression for vz(r) in terms of two constants:
31
Note, that as ε goes to zero, the velocity profile approaches the parabolic profile for laminar flow in a
cylinder. The velocity profile is shown below for values of ε = 0.01, 0.1, 0.5. For all values of ε,
there is a significant distortion of the velocity profile due to the presence of the catheter.
To find the volumetric flow rate, compute the average velocity in the fluid between r and R and
32
The first term in the integral can be obtained by integration by parts (Appendix A1A). Let u=lnr,
Evaluating r between the limits yields:
The left hand side equals the ratio of the flow rate through the annulus to the flow rate through a
cylinder. The term on the right hand side is less than or equal to one. The right hand side reduces to
1 as ε goes to zero. The presence of the annulus has a significant effect on the flow rate that is larger
than the relative reduction in the cross-sectional area (1-ε2). For ε = 0.01, 0.1, 0.5 the flow rate is
respectively, 78.3%, 57.4% and 12.6% of the flow rate in a cylinder.
3.4. The flow rate in the channel is
Inserting the velocity vx (equation (3.4.27)) into the expression for the flow rate.
33
Integrating term by term:
Evaluating the terms in the summation at their limits for z and y and collecting terms yields Equation
(3.8.1).
Defining the term in brackets as g(w/h) and using the one-dimensional definition of the wall shear
stress, 6µQ/wh2, the wall shear stress is estimated as:
The normalized shear stress τw/(Δph/2L) computed from the flow rate (=g(w/h))and the bracketed
term in Equation (3.4.28), f(w/h), indicates a deviation between the two results. The error, shown in
the panel on the right, decreases as the ration w/h increases. The reason for the deviation is that the
one-dimensional approximation for the shear stress, 6µQ/wh2 = Δph/2L, neglects the contribution of
the stresses τzx at ±w/2. Integrating Equation (3.4.7) from –h/2 to h/2 in the y direction and –w/2 to
w/2 in the z direction yields:
The overbar represents the spatial average in the z and y directions for τyx and τzx, respectively.
34
3.5. For w/h=40, the one-dimensional approximation (Figure 3.9) is very good. The time to reach
within 1% of the steady state value can be assessed by adjusting the dimensionless time, th2/ν until
The velocity is within 1% of its steady state value for tν/h2= 0.5. Based on the half-thickness
(4tν/h2) as shown in Figure 3.10, the time dimensionless time to reach steady state is 2.0. For ν =
0.007 cm2 s-1 and h = 0.05 cm, the time to reach steady state is 0.1786 s. Note that the statement on
page 144, line 2 should indicate that for tν/h2 = 0.5 (or 4tν/h2 = 2.0), the solution is within 1% of its
steady state value.
3.6. (a) From equation (3.4.45) the shear stress τyx(y, t) is found as:
35
Evaluating the time derivative at the surface y=-h/2 and normalizing by hL/4νΔp yields:
(b) The normalized gradient is shown below.
(c) The quantity, hL/4νΔp can be rewritten as h2/8ντw. Multiplying the normalized gradients by 8ντw/h2
yields the dimensional gradient. For and ν = 0.007 cm2 s-1, h = 0.015 cm, h = 0.05 cm and τw = 1 dyne
cm-2, the gradients are shown below. The shear stress gradients are very different. To ensure constant
3.7. The unsteady velocity profile for flow in a cylindrical tube of radius R is:
36
The following m-file was written to solve equation 2
Panel A of the figure shows the relative velocity as a function of dimensionless time. To identify
when the profile is within 1% of steady state the unsteady term (second term on the right hand side
of equation 2) was isolated and plotted for various values of the dimensionless time t. The solution is
3.8. The force balance is the same that presented in equation (3.6.29) and shown in Figure 3.17.
Thus, the velocity vz is obtained from equation (3.6.30) after a change in notation:
37
Inserting the expression for r (z) and expressing the velocity as the derivation of the z position with
Rewriting in terms of the time constant for particle settling, tc = 2rpa2/9µ,
where ρ = ρ0/ρπ and α’= α/ρπ.
Rearranging:
Applying the initial condition t = 0 z = z0, C1 = ln(1-ρ–α’z0). The solution becomes:
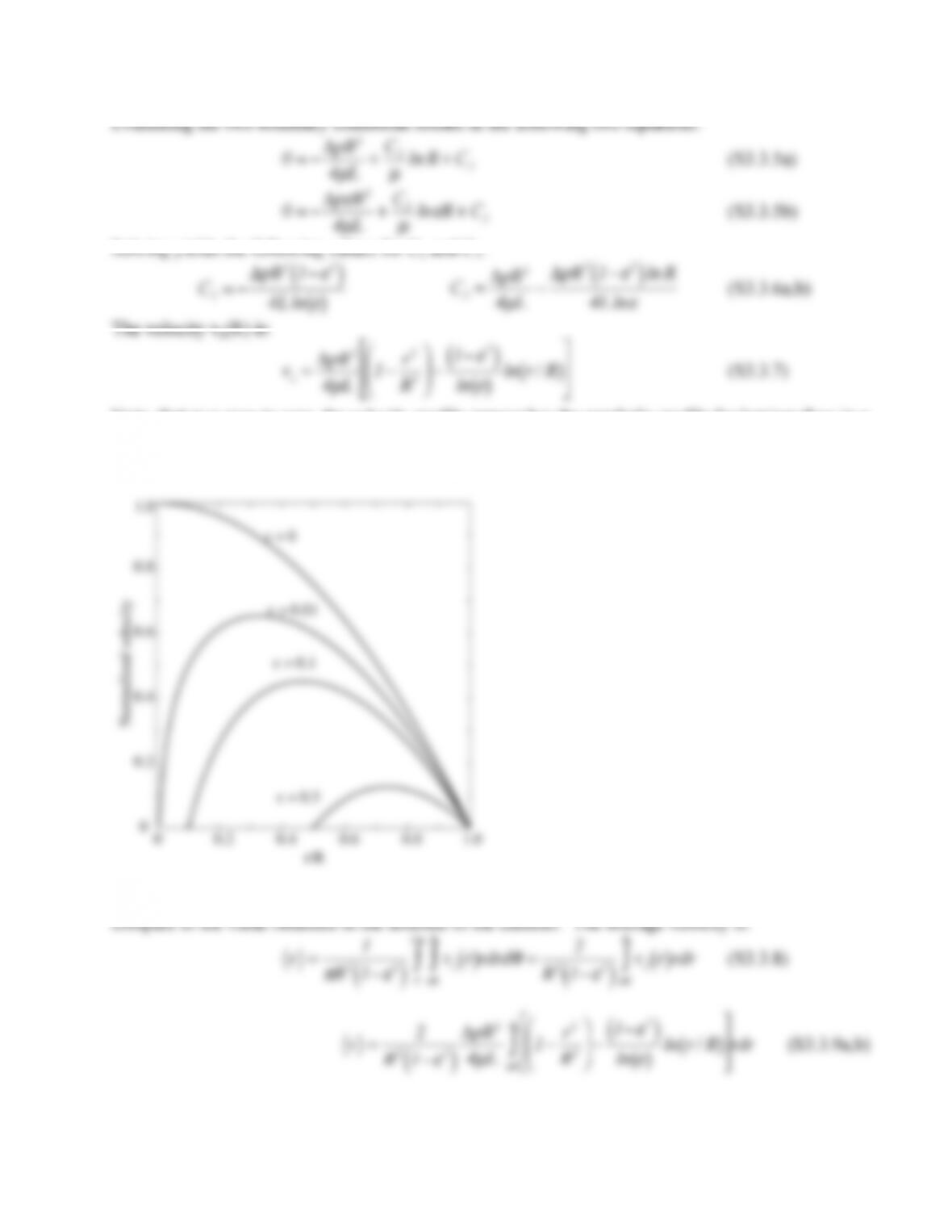
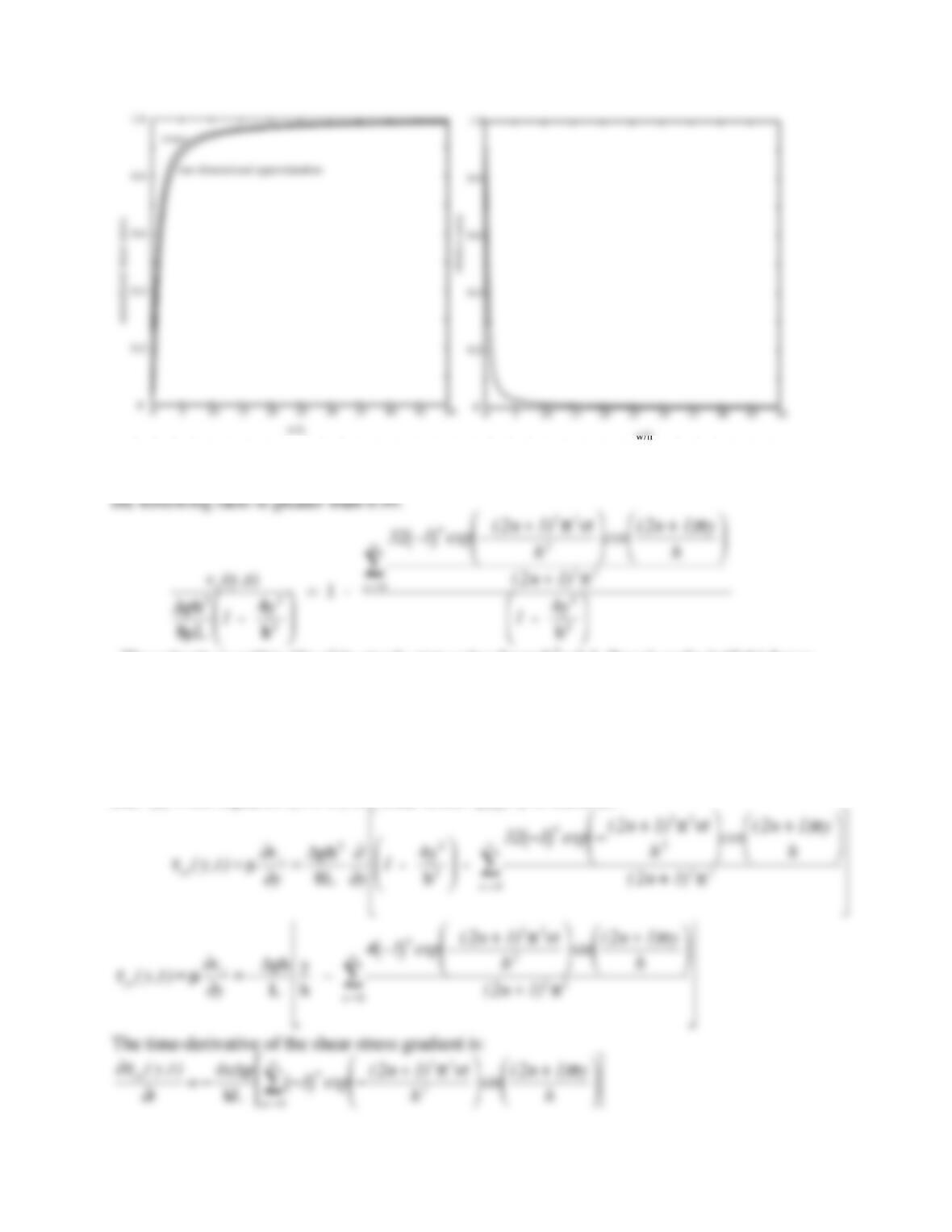
The exponential is less than 0.01 and ρ0 + αz0 ≈ ρπ for t > 5/α’tcg.
3.9. The time rate of change in cell length in the pipet L’ is a function of the following variables:
There are three dimensions (m L t) and five dimensional groups. Thus, according to the Pi theorem
there are two dimensionless groups. Choose the following basis group which contains all three
dimensions (µc)a(ΔP)b(RP)c. The two dimensionless groups will include L’ and Rc.
1
L’µc(ΔP)-1RP-1
The group L’µcRP-1 represents viscous stresses in the cell which are balanced by the applied pressure.
The second dimensionless group can be found, using the same approach, to be RcRP-1.
3.10. The force F acting on the leukocyte is a function of the following variables:
F =f(dc,Dt, vc, vf, Hct, ρ, µ)
While there are eight variables, the hematocrit Hct is dimensionless. So there are 7 dimensional
variables and three characteristic dimensions, mass, length and time. Thus, in addition, there are four
38
other dimensionless groups. If we choose the basis group as (µ)a(Vf)b(dt)c, the four other variables
to make dimensionless are F, ρ, Dc and vc. While the Reynolds number is clearly one of the groups
3.11. (a) For two-dimensional flow of an incompressible fluid, the conservation of mass yields:
When the fluid contacts the sold surface the no slip condition results in fluid deceleration in the x-
Since the derivative is positive within the boundary layer, vy is positive throughout this region.
39
(b) Assume that vx can be represented as: vx = a1(x)y + a2(x)y2 + .… This velocity profile
guarantees that vx is zero at y = 0. At y = δ we expect that vx = U. Just using the first term,
application of the boundary condition at y = d yields:
vx = Uy/δ
Note that δ is increasing with x so its derivative with respect to x is positive. Integrating this
result yields the following:
vy=Uy2
δ
2
d
δ
dx
since vy = 0 at y=0. The resulting shape is a
parabola.
(c) For the case in which the velocity profile is limited to two terms, vx = a1(x)y + a2(x)y2, the
boundary conditions are satisfied when U = a1δ + a2δ2 and the first derivative at y = δ equals zero
results in a1 =-2 a2δ. This latter condition ensures that the shear stress is continuous. As a result, a2
= -U/δ2 and a1 = 2U/δ. The resulting velocity profile is: vy = U(2y/δ – y2/δ2). The assumption that
the velocity gradient is zero at y = δ and the parabolic shape of the profile ensure that the velocity is
a maximum at y = δ.
3.12. (a) Using the dimensionless variables listed, equation (3.8.5a) becomes:
The group ραωR2/µ represents the ratio of inertial to viscous forces.
The dimensionless form of the z-component is
40
(b) Assuming that ραωR2/µ << 1 and α<<1, Equations (S3.12.1), (S3.12.2) and (S3.12.3) simplify
(1) If inertial forces are much smaller than viscous forces, then vr does not change in the r direction
and must nut depend on r since vr = 0 at r = 0 . From equation (S3.13.4), vr* = C1z*+ C2. From the
boundary condition at z = z* = 0, C2 = 0. In order to satisfy the boundary condition at z = h or z* =
h/αR, C1 must equal 0. Therefore vr* = 0 throughout.
(2) As a result, the conservation of mass reduces to:
Integrating, vz* = C3. The only way in which both boundary conditions could be satisfied (vz* = 0 at
z = 0 and vz* = ωr a z= h(α)) is for vz* = 0.
(3) Note that the problem statement should refer to the θ component of the conservation of linear
momentum, not mass.
Based on the results obtained above, the only nonzero component of velocity
is vθ. As a result, equation (3.8.5b) reduces to
Integrating twice yields:
The boundary conditions are that at z = 0, vθ* = 0 and at z = h(α), vθ* = ωr. From the condition at z
= 0, C2 = 0. From the condition at z = h(α),C1 = ωr/h(α). The resulting expression for vθ* is:
which agrees with Equation (3.8.3).
(c) The shear stress at the plate surface is obtained by inserting equation (3.8.6) into equation