Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
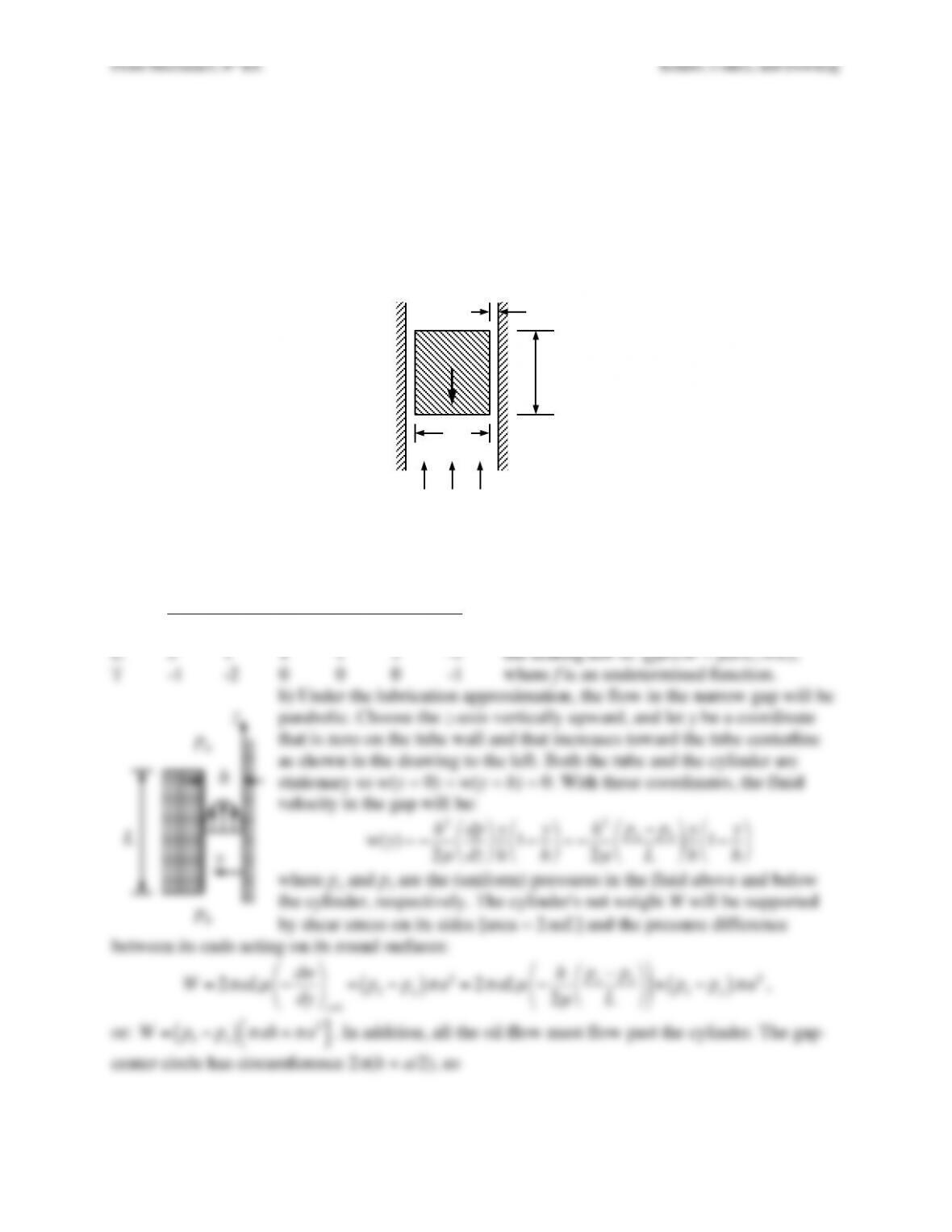
Exercise 9.17. For lubrication flow under the sloped bearing of Example 9.3, the assumed
velocity profile was
u(x,y)=−1 2
µ
( )
dP dx
( )
y h(x)−y)
( )
+Uy h(x)
, the derived pressure was
P(x)=P
e+3
µ
U
α
ho
2L
( )
x(L−x)
, and the load (per unit depth) carried by the bearing was
W=
µ
U
α
L22ho
2
. Use these equations to determine the frictional force (per unit depth), Ff,
applied to the lower (flat) stationary surface in terms of W, ho/L, and
α
. What is the spatially-
averaged coefficient of friction under the bearing?
Solution 9.17. For lubrication flow under the sloped bearing, the velocity field was assumed to
be:
u(y)=−1
2
µ
∂
p
∂
xy(h−y)+Uy
h
, so the wall shear stress is:
τ
w=
µ∂
u
∂
y
$
%
&
‘
(
)
y=0
=−h
2
∂
p
∂
x+
µ
U
h
.
Integrate this from x = 0 to x = L to find the friction force Ff per unit depth. Here the pressure
gradient will be:
dp dx =3
µ
U
α
ho
2L
( )
(L−2x)
, so
Ff=
τ
wdx =−ho(1−
α
x L)
2
3
µ
U
α
ho
2L(L−2x)+
µ
U
ho(1−
α
x L)
%
&
‘
(
)
*
0
L
∫
0
L
∫dx
Both integrals are elementary, so Ff is found to be:
.
However, because
α
is small, only the
α
0 and
α
1 terms should be reported so,
Ff≅
µ
UL
ho
1+
α
2
$
%
& ‘
(
)
,
or using
W=
µ
U
α
L2
2ho
2
,
Ff≅Who
α
L
2+
α
( )
. The coefficient of friction is a ratio:
Ff
W≅ho(2 +
α
)
α
L≈2ho
α
L
.
Hence, we can see that it is not possible to obtain a small coefficient of friction since the
maximum value of
α
is ~ho/L. Thus, the purpose of this type of bearing would not necessarily be
to produces a small coefficient of friction. Instead it prevents scuffing and wear since the
presence of the oil film prevents the bearing pad and the surface from coming into contact.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.18. A bearing pad of total length 2L moves to the right at constant speed U above a
thin film of incompressible oil with viscosity µ and density
ρ
. There is a step change in the gap
thickness (from h1 to h2) below the bearing as shown. Assume that the oil flow under the bearing
pad follows:
u(y)=−y(hj−y)
2
µ
dP(x)
dx +Uy
hj
, where j = 1 or 2. The pad is instantaneously
aligned above the coordinate system shown. The pressure in the oil ahead and behind the bearing
is Pe.
a) By conserving mass for the oil flow, find a relationship between µ, U, hj, dP/dx, and an
unknown constant C.
b) Use the result of part a) and continuity of the pressure at x = 0, to determine
P(0) −P
e=6
µ
UL(h1−h2)
h2
3+h1
3
c) Can this bearing support a externally applied downward load when h1 < h2?
Solution 9.18. a) Use a CV attached to the bearing pad with vertical flux surfaces that span the
gap. Conservation of mass implies:
u(y)−U
( )
dy
0
hj
∫=C
→
−y(hj−y)
2
µ
dP(x)
dx +Uy
hj
−U
#
$
%
%
&
‘
(
(
dy
0
hj
∫=C
Performing the integration produces:
=6
h1
2
h1
3+h2
3
$
%
‘
( =6
h1
2
h1
3+h2
3
$
%
‘
( =6
µ
UL h1−h2
h1
3+h2
3
$
%
‘
(
c) When h1 < h2, the pressure at x = 0 is less than Pe. This means that the bearing pad is sucked
toward the surface, so when h1 < h2 the bearing cannot support an external load.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.19. A flat disk of radius a rotates above a solid boundary at a steady rotational speed
of Ω. The gap, h (<< a), between the disk and the boundary is filled with an incompressible
Newtonian fluid with viscosity µ and density
ρ
. The pressure at the edge of the disk is p(a).
a) Using cylindrical coordinates and assuming that the only non-zero velocity component is
u
ϕ
(R,z), determine the torque necessary to keep the disk turning.
b) If p(a) acts on the exposed (upper) surface of the disk, will the pressure distribution on the
disk’s wetted surface tend to pull the disk toward or push it away from the solid boundary?
c) If the gap is increased, eventually the assumption of part a) breaks down. What happens?
Explain why and where ur and uz might be non-zero when the gap is no longer narrow.
Solution 9.19. a) By analogy with plane Couette flow, set
u
ϕ
(R,z)=ΩR(z/h)
. The shear stress
on any element of the rotating disk will be:
τ
=
µ
(du
ϕ
/dz)=
µ
ΩR/h
. The torque necessary to
keep the disk turning will be:
Torque =R
τ
dA
r=0
r=a
∫=2
π
(
µ
Ω/h)R3dR
r=o
r=a
∫=
πµ
Ωa42h
.
b) For the assumed solution used to determine the answer to part a) to be valid, the quadratic
velocity terms in the conservation of momentum equation must approach zero. Thus, the radial
momentum equation:
−
ρ
u
ϕ
2
R≈ −
∂
p
∂
R
suggests that the radial pressure gradient will be very small
but positive because u
ϕ
is non-zero just underneath the rotating disk. Thus, the pressure near the
axis of rotation may be slightly lower. This suggests the disk may be mildly drawn downward as
it rotates.
c) If the gap increases in size, a secondary flow with closed streamlines will develop that has
fluid moving away from the axis of rotation near the spinning disk, downward as the fluid
approaches R = a, toward the axis of rotation near the stationary disk, and upward near the axis
of rotation. This secondary flow will be driven by the radial pressure gradient mentioned above
in part b).
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.20. A circular block with radius a and weight W is released at t = 0 on a thin layer of
an incompressible fluid with viscosity
µ
that is supported by a smooth horizontal motionless
surface. The fluid layer’s initial thickness is ho. Assume that flow in the gap between the block
and the surface is quasi-steady with a parabolic velocity profile:
uR(R,z,t)=−dP(R)dR
( )
z h(t)−z)
( )
2
µ
where R is the distance from the center of the block, P(R) is the pressure at R, z is the vertical
coordinate from the smooth surface, h(t) is the gap thickness, and t is time.
a) By considering conservation of mass, show that:
dh dt =(h36
µ
R)(dP dR)
.
b) If W is known, determine h(t) and note how long it takes for h(t) to reach zero.
Solution 9.20. Consider a circular control volume under the block with radius of R and height
h(t). The integral form of conservation of mass implies:
π
R2
ρ
dh
dt =−2
π
R
ρ
uR(R,z,t)dz
0
h(t)
∫=2
π
R
ρ
1
2
µ
dP(R)
dR
z h(t)−z)
( )
dz
0
h(t)
∫=
π
R
ρ
µ
dP(R)
dR
h3
6
which simplifies to:
dh
dt =h3
6
µ
R
dP(R)
dR
.
b) Since h does not depend on R, this equation can be integrated in the radial direction from R to
a:
dh
dt
RdR =h3
6
µ
dP
R
a
∫
R
a
∫
which implies
dh
dt
a2−R2
2
#
$
%
&
‘
( =h3
6
µ
P
e−P(R)
( )
,
where Pe is the exterior pressure on the circular block (perhaps equal to 1 atm). Assuming quasi-
steady conditions, the weight of the block must be balanced by an integral of the excess-pressure
underneath the block:
W=2
π
P(R)−P
e
( )
0
a
∫RdR =−dh
dt
6
πµ
h3a2−R2
( )
0
a
∫RdR =−dh
dt
6
πµ
h3a2a2
2−a4
4
%
&
‘
(
)
* =−dh
dt
3
πµ
a4
2h3
.
Now, treat this result as a first-order nonlinear differential equation for h(t) and integrate in time:
h−3dh
ho
h(t)
∫=−1
2
1
h2−1
ho
2
$
%
&
‘
(
) =−2Wt
3
πµ
a4
which implies
1
h2(t)=1
ho
2+4Wt
3
πµ
a4
.
Solving for h(t) yields:
h(t)=ho1+4Who
2t
3
πµ
a4
#
$
%
&
‘
(
−1 2
. For this solution, h(t) → 0 only when t → ∞.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.21. Consider the inverse of the previous exercise. A block and smooth surface are
separated by a thin layer of a viscous fluid with thickness ho. At t = 0, a force, F, is applied to
separate them. If ho is arbitrarily small can the block and plate be separated easily? Perform some
tests in your kitchen. Use maple syrup, peanut butter, liquid soap, pudding, etc. for the viscous
liquid. The flat top-side of a metal jar lid or the flat bottom of a drinking glass make a good
circular block. (Lids with raised edges and cups & glasses with ridges or sloped bottoms do not
work well). A flat countertop or the flat portion of a dinner plate can be the motionless smooth
surface. Can the item used for the block be more easily separated from the surface when tilted
relative to the surface? Describe your experiments and try to explain your results.
Solution 9.21. Using a Formica counter-top and the underside of a coffee-cup saucer, a
compelling case can be made for the accuracy of the result of Exercise 9.20. If an upward force
F is applied to the flat-bottomed block, the gap distance as a function of time is:
h(t)=ho1−4Fho
2t
3
πµ
a4
$
&
‘
)
−1 2
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.22. A rectangular slab of width 2L (and depth B into the page) moves vertically on a
thin layer of oil that flows horizontally as shown. Assume
u(y,t)=−h22
µ
( )
dP dx
( )
y h
( )
1−y h
( )
where h(x,t) is the instantaneous gap between the slab
and the surface,
µ
is the oil’s viscosity, and P(x,t) is the pressure in the oil below the slab. The
slab is slightly misaligned with the surface so that
h(x,t)=ho1+
α
x L
( )
+˙
h
ot
where
α
<< 1 and
˙
h
o
is the vertical velocity of the slab. The pressure in the oil outside the slab is Po. Consider the
instant t = 0 in your work below.
a) Conserve mass in an appropriate CV to show that:
∂
h
∂
t+
∂
∂
xu(y,t)dy
0
h(x,t)
∫
( )
=0
.
b) Keeping only linear terms in
α
, and noting that C and D are constants, show that:
P(x,t=0) =12
µ
ho
3
˙
h
ox2
21−2
α
x
L
$
%
& ‘
(
) +Cx 1−3
α
x
2L
$
%
& ‘
(
)
$
%
&
‘
(
) +D
.
c) State the boundary conditions necessary to evaluate the constants C and D.
d) Evaluate the constants to show that the pressure distribution below the slab is:
P(x,t)−P
o=−6
µ
˙
h
oL2
ho
3(t)1−x L
( )
2
( )
1−2
α
x
L
$
%
& ‘
(
)
.
e) Does this pressure distribution act to increase or decrease alignment between the slab and
surface when the slab is moving downward? Answer the same question for upward slab motion.
Solution 9.22. a) Use the differential CV shown to the right and
note that the upper boundary is moving at speed
b=dh dt
( )
ey=˙
h
oey
but there is no mass flux through it. The other
boundaries are stationary. Thus, Cons. of Mass,
!”#
$““#!”$%%#
$““$%%#
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
d) Using the results of part b) and c), leads to two equations for the constants C and D.
P(–L)=P
o=12
µ
ho
3
˙
h
o
L2
21+2
α
( )
−CL 1+3
α
2
$
%
& ‘
(
)
$
%
&
‘
(
) +D
, and
P(+L)=P
o=12
µ
ho
3
˙
h
o
L2
21−2
α
( )
+CL 1−3
α
2
$
%
& ‘
(
)
$
%
&
‘
(
) +D
.
These can be solved to find:
C=˙
h
oL
α
and
D=P
o−6
µ
L2
ho
3
˙
h
o
,
where quadratic terms in
α
have been dropped. Thus, the pressure distribution is:
P(x)=12
µ
ho
3
˙
h
o
x2
21−2
α
L
x
$
%
& ‘
(
) +˙
h
oL
α
x1−3
α
2L
x
$
%
& ‘
(
)
$
%
&
‘
(
) +P
o−6
µ
L2
ho
3
˙
h
o
=P
o+6
µ
L2
ho
3
˙
h
o
x2
L21−2
α
L
x
$
%
& ‘
(
) +2
α
x
L1−3
α
2L
x
$
%
& ‘
(
) −1
$
%
&
‘
(
)
=P
o−6
µ
L2
ho
3
˙
h
o1−x2
L2+2
α
L3x3−2
α
L
x+…
$
%
&
‘
(
)
=P
o−6
µ
L2
ho
3
˙
h
o1−x2
L2
$
%
&
‘
(
) 1−2
α
L
x
$
%
& ‘
(
) +…
where again quadratic terms in
α
have been dropped.
e) When the slab is moving downward (so
˙
h
o
is negative), the highest pressure point lies in the
region where the gap is the smallest and this pressure distribution causes a moment on the slab
that tends to align the slab with the surface. This is beneficial in lubrication flows because the
oil pressure acts to prevent contact between slab and the surface. When the slab is moving
upward, the lowest pressure lies in the region where the gap is the smallest and this pressure
distribution causes a moment on the slab that tends to misalign the slab with the surface. These
phenomena are readily apparent when pressing or lifting a flat object from a wet or oily surface.
In particular, it may be nearly impossible to lift the object straight up; however, once one corner
or edge is tilted up, the object is much easier to lift completely.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.23. Show that the lubrication approximation can be extended to viscous flow within
narrow gaps h(x,y,t) that depend on two spatial coordinates. Start from (4.10) and (9.1), and use
Cartesian coordinates oriented so that x–y plane is locally tangent to the center-plane of the gap.
Scale the equations using a direct extension of (9.14):
x* = x/L , y* = y/L , z* = z/h = y/
ε
L , t* = Ut/L , u* = u/U , v* = v/U , w* = w/
ε
U , and p* = p/Pa.
where L is the characteristic distance for the gap thickness to change in either the x or y direction,
and
ε
= h/L. Simplify these equation when
ε
2ReL → 0, but
µ
UL/Pah2 remains of order unity to
find:
0≅ − 1
ρ
∂
p
∂
x+
∂
2u
∂
z2
,
0≅ − 1
ρ
∂
p
∂
y+
∂
2v
∂
z2
, and
0≅ − 1
ρ
∂
p
∂
z
.
Solution 9.23. In Cartesian coordinates the starting equations (4.10) and the three components of
(8.1) are:
∂
u
∂
x+
∂
v
∂
y+
∂
w
∂
z=0
,
x:
∂
u
∂
t+u
∂
u
∂
x+v
∂
u
∂
y+w
∂
u
∂
z=−1
ρ
∂
p
∂
x+
ν∂
2u
∂
x2+
∂
2u
∂
y2+
∂
2u
∂
z2
&
‘
(
)
*
+
,
y:
∂
v
∂
t+u
∂
v
∂
x+v
∂
v
∂
y+w
∂
v
∂
z=−1
ρ
∂
p
∂
y+
ν∂
2v
∂
x2+
∂
2v
∂
y2+
∂
2v
∂
z2
&
‘
(
)
*
+
, and
z:
∂
w
∂
t+u
∂
w
∂
x+v
∂
w
∂
y+w
∂
w
∂
z=−1
ρ
∂
p
∂
z+
ν∂
2w
∂
x2+
∂
2w
∂
y2+
∂
2w
∂
z2
&
(
)
+
.
Multiply through by
ρ
h2/
µ
U, and use
ε
= h/L and ReL =
ρ
UL/
µ
to reach:
ε
2ReL
∂
u*
∂
t*+u*
∂
u*
∂
x*+v*
∂
u*
∂
y*+w*
∂
u*
∂
z*
$
%
&
‘
(
) =−Pah2
µ
UL
∂
p*
∂
x*+
ε
2
∂
2u*
∂
x*2+
∂
2u*
∂
y*2
$
%
&
‘
(
) +
∂
2u*
∂
z*2
.
When
ε
2ReL → 0, but
µ
UL/Pah2 remains of order unity this equation simplifies to:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
The procedure for the y-momentum equation is the same as that for the x-momentum equation
with u replaced by v and ∂p/∂x replaced by ∂p/∂y. The final dimensionless and dimensional
forms are:
0≅ − Pah2
µ
UL
∂
p*
∂
y*+
∂
2v*
∂
z*2
, or in dimensional form:
0≅ − 1
ρ
∂
p
∂
y+
ν∂
2v
∂
z2
.
The z-momentum equation is different that the other two because the scaling of w differs
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.24. A squeegee is pulled across a smooth flat stationary surface at a constant speed U.
The gap between the squeegee and surface is h(x) = hoexp{+
α
x/L} and this gap is filled with a
fluid of constant density
ρ
and viscosity
µ
. If the squeegee is wetted by the fluid for 0 ≤ x ≤ L,
and the pressure in the surrounding air is pe, what is the pressure p(x) distribution under the
squeegee and what force W perpendicular to the surface is needed to hold the squeegee in place?
Ignore gravity in your work.
Solution 9.24. Follow the solution approach in Example 9.3. Here the pressure gradient will be:
dp(x)
dx =−12
µ
h3(x)C1−6
µ
U
h2(x)=−12
µ
ho
3C1e−3
α
x L −6
µ
U
ho
2e−2
α
x L
.
Integrate to find the pressure distribution:
p(x)= +12
µ
L
3ho
3
α
C1e−3
α
x L +6
µ
UL
2ho
2
α
e−2
α
x L +C2=3
µ
UL
ho
2
α
4
3Uho
C1e−3
α
x L +e−2
α
x L
“
#
$%
&
‘+C2
.
The boundary conditions are p(0) = p(L) = pe, and these lead to two equations for the two
constants C1 and C2.
pe=3
µ
UL
ho
2
α
4
3Uho
C1+1
!
“
#$
%
&+C2
, and
pe=3
µ
UL
ho
2
α
4
3Uho
C1e−3
α
+e−2
α
“
#
$%
&
‘+C2
. (1,2)
Subtract (2) from (1) to find C1:
0=3
µ
UL
ho
2
α
4
3Uho
C1(1−e−3
α
)+1−e−2
α
“
#
$%
&
‘
or
C1=−3Uho
4
1−e−2
α
1−e−3
α
.
Substitute this into (1) to find C2:
C2=pe−3
µ
UL
ho
2
α
e−2
α
−e−3
α
1−e−3
α
“
#
$%
&
‘
.
Use these constants to construct p(x):
p(x)−pe=3
µ
UL
ho
2
α
−1−e−2
α
1−e−3
α
e−3
α
x L +e−2
α
x L −e−2
α
−e−3
α
1−e−3
α
“
#
$%
&
‘
.
The force (or load) W is determined from the integral:
W=p(x)−pe
( )
0
L
∫dx =3
µ
LU
ho
2
α
−1−e−2
α
1−e−3
α
e−3
α
x L +e−2
α
x L −e−2
α
−e−3
α
1−e−3
α
#
$
%&
‘
(dx
0
L
∫
.
The integration of the exponentials may be done directly:
W=3
µ
LU
ho
2
α
−1−e−2
α
1−e−3
α
e−3
α
x L
−3
α
L
“
#
$%
&
‘
0
L
+e−2
α
x L
−2
α
L
“
#
$%
&
‘
0
L
−e−2
α
−e−3
α
1−e−3
α
x
[ ]
0
L
(
)
*
*
+
,
–
–
.
Continue with the evaluation:
U!
x!
x = L!
x = 0!
h(x)!
pe!
pe!
W!
p(x) = ?!
ho!
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
W=3
µ
LU
ho
2
α
L
3
α
1−e−2
α
1−e−3
α
e−3
α
−1
( )
−L
2
α
e−2
α
−1
( )
−e−2
α
−e−3
α
1−e−3
α
L
“
#
$%
&
‘
=3
µ
LU
ho
2
α
L
6
α
1−e−2
α
( )
−e−2
α
−e−3
α
1−e−3
α
L
“
#
$%
&
‘
And, when
α
<< 1, this reduces to
W=
αµ
L2U
2ho
2
, which is the same as the result of Example 9.3.
This occurs because the two shapes become the same; h(x) = hoexp{+
α
x/L} ≈ ho(1 +
α
x/L) when
α
<< 1.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.25. A close-fitting solid cylinder with net weight W (= actual weight – buoyancy),
length L, and radius a is centered in and may slide along the axis of a long vertical tube with
radius a + h, where h << a. The tube is filled with oil having constant viscosity
µ
that is pumped
slowly upward at a volume flow rate Q.
a) Use dimensional analysis to find a scaling law for the value of Q that holds the cylinder
stationary when fluid inertia is unimportant.
b) Use the lubrication approximation and assume that the pressure is uniform above and below
the cylinder to determine a formula for the value of Q that holds the cylinder stationary.
Solution 9.25. a) There are six parameters (Q, W, L, a, h,
µ
) so the units matrix (which has rank
3) is:
Q W L a h
µ.
The 6 – 3 = 3 dimensionless groups are:
M 0 1 0 0 0 1 Π1 = Q
µ
/LW, Π2 = a/L, and Π3 = h/L, so
L 3 1 1 1 1 -1 the scaling law is: Q
µ
/LW = f(a/L, h/L),
L!
h!
2a!
Q!
W!
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.26. A thin film of viscous fluid is bounded below by a flat stationary plate at z = 0. If
the in-plane velocity at the upper film surface, z = h(x,y,t), is U = U(x,y,t)ex + V(x,y,t)ey, use the
equations derived in Exercise 8.19 to produce the Reynolds equation for constant-density thin-
flim lubrication:
∇ ⋅ h3
µ
$
%
&
‘
(
)
∇p
*
+
,
–
.
/ =12
∂
h
∂
t+6∇ ⋅ hU
( )
where
∇=ex
∂ ∂
x
( )
+ey
∂ ∂
y
( )
merely involves the two in-plane dimensions.
Solution 9.26. For the specified geometry, solutions for the x– and y-direction equations are
given by appropriately evaluated versions of (8.19):
u≅ − h2
2
µ
∂
p
∂
x
z
h
1−z
h
%
&
‘ (
)
* +Uz
h
, and
v≅ − h2
2
µ
∂
p
∂
y
z
h
1−z
h
%
&
‘ (
)
* +Vz
h
.
where h, U, and V are functions of x, y, and t.
Now consider a stationary nearly-rectangular control volume of with elemental base area
∇=ex
x
( )
+ey
y
( )
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 8.21. Fluid of density
ρ
and viscosity µ flows inside a long tapered tube of length L and
radius R(x) = (1 –
α
x/L)Ro where
α
< 1, and Ro << L.
a) Estimate the volume discharge rate Qv through the tube, for a given pressure difference Δp
sustained between the inlet and the outlet.
b) Discuss the range of validity of your solution in terms of the parameters of the problem.
Solution 8.21. a) If the tube diameter changes slowly enough, the flow will be in equilibrium
with the local diameter of the pipe and the axial velocity profile will be the same as that in an
untapered pipe of the same diameter. For flow in an untapered pipe:
Qv=Uave A
, and
Uave =−d2
32
µ
dp
dx
. For this problem let
Uave =Uave (x)
since A = A(x) and
Qv=Uave (x)A(x)=const
, therefore:
Uave (x)=−R2(x)
8
µ
dp
dx
. Eliminate
Uave (x)
from the last two
equations and use
A(x)=
π
R2(x)
to get:
Qv=−R2(x)
8
µ
dp
dx A(x)=−
π
R4(x)
8
µ
dp
dx
(1)
Now solve for
−dp dx
and integrate in x from 0 to L:
−p(L)−p(0)
( )
=8
µ
Qv
π
R4(x)
0
L
∫dx =8
µ
Qv
π
Ro
41−
α
x
L
&
‘
( )
*
+
−4
0
L
∫dx =8
µ
QvL
π
Ro
4
αζ
−4
1
1−
α
∫d
ζ
The integral is elementary and is easily evaluated, yielding:
p(0) −p(L)=8
µ
QvL
3
π
Ro
4
α
1
(1−
α
)3−1
%
&
‘ (
)
*
.
Inverting this to find Qv produces:
Qv=3
π
Ro
4
α
8
µ
Lp(0) −p(L)
( )
1
(1−
α
)3−1
%
&
‘ (
)
*
−1
.
This result should be expanded for
α
<< 1,
1
(1−
α
)3−1
$
%
& ‘
(
)
−1
=(1−
α
)3
1−(1−
α
)3=1−3
α
+3
α
2−
α
3
3
α
−3
α
2+
α
3=1
3
α
1−3
α
+…
1−
α
+…
$
%
& ‘
(
) ≈1
3
α
1−3
α
( )
(1+
α
)≈1−2
α
3
α
.
Therefore:
Qv=
π
Ro
4
8
µ
p(0) −p(L)
L
$
%
& ‘
(
)
(1−2
α
)
,
so for a fixed pressure difference Qv is larger when the pipe expands (
α
< 0) and is smaller when
the pipe contracts (
α
> 0).
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
b) The flow accelerates or decelerates inside a contracting or expanding pipe, respectively. The
above solution will only be valid if fluid acceleration can be ignored compared to
1
ρ
dp
dx
or
ν
∇2u
(recall that these two terms are set equal to obtain the solution for the velocity profile). Hence
we need only consider one of them, and therefore must have:
u
∂
u
∂
x<< 1
ρ
dp
dx
where
u
∂
u
∂
x
is a
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.28. A circular lubricated bearing of radius a holds a stationary round shaft. The
bearing hub rotates at angular rate Ω as shown. A load per unit depth on the shaft, W, causes the
center of the shaft to be displaced from the center of the rotating hub by a distance
ε
ho, where ho
is the average gap thickness and ho << a. The gap is filled with an incompressible oil of viscosity
µ. Neglect the shear stress contribution to W.
a) Determine a dimensionless scaling law for |W|.
b) Determine W by assuming a lubrication flow profile in the
gap and h(
θ
) = ho(1 +
ε
cos
θ
) with
ε
« 1.
c) If W is increased a little bit, is the lubrication action
stabilizing?
Solution 9.28. a) Follow the usual dimensional analysis
approach. The flow is caused by the balance of pressure and
viscous forces, so the fluid inertia (i.e.
ρ
) doesn’t matter.
• Boundary condition & material parameters: µ = viscosity of the fluid
Ω = rotation speed of the shaft
b) Because the fluid gap is very narrow, consider a new coordinate: z that is zero on the surface
of the shaft and equal to h(
θ
) at the outer rotating bearing surface, and
ξ
= a
θ
= downstream
distance. Place a stationary control volume in the gap that encloses the fluid in the gap between
angles
θ
1 and
θ
2. Conservation of mass for the gap-flow will imply that:
u(z)dz
0
h
∫
[ ]
θ
=
θ
1
=u(z)dz
0
h
∫
[ ]
θ
=
θ
2
=C1=const.
(Note, this situation is subtly different than the sloped bearing worked in lecture; in the
unwrapped geometry, the upper wall is moving but its geometric shape does not move.)
Conservation of momentum in the angular direction in the gap will be satisfied locally by using
the Couette flow solution for u(z): i.e.
C1=−z(h−z)
2
µ
a
dP
d
θ
+Ωaz
h
%
&
‘
(
)
*
dz
0
h
∫=−h3
12
µ
a
dP
d
θ
+Ωah
2
Treat this result as a differential equation for P(
θ
):
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
dP
d
θ
=−12
µ
aC1+6
µ
Ωa2h
h3=−12
µ
aC1+6
µ
Ωa2ho(1+
ε
cos
θ
)
ho
3(1+
ε
cos
θ
)3
Even though integration of this equation can be completed exactly, it’s tedious and unnecessary
because
ε
<< 1. Therefore, expand for
ε
<< 1 and only keep the linear terms in
ε
:
dP
d
θ
=−12
µ
aC1+6
µ
Ωa2ho
ho
3+36
µ
aC1−12
µ
Ωa2ho
ho
3
ε
cos
θ
+…
Integrate from 0 to
θ
:
P(
θ
)−P(0) =−12
µ
aC1+6
µ
Ωa2ho
ho
3
θ
+36
µ
aC1−12
µ
Ωa2ho
ho
3
ε
sin
θ
+…
For the pressure to be single valued [i.e. P(2π) = P(0)], we must have C1 = Ωaho/2 (this makes
the linear-in–
θ
term in the last equation equal to zero). Therefore
.
Now use this pressure distribution to determine the load on the shaft and ignore any net
contributions from shear stresses. The external load on the shaft, which is equal an opposite to
the fluid load applied to the shaft, may have both x– and y-components, so
W=(Wx,Wy)=−P(0) −P(
θ
)
( )
n ad
θ
0
2
π
∫
,
where
n=(cos
θ
,sin
θ
)
is the outward normal from the shaft’s surface.
W=6
µ
Ωa3
ε
ho
2sin
θ
cos
θ
,sin
θ
( )
d
θ
0
2
π
∫=0,+6
πµ
Ωa3
ε
ho
2
‘
(
)
*
+
,
This answer matches the dimensional analysis result. However, the external load applied to the
shaft pushes the shaft up! This seemingly odd result, makes sense if you think about what’s
going on. For the fluid to pass through the narrowest part of the gap at
θ
= π where the viscous
forces are largest, there must be a favorable (i.e negative) dP/d
θ
in this region. This means that
the pressure above the shaft (near
θ
= π/2) must be greater than the pressure below the shaft
(near
θ
= 3π/2). With high pressure above and low pressure below, the lubricant must be trying
to push the shaft down. In order to maintain shaft position, there must be an upward-directed
externally-applied load on the shaft.
c) If Wy is impulsively increased by a small amount, the shaft will move vertically. The
lubrication action will then push the shaft to the left. Eventually a new steady state will be
reached with the shaft misaligned in the bearing (shaft center to the left) with a larger value of
ε
than the original configuration. So, the lubrication action can be considered stabilizing.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.29. As a simple model of small-artery blood flow, consider slowly varying viscous
flow through a round flexible tube with inlet at z = 0 and outlet at z = L. At z = 0, the volume flux
entering the tube is
Qo(t)
. At z = L, the pressure equals the exterior pressure pe. The radius of the
tube, a(z,t), expands and contracts in proportion to pressure variations within the tube so that (i)
a−ae=
γ
(p−pe)
, where ae is the tube radius when the pressure, p(z,t), in the tube is equal to pe,
and
γ
is a positive constant. Assume the local volume flux, Q(z,t), is related to
∂
p
∂
z
by (ii)
Q=−
π
a48
µ
( )
∂
p
∂
z
( )
.
a) By conserving mass, find a partial differential equation that relates Q and a.
b) Combine (i), (ii), and the result of part a) into one partial differential equation for a(z,t).
c) Determine a(z) when Qo is a constant and the flow is perfectly steady.
Solution 9.29. a) Conserve mass in a differential disk control volume perpendicular to the flow
direction that has circular inlet and outlet surfaces located at z and z + Δz with radii of a(z,t) and
a(z + Δz,t). There is no flow through the tube wall so:
∂
∂
t
ρ
dV
CV
∫−
ρ
u(r,t)
0
a(z,t)
∫2
π
rdr +
ρ
u(r,t)
0
a(z+Δz,t)
∫2
π
rdr =0
.
Noting that
Q(z,t)=u(r,t)2
π
rdr
r=0
r=R(z,t)
∫
and that the CV volume is
π
a2(z,t)Δz
allows the
equation above to be simplified to:
∂ ∂
t
( )
π
a2
( )
+Q(z+Δz,t)−Q(z,t)
( )
Δz=0
,
or taking the limit as
Δz→0
:
∂
∂
t
π
a2
( )
+
∂
Q
∂
z=0
.
b) Differentiate (i) to find:
∂
a
∂
z=
γ ∂
p
∂
z
. Put this into (ii) to get:
Q=−
π
a48
γµ
( )
∂
a
∂
z
( )
.
Insert this into the result of part a):
∂
∂
t
a2
( )
=
∂
∂
z
a4
8
γµ
∂
a
∂
z
$
%
&
‘
(
)
.
c) For steady flow, the part a) result is not needed. From part b), (i) and (ii) imply:
Qo=−
π
a48
γµ
( )
∂
a
∂
z
( )
. Integrate once to find:
π
a5
40
γµ
=−Qoz+C
where C is a constant. At z =
L, a = ae so
C=
π
ae
5
40
γµ
+QoL
. Thus the final answer is:
a(z)=40
γµ
Qo
π
(L−z)+ae
5
%
&
‘
(
)
*
1 5
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 9.30. Consider a simple model of flow from a tube of toothpaste. A liquid with
viscosity µ and density
ρ
is squeezed out of a round horizontal tube having radius a(t). In your
work, assume that a is decreasing and use cylindrical coordinates with the z-axis coincident with
the centerline of the tube. The tube is closed at z = 0, but is open to the atmosphere at z = L.
Ignore gravity.
a) If w is the fluid velocity along the z-axis, show that:
za da
dt +w(z,R,t)RdR
0
a
∫=0
.
b) Determine the pressure distribution, p(z) – p(L), by assuming the flow in the tube can be
treated within the lubrication approximation by setting
w(z,R,t)=−1
4
µ
dp
dz a2(t)−R2
( )
.
c) Find the cross-section-average flow velocity
wave (z,t)
in terms of z, a, and
da dt
.
d) If the pressure difference between z = 0 and z = L is ΔP, what is the volume flux exiting the
tube as a function of time. Does this answer partially explain why fully emptying a toothpaste
tube by squeezing it is essentially impossible?
Solution 9.30. a) Choose a CV that encloses the fluid inside the tube from the capped end to an
axial distance z. Conservation of mass implies:
d
dt
ρπ
a2z
( )
+
ρ
w(z,R,t)2
π
RdR =0
0
a
∫
or
za da
dt +w(z,R,t)RdR =0
0
a
∫
.
b) Here p = p(z) alone; insert the given velocity profile into the result of part a) and integrate:
za da
dt =1
4
µ
dp
dz a2(t)−R2
( )
RdR
0
a
∫=a4(t)
16
µ
dp
dz
.
The ends of this equality form a differential equation for the pressure; integrate from z to L:
p(z)−p(L)=−8
µ
a3
( )
da dt
( )
L2−z2
( )
.
c) Multiply the result of part a) by 2a–2, insert factors of π, and recognize the definition of wave:
2
a2w(z,R,t)RdR
0
a
∫=1
π
a2w(z,R,t)2
π
RdR
0
a
∫=wave (z,t)=−2
a2
%
&
‘ (
)
*
za da
dt =−2z
a
da
dt
,
d) Use Q(t) = πa2wave(L,t), and combine the results of parts b) and c) to eliminate da/dt. From
part b):
p(0) −p(L)=ΔP=−8
µ
a3
da
dt
$
%
& ‘
(
) L2−02
( )
, or
da
dt
“
#
$ %
&
‘ =−a3ΔP
8
µ
L2
; so
Q(t)=
π
a2wave (L,t)=−
π
a22L
a
da
dt =−2
π
La da
dt =−2
π
La −a3ΔP
8
µ
L2
%
&
‘
(
)
* =
π
4
a4ΔP
µ
L
Yes, this answer explains a lot about flow from a toothpaste tube. Here, it is clear that for a
finite ΔP (the pressure one can exert by squeezing), the volume flux from the tube will be
proportional to
a4
. Thus, as the tube empties and its effective cross section decreases, the flow
rate from the tube approaches zero. The only hope for better emptying the tube is to decrease L,
and it is an easily observed fact that more toothpaste can be extracted from a nearly-empty tube
by rolling it up from the sealed end.