Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.16. Ideal flow past a cylinder (7.33) is perturbed by adding a small vertical velocity
without changing the orientation of the doublet:
ψ
=−U
γ
x+Uy −Ua2y
x2+y2=−U
γ
rcos
θ
+U r −a2
r
&
‘
(
)
*
+
sin
θ
.
a) Show that the stagnation point locations are rs = a and
θ
s =
γ
/2, π +
γ
/2 when
γ
<< 1.
When
γ
<< 1, these two results can be expanded:
r
s
a≅1−1
4
γ
2+…
, and
tan
θ
s≅
γ
1+1+1
2
γ
2+… =
γ
2
1−1
4
γ
2+…
&
‘
( )
*
+
.
Thus, to lowest order, the two stagnation point locations are r = a, and
θ
=
γ
/2,
π
+
γ
/2.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.17. For the following flow fields (b, U, Q, and Γ are positive real constants), sketch
streamlines.
a)
ψ
=b r cos
θ
2
( )
for |
θ
| < 180°
b)
ψ
=Uy +Γ2
π
( )
ln x2+(y−b)2
( )
−ln x2+(y+b)2
( )
[ ]
c)
φ
=Q2
π
( )
ln x2+(y−2na)2
( )
n=−∞
n= +∞
∑
for |y| < a.
Solution 7.17. There are several ways to attack this problem. The most direct is to take a few
derivatives to determine the velocity components and then plot the results. However, detailed
plots are not required here (the problem merely says sketch the streamlines); thus, flow directions
can be ascertained by considering limiting values of the independent variables. In particular, for
parts b) and c), very near a point vortex or a point source the streamlines will be circular or
radial, respectively, because the 1/r–factor will cause the point vortex or point source to
dominate the local flow field. Similarly, very far from a point vortex or a point source the
streamlines will be little changed from those that would exist in the absence of the point source
c)
y
x
y
x
y
x
y = a
y = –a
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.18. Take a standard sheet of paper and cut it in half. Make a simple airfoil with one
half and a cylinder with the other half that are approximately the same size as shown.
a) If the cylinder and the airfoil are dropped from the same height at the same time with the
airfoil pointed toward the ground in its most streamlined configuration, predict which one
reaches the ground first.
b) Stand on a chair and perform this experiment. What happens? Are your results repeatable?
c) Can you explain what you observe?
Solution 7.18. a) If the airfoil remains aligned in the vertical direction, its drag is less than the
cylinder. Therefore, it should reach the ground first.
b) The airfoil that was tested for this solution manual, reached the ground first about 1 out of 10
Tape
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.19. Consider the following two-dimensional stream function composed of a uniform
horizontal stream of speed U and two vortices of equal and opposite strength in (x,y)-Cartesian
coordinates.
ψ
(x,y)=Uy +Γ2
π
( )
ln x2+(y−b)2− Γ 2
π
( )
ln x2+(y+b)2
a) Simplify this stream function for the combined limit of
b→0
and
Γ → ∞
when 2bΓ = C =
constant to find:
ψ
(x,y)=Uy 1−C2
π
U
( )
x2+y2
( )
−1
( )
b) Switch to (r,
θ
)-polar coordinates and find both components of the velocity using the
simplified stream function.
c) For the simplified stream function, determine where ur = 0.
d) Sketch the streamlines for the simplified stream function, and describe this flow.
Solution 7.19. a) The square roots may be simplified for the limit
b→0
:
x2+(y±b)2=r1+(±2yb +b2)r2≈r1±by r2
( )
where
r2=x2+y2
. Thus:
ln x2+(y±b)2≈ln(r)+ln 1±by r2
( )
≈ln(r)±by r2
, so the stream
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.20. Graphically generate the streamline pattern for a plane half-body in the following
manner. Take a source of strength qs = 200 m2/s and a uniform stream U = 10 m/s. Draw radial
streamlines from the source at equal intervals of Δ
θ
=
π
/10, with the corresponding stream
function interval : Δ
ψ
source = (qs/2
π
)Δ
θ
= 10 m2/s. Now draw streamlines of the uniform flow
with the same interval, that is, Δ
ψ
stream = UΔ
ψ
= 10 m2/s. This requires Δy = 1 m, which can be
plotted assuming a linear scale of 1 cm = 1m. Connect points of equal
ψ
=
ψ
source +
ψ
stream to
display the flow pattern.
Solution 7.20. Let subscripts “1” and “2” represent the source and the free stream, respectively.
ψ
1=qs
2
πθ
→ Δ
ψ
1=qs
2
π
Δ
θ
=qs
20 =10m2/s
, and
ψ
2=Uy → Δ
ψ
2=UΔy=10m2/s
.
With the above intervals for the stream functions, draw the streamlines for the source and the
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
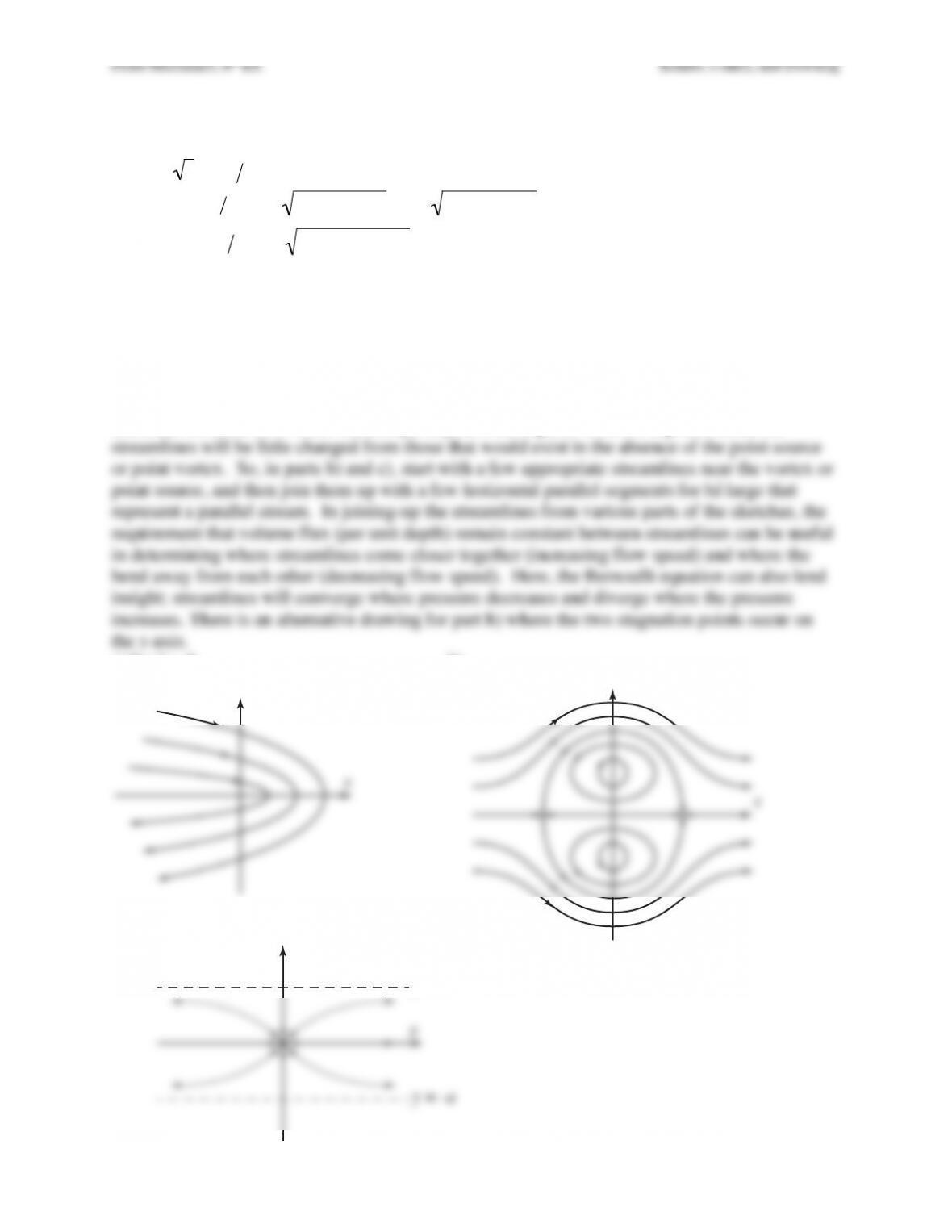
Exercise 7.21. Consider the two-dimensional steady flow formed by combining a uniform
stream of speed U in the positive x-direction, a source of strength qs > 0 at (x,y) = (–a, 0), and a
sink of strength qs at (x,y) = (+a, 0) where a > 0. The pressure far upstream of the origin is p∞.
a) Write down the velocity potential and the
stream function for this flow field.
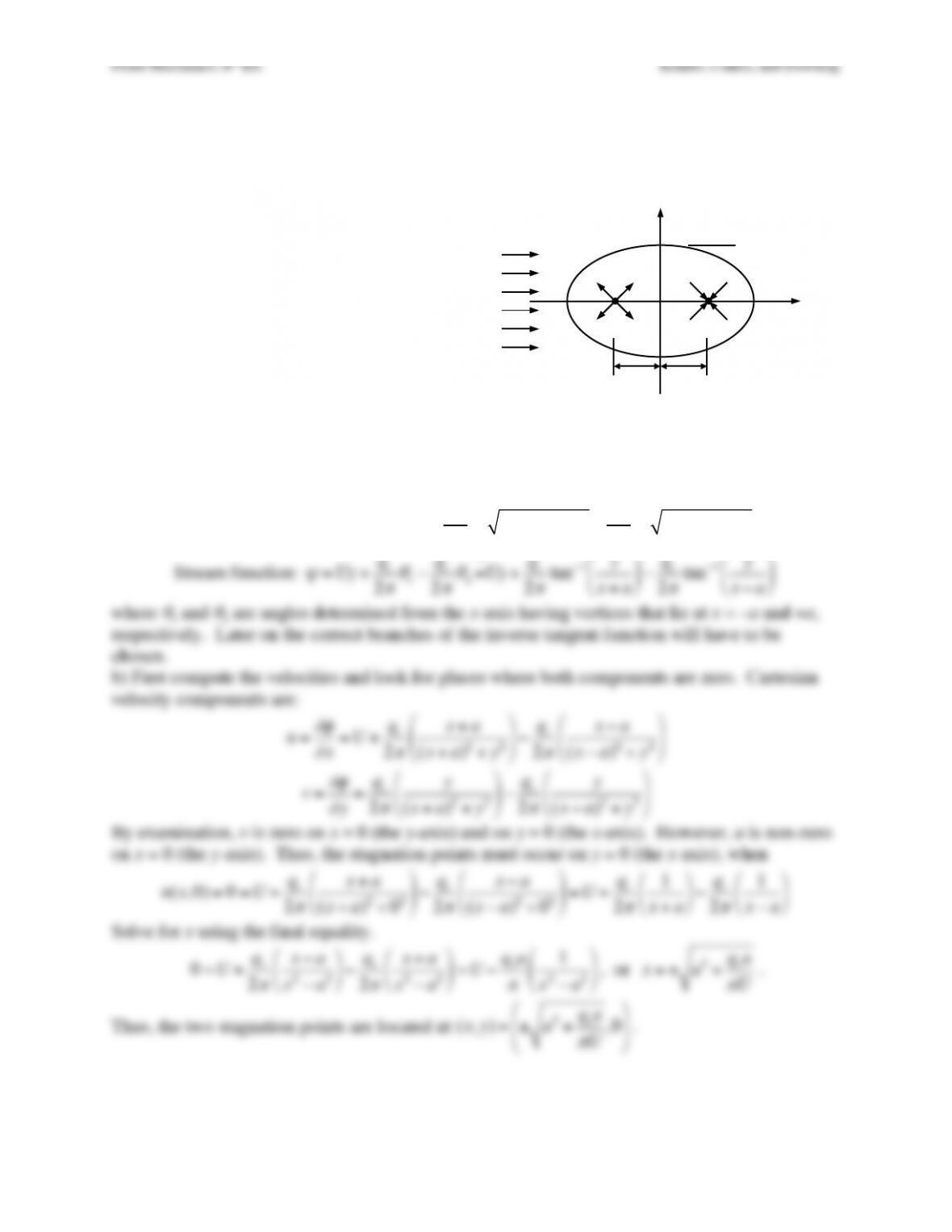
b) What are the coordinates of the stagnation
points, marked by s in the figure?
c) Determine the pressure in this flow field along
the y-axis.
d) There is a closed streamline in this flow that
defines a Rankine body. Obtain a transcendental
algebraic equation for this streamline, and show
that the half-width, h, of the body in the y–
direction is given by: h/a = cot(
π
Uh/qs). (The
introduction of angles may be useful here.)
Solution 7.21. a) For both ideal flow functions, there will three terms: the free stream, source at
x = –a, and the sink at x = +a.
Velocity potential:
φ
(x,y)=Ux +qs
2
π
ln (x+a)2+y2−qs
2
π
ln (x−a)2+y2
Stream function:
ψ
=Uy +qs
1−qs
2=
?
Uy +qs
tan−1y
“
#
$%
&
‘−qs
tan−1y
“
#
$%
&
‘
x!
y!
y = h!
a!
a!
U!
s! s!
+qs! –qs!
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
c) Using the information above,
(u,v)x=0=U+qs
π
a
a2+y2
!
“
#$
%
&, 0
!
“
#$
%
&
on the y-axis. Thus, use the
steady ideal flow Bernoulli equation,
p(x,y)+1
2
ρ
u2+v2
( )
=p∞+1
2
ρ
U2
, with the above
replacements for u and v.
p(x,y)+1
2
ρ
U+qs
π
a
a2+y2
!
“
#$
%
&
!
“
#$
%
&
2
=p∞+1
2
ρ
U2
Simplify this expression to find:
p(0, y)−p∞= + 1
2
ρ
U2−1
2
ρ
U+qs
π
a
a2+y2
#
$
%&
‘
(
#
$
%&
‘
(
2
=−
ρ
qsU
π
a
a2+y2
#
$
%&
‘
(−
ρ
qs
2
2
π
2
a
a2+y2
#
$
%&
‘
(
2
.
d) The streamline that lies on the x-axis divides to become the closed streamline. Upstream of
the source and sink, all the angles are π. Thus the stream-function constant, can be evaluated
ψ
=Ur sin
θ
+qs
2
πθ
2−qs
2
πθ
1=Ur sin
π
+qs
2
ππ
−qs
2
ππ
=0
So the equations for the shape of the Rankine body in polar and Cartesian coordinates are:
0=Ur sin
θ
+qs
2
πθ
1−
θ
2
( )
, or
0=Uy +qs
2
π
tan−1y
x+a
“
#
$%
&
‘−qs
2
π
tan−1y
x−a
“
#
$%
&
‘
,
where the branches of the inverse tangent function must be chosen correctly. To find an
equation for the half width, h, of the body, a combination of the above formulae is needed. From
the symmetry of the flow, the widest part of the Rankine body will occur at x = 0, so the body
contour will cross the y-axis at (0,±h). Consider the point (0,h). The angles associated with this
point are:
θ
=
π
2
,
θ
1=tan−1h a
( )
, and
θ
2=
π
−tan−1h a
( )
, where the usual range, –π/2 to + π/2,
of the inverse tangent has been chosen. Thus, the polar-coordinate body-shape equation
produces:
0=Uh +qs
2
π
2 tan−1h
a
“
#
$%
&
‘−
π
“
#
$%
&
‘
, or
π
Uh
qs
=
π
2−tan−1h
a
“
#
$%
&
‘
, or
Now take the cotangent of both sides of the final equation and use the trigonometric identity:
cot
π
2−z
$
%
& ‘
(
) =tan(z)
to find:
cot
π
Uh
qs
!
“
#$
%
&=cot
π
2−tan−1h
a
!
“
#$
%
&
!
“
#$
%
&=tan tan−1h
a
!
“
#$
%
&
!
“
#$
%
&=h
a
, and the two
ends of this extended equality are the desired result.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.22. A stationary ideal two-dimensional vortex with clockwise circulation Γ is located
at point (0, a), above a flat plate. The plate coincides with the x-axis. A uniform stream U
directed along the x-axis flows past the vortex.
a) Sketch the flow pattern and show that it represents the flow over an oval-shaped body when
Γ/πa > U. [Hint: Introduce the image vortex and locate the two stagnation points on the x-axis.]
b) If the pressure far from the origin just above the x-axis is p∞ show that the pressure p at any
location on the plate is:
p∞−p=
ρ
Γ2a2
2
π
2(x2+a2)2−
ρ
UΓa
π
(x2+a2)
.
c) Using the result of part b), show that the total upward force F on the plate per unit depth into
the page is F = –
ρ
UΓ +
ρ
Γ2/4
π
a when the pressure everywhere below the plate is p∞.
Solution 7.22. a) The stream function for this flow field is:
ψ
=Uy +Γ2
π
( )
ln x2+(y−a)2
( )
−ln x2+(y+a)2
( )
[ ]
,
where the image vortex is represented by the second natural log function. For y = 0 and Γ/πa > U
the flow field looks like:
b) The horizontal velocity on y = 0 is:
u(x,0) =
∂ψ
∂
y
$
%
&
‘
(
)
y=0
=U+Γ
2
π
y−a
x2+(y−a)2−Γ
2
π
y+a
x2+(y+a)2
$
%
&
‘
(
)
y=0
=U−Γ
π
a
x2+a2
.
The Bernoulli equation evaluated on y = 0 determines the pressure p on the plate:
=
π
(
π
)+
2
π
2a
2
)
* +
,
– =
4
π
a−
ρ
UΓ.
y
x
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.23. Consider plane flow around a circular cylinder. Use the complex potential and
Blasius theorem (7.60) to show that the drag is zero and the lift is L =
ρ
UΓ. (In Section 7.3, these
results were obtained by integrating the surface pressure distribution.)
Solution 7.23. The complex potential and its derivative for ideal flow past a circular cylinder
with circulation –Γ are:
w=U z +a2
z
“
#
$
%
&
‘ +iΓ
2
π
log z
, and
dw
dz =U1−a2
z2
#
$
%
&
‘
( +iΓ
2
π
z
.
Use these in Blasius’ theorem (7.60):
D−iL =1
2
i
ρ
dw
dz
$
%
& ‘
(
)
2
dz
∫=1
2
i
ρ
U1−a2
z2
$
%
&
‘
(
) +iΓ
2
π
z
$
%
&
‘
(
)
2
dz
∫
=1
2
i
ρ
U21−a2
z2
$
%
&
‘
(
)
2
+iΓ
π
z
U1−a2
z2
$
%
&
‘
(
) −Γ2
4
π
2z2
$
%
&
&
‘
(
)
)
2
dz
∫=1
2
i
ρ
iΓ
π
z
Udz
∫
=1
2
i
ρ
iΓ
π
U(2
π
i)=−i
ρ
UΓ.
where the last two equalities follow from the residue theorem. Thus, equating real and imaginary
parts produces:
D = 0, and L =
ρ
UΓ.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.24. For the doublet flow described by (7.29) and sketched in Figure 7.6, show u < 0
for y < x and u > 0 for y > x. Also, show that v < 0 in the first quadrant and v > 0 in the second
quadrant.
Solution 7.24. Start from (7.30)
φ
=d
2
π
cos
θ
r=d
2
π
x
x2+y2
.
The velocity field is obtained by direct differentiation.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.25. Hurricane winds blow over a Quonset hut, that is, a long half-circular cylindrical
cross-section building, 6 m in diameter. If the velocity far upstream is U∞ = 40 m/s and p∞ =
1.003 × 105 N/m,
ρ
∞ = 1.23 kg/m3, find the force per unit depth on the building, assuming the
pressure inside the hut is a) p∞, and b) stagnation pressure,
p∞+1
2
ρ
∞U∞
2
.
Solution 7.25. If the flow over the hut is ideal, it can be modeled using the potential for flow past
a cylinder without circulation, and compute the velocity components:
φ
=U r +a2
r
#
$
%
&
‘
(
cos
θ
, and
ur=
∂φ
∂
r=U1−a2
r2
%
&
‘
(
)
*
cos
θ
&
u
θ
=1
r
∂φ
∂θ
=−U1+a2
r2
&
‘
(
)
*
+
sin
θ
.
The ideal flow pressure ps on the surface of the hut (r = a) can be determined from the steady
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.26. In a two-dimensional ideal flow, a source of strength qs is located a meters above
an infinite plane. Find the fluid velocity on the plane, the pressure on the plane, and the reaction
force on the plane assuming constant pressure p∞ below the plane.
Solution 7.26. The potential function for this flow field is:
φ
=qs2
π
( )
ln x2+(y−a)2
( )
+ln x2+(y+a)2
( )
“
#
$%
&
‘
,
The velocity components are:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.27. Consider a two-dimensional ideal
flow over a circular cylinder of radius r = a with
axis coincident with a right angle corner, as shown
in the figure. Assuming that
ψ
= Axy (with A =
constant) when the cylinder is absent, solve for the
stream function and velocity components.
Solution 7.27. When the cylinder is present, it will
only effect the flow near the origin, so the stream
function far from the origin will be
ψ
o = Axy. And,
the field equation for the stream function,
∇2
ψ
=0
, is linear, so a solution in the form of a simple
superposition can be sought:
ψ
(x,y)=
ψ
o+
ψ
1=Axy +
ψ
1=Ar2sin
θ
cos
θ
+
ψ
1(r,
θ
)=A2
( )
r2sin(2
θ
)+R(r)Θ(
θ
)
,
where
ψ
1 is the modification of the stream function necessary near the origin, x = rcos
θ
, y =
rsin
θ
, and the further assumption that
ψ
1 can be found via separation of variables in polar
coordinates has been made. Placing this two term trial solution into the field equation produces:
0=1
r
∂
∂
r
r
∂ψ
1
∂
r
$
%
& ‘
(
) +1
r2
∂
2
ψ
1
∂θ
2
, (&)
and the boundary condition on
ψ
1 is determined from:
1
r
∂ψ
∂θ
%
&
‘
(
)
*
r=a
=1
r
∂ψ
o
∂θ
+1
r
∂ψ
1
∂θ
%
&
‘
(
)
*
r=a
=ur(a,
θ
)=0
.
For
ψ
o=A2
( )
r2sin(2
θ
)
, this implies:
Aacos(2
θ
)+1
a
∂ψ
1
∂θ
%
&
‘
(
)
*
r=a
=0
, or
∂ψ
1
∂θ
%
&
‘
(
)
*
r=a
=−Aa2cos(2
θ
)→
ψ
1(a,
θ
)=−Aa2
2sin(2
θ
)
.
Thus, the form Θ(
θ
) = sin(2
θ
) has been determined so that
ψ
1=R(r)sin(2
θ
)
, and the boundary
condition implies
R(a)=−Aa22
. Placing the trial solution for
ψ
1 into (&) produces:
0=1
r
d
dr
rdR
dr
“
#
$ %
&
‘ −4
r2R
)
*
+
,
–
.
sin(2
θ
)
,
where the partial derivatives have been changed total derivatives because R(r) only depends on
the variable r. This equation is equi-dimensional and therefore has power law solutions of the
form R = Brm. Substituting in this solution form leads to the algebraic equation:
m2rm−2−4rm−2=0
,
which implies m = ±2. The positive root reproduces
ψ
o, so the negative root should be chosen
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.28. Consider the following two-dimensional velocity potential consisting of two
sources and one sink, all of strength qs:
<Begin Equation>
φ
(x,y)=qs2
π
( )
ln (x−b)2+y2+ln (x−a2b)2+y2−ln x2+y2
( )
.
</End Equation>
Here a and b are positive constants and b > a.
a) Determine the locations of the two stagnation points in this flow field.
b) Sketch the streamlines in this flow field.
c) Show that the closed streamline in this flow is given by x2 + y2 = a2.
Solution 7.28. a) Here
φ
only depends on y2 when x = 0. Thus,
v=
∂φ ∂
y=0
on y = 0, the x–
axis, so finding the stagnation points means, finding the x-axis locations where
u=
∂φ ∂
x=0
;
∂φ
∂
x
!
“
#$
%
&
y=0
=qs
2
π
x−b
(x−b)2+y2+x−a2b
(x−a2b)2+y2−x
x2+y2
!
“
#$
%
&
y=0
=qs
2
π
1
x−b+1
x−a2b−1
x
(
)
*+
,
–=0
Working with the final equality produces:
x(x−a2b)+x(x−b)−(x−b)(x−a2b)=0
, which
simplifies to:
x2−a2=0
. Thus, the
two stagnation points are at (±a,0).
b) The two sources and the sink all
lie on the x–axis with the sink at the
origin. The stagnation points lie
between the two sources at x = +a
and to left of the origin at x = –a.
A closed body is obtained, and it is
shown in part c) that it is circular.
To allow labeling, the streamlines
are as shown for y ≥ 0 only. They
are symmetric about y = 0.
c) First convert everything to the
usual polar coordinates, and define:
r
1≡(x−b)2+y2=(rcos
θ
−b)2+r2sin2
θ
=r2−2brcos
θ
+b2
, and
r2≡(x−a2b)2+y2=(rcos
θ
−a2b)2+r2sin2
θ
=r2−2(a2b)rcos
θ
+(a2b)2
.
Thus, the potential can be written:
x
y
–a
+a
+b
+a2/b
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.29. Without using complex variables, derive the results of the Kutta–Zhukhovsky lift
theorem (7.62) for steady two-dimensional
irrotational constant-density flow past an
arbitrary-cross-section object by considering the
clam-shell control volume (shown as a dashed
line) in the limit as
r→ ∞
. Here A1 is a large
circular contour, A2 follows the object’s cross
section contour, and A3 connects A1 and A2. Let
p∞ and Uex be the pressure and flow velocity far
from the origin of coordinates, and denote the
flow extent perpendicular to the x–y plane by B.
Solution 7.29. In ideal flow, the only surface forces are pressure forces. Therefore, the drag (D)
and lift (L) forces on the body are defined by:
Dex+Ley=−p−p∞
( )
n2dA
A2
∫=p−p∞
( )
ndA
A2
∫
, (a)
where the free stream velocity is U = Uex, and n is the outward normal on the total control
volume; thus it points into the body on surface A2 so the usual minus sign is missing from the
final equality in (a) because n = –n2. Note that the constant p∞ can be included in the pressure
integration because
p∞ndA
closed surface
∫=0
.
The starting point for the derivation is the integral form of the steady ideal flow
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
where the limit
r→ ∞
is now explicitly written. Collect terms under the integrand according to
their power of r:
D
B=−lim
r→∞
ρ
U2rcos
θ
−
ρ
ΓU
2
π
sin
θ
cos
θ
+
ρ
ΓU
2
π
sin
θ
cos
θ
−
ρ
Γ2cos
θ
8
π
2r−
ρ
Ud cos
θ
2
π
r+…
)
*
+
,
–
.
0
2
π
∫d
θ
.
The first integrand term makes no contribution because the integral of the cosine over one period
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.30. Pressure fluctuations in wall-bounded turbulent flows are a common source of
flow noise. Such fluctuations are caused by turbulent eddies as they move over the bounding
surface. A simple ideal-flow model that captures some of the important phenomena involves a
two-dimensional vortex that moves above a flat surface in a fluid of density
ρ
. Thus, for the
following items, use the potential:
φ
(x,y,t)=−Γ
2
π
tan−1y−h
x−Ut
&
‘
( )
*
+ +Γ
2
π
tan−1y+h
x−Ut
&
‘
( )
*
+
where h is the distance of the vortex above the flat surface, Γ is the vortex strength, and U is the
convection speed of the vortex.
a) Compute the horizontal u and vertical v velocity components and verify that v = 0 on y = 0.
b) Determine the pressure at x = y = 0 in terms of
ρ
, t, Γ, h, and U.
c) Based on your results from part b), is it possible for a fast-moving high-strength vortex far
from the surface to have the same pressure signature as a slow-moving low-strength vortex
closer to the surface?
Solution 7.30. a) Start with the given potential,
φ
(x,y,t)=−Γ
2
π
tan−1y−h
x−Ut
&
‘
( )
*
+ +Γ
2
π
tan−1y+h
x−Ut
&
‘
( )
*
+
,
and differentiate to find:
u=
∂φ
∂
x=−Γ
2
π
1+(y−h)2
(x−Ut)2
‘
(
)
*
+
,
−1
−y−h
(x−Ut)2
‘
(
) *
+
, +Γ
2
π
1+(y+h)2
(x−Ut)2
‘
(
)
*
+
,
−1
−y+h
(x−Ut)2
‘
(
) *
+
,
=Γ
2
π
y−h
(x−Ut)2+(y−h)2−y+h
(x−Ut)2+(y+h)2
%
&
(
)
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling