Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
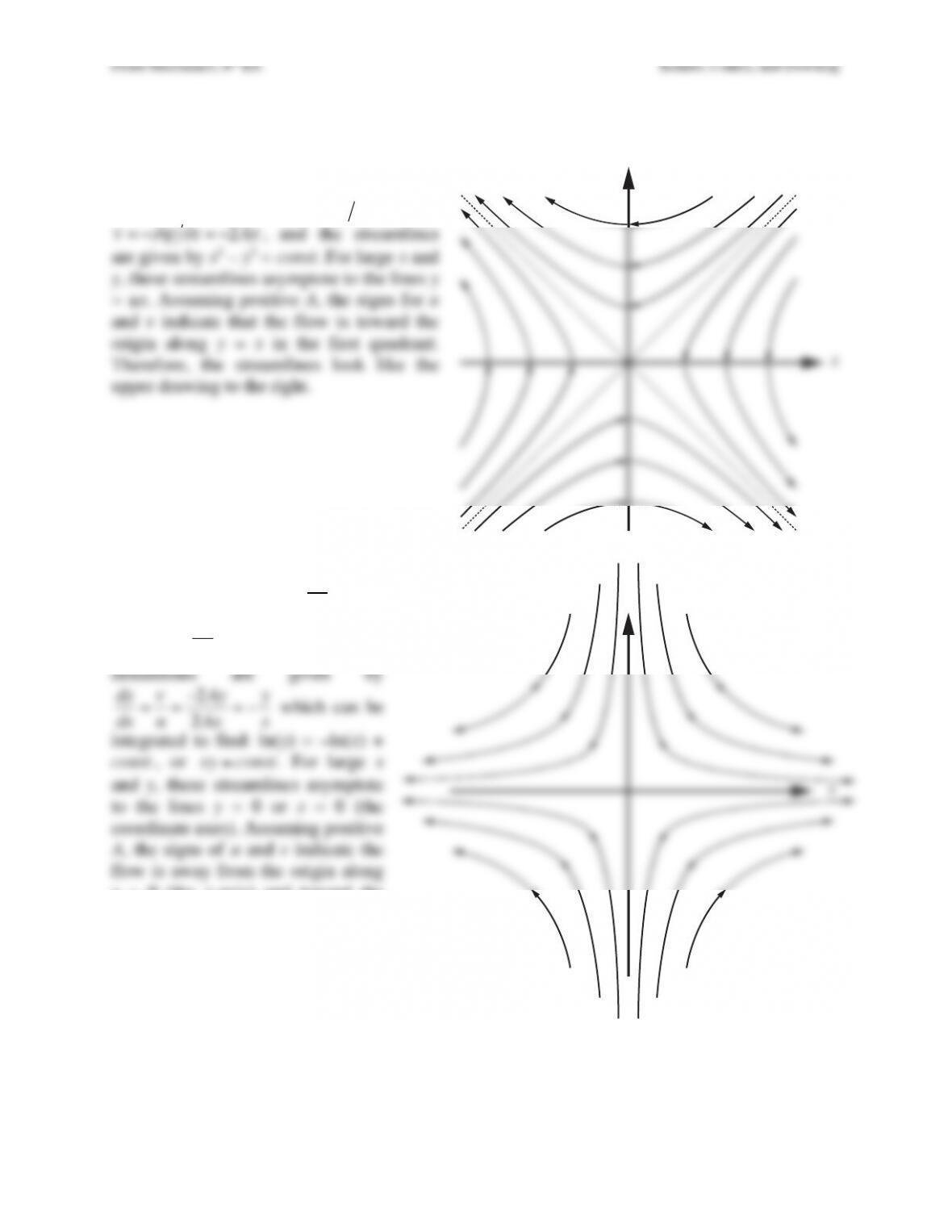
Exercise 7.1. a) Show that (7.7) solves (7.5) and leads to u = (U,V).
b) Integrate (7.6) within circular area centered on (x´, y´) of radius
“
r =(x−“
x )2+(y−“
y )2
to
show that (7.8) is a solution of (7.6).
Solution 7.1. a) Start with
ψ
=−Vx +Uy
. Insert this into the Laplace equation:
∇2
ψ
=
∂
2
∂
x2+
∂
2
∂
y2
%
&
‘
(
)
* −Vx +Uy
( )
=0
.
The final equality follows because
ψ
is just a linear function so both of the indicated second
derivatives are zero. The velocity field is found via differentiation.
u=
∂ψ
∂
y,−
∂ψ
∂
x
%
&
‘
(
)
* =
∂
(−Vx +Uy)
∂
y,−
∂
(−Vx +Uy)
∂
x
%
&
‘
(
)
* =U,V
( )
.
b) First apply a simple shift transformation that places x´ = (x´, y´) at the origin of coordinates.
Define these new coordinates by:
X=x−#
x
,
Y=y−#
y
and set
“
r =x−“
x =X2+Y2
. The
gradient operator
∇XY
in the shifted coordinates X = (X, Y) is the same as
∇
in the unshifted
coordinates (x, y), so the (7.6) becomes:
∇XY
2
ψ
=−Γ
δ
(x−‘
x )
δ
(y−‘
y )=−Γ
δ
(X)
δ
(Y)
.
Integrate this equation inside a circle of radius r´:
∇XY
2
ψ
circular area
∫∫ dA =∇XY
ψ
⋅n
circle
∫d=−Γ
δ
(X)
δ
(Y)
x=−)
r 2−y2
x= + )
r 2−y2
∫
y=−)
r
y= + )
r
∫dXdY
,
where the first equality follows from Gauss’ divergence theorem in two dimensions, and the
double integral on the right side includes the location X = 0 (aka x = x´) so a contribution is
collected from both delta functions. Thus, the double integral on the right side of this equation is
unity.
The dot product in the middle portion of the above equation simplifies to ∂
ψ
/∂r´ because
n = er on the circle and
er⋅ ∇XY =
∂ ∂
%
r
. Plus, in an unbounded uniform environment, there are
no preferred directions so
ψ
=
ψ
(r´) alone (no angular dependence). Thus, the integrated field
equation simplifies to
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.2. For two-dimensional ideal flow, show separately that: a)
∇
ψ
⋅ ∇
φ
=0
, b)
−∇
ψ
× ∇
φ
=u2ez
, c)
∇
ψ
2=∇
φ
2
, and d)
∇
φ
=−ez× ∇
ψ
.
Solution 7.2. In terms of components, the velocity u = (u, v) = (∂
ψ
/∂y, –∂
ψ
/∂x) = (∂
φ
/∂x, ∂
φ
/∂y),
and these equations can be used to complete the various parts of this problem.
a)
∇
ψ
⋅ ∇
φ
=
∂ψ
∂
x,
∂ψ
∂
y
‘
)
*
,
⋅
∂φ
∂
x,
∂φ
∂
y
‘
)
*
, =−v,u
( )
⋅u,v
( )
=−vu +uv =0
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.3. a) Show that (7.14) solves (7.12) and leads to u = (U,V).
b) Integrate (7.13) within circular area centered on (x´, y´) of radius
!
r=(x−!
x)2+(y−!
y)2
to show that (7.15) is a solution of (7.13).
c) For the flow described by (7.15), show that the volume flux (per unit depth into the page)
=u⋅n
C
∫ds
computed from a closed contour C that encircles the point (x´, y´) is qs. Here n is
the outward normal on C and ds is a differential element of C.
Solution 7.3. a) Start with
φ
=Ux +Vy
. Insert this into the Laplace equation:
∇2
φ
=
∂
2
∂
x2+
∂
2
∂
y2
%
&
‘
(
)
* Ux +Vy
( )
=0
The final equality follows because
φ
is a linear function so both of the indicated second
derivatives are zero. The velocity field is found via differentiation.
u=
∂φ
∂
x,
∂φ
∂
y
$
%
&
‘
(
) =
∂
(Ux +Vy)
∂
x,
∂
(Ux +Vy)
∂
y
$
%
&
‘
(
) =U,V
( )
.
b) First apply a simple shift transformation that places x´ = (x´, y´) at the origin of coordinates.
Define these new coordinates by:
X=x−#
x
,
Y=y−#
y
and set
“
r =x−“
x =X2+Y2
. The
gradient operator
∇XY
in the shifted coordinates X = (X, Y) is the same as
∇
in the unshifted
coordinates (x, y), so the (7.13) becomes:
∇XY
2
φ
=qs
δ
(x−#
x)
δ
(y−#
y)=qs
δ
(X)
δ
(Y)
.
Integrate this equation inside a circle of radius r´:
∇XY
2
φ
circular area
∫∫ dA =∇XY
φ
⋅n
circle
∫d=m
δ
(X)
δ
(Y)
x=−(
r 2−y2
x= + (
r 2−y2
∫
y=−(
r
y= + (
r
∫dXdY
,
where the first equality follows from Gauss’ divergence theorem in two dimensions, and the
double integral on the right side includes the location X = 0 (aka x = x´) so a contribution is
collected from both delta functions. Thus, the right-side double integration is unity.
The dot product in the middle portion of the above equation simplifies to ∂
φ
/∂r´ because
n = er´ on the circle and
e“
r ⋅ ∇XY =
∂ ∂
“
r
. Plus, in a unbounded uniform environment, there are
no preferred directions so
φ
=
φ
(r´) alone (no angular dependence). Thus, the integrated field
equation simplifies to
∂φ
∂
!
r
“
#
$%
&
‘
θ
=0
2
π
∫!
r d
θ
=2
π
!
r
∂φ
∂
!
r=qs
, which implies
∂φ
∂
!
r=qs
2
π
!
r
or
φ
=qs
2
π
ln( !
r)=qs
2
π
ln (x−!
x)2+(y−!
y)2
,
and this is (7.15). Thus, (7.15) is a solution of (7.13). Here, the constant from the final
integration has been suppressed (set equal to zero) because it has no impact on the velocity field,
which depends on derivatives of
φ
.
c) For the flux integration, evaluate the dot product of u and n = er´:
u⋅n=∇
φ
⋅e%
r =
∂φ ∂
%
r =u%
r
,
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.4. Show that (7.1) reduces to
∂φ
∂
t+1
2∇
φ
2+p
ρ
=const.
when the flow is described by
the velocity potential
φ
.
Solution 7.4. Start from (7.1),
∂
u
∂
t+u⋅ ∇
( )
u+1
ρ
( )
∇p=0
, and use the vector identity (B3.9),
u⋅ ∇
( )
u=∇1
2u2
( )
−u× ∇ × u
( )
to replace the advective acceleration with a gradient and cross
product term:
Now define a mildly revised potential that includes B(t):
φ
=#
φ
+B(
τ
)−const.
( )
d
τ
0
t
∫
, so that
∂φ
∂
t=
∂
$
φ
∂
t+B(t)−const.
This does not change the velocity field because
∇#
φ
=∇
φ
=u
. Therefore, the remnant of (7.1)
becomes:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.5. Consider the following two-dimensional Cartesian flow fields: (i) solid body
rotation (SBR) at angular rate Ω about the origin: (u, v) = (–Ωy, Ωx); and (ii) uniform expansion
(UE) at linear expansion rate Θ: (u, v) = (Θx, Θy). Here Ω, Θ, and the fluid density
ρ
are positive
real constants and there is no body force.
a) What is the stream function
ψ
SBR(x,y) for solid body rotation?
b) Is there a potential function
φ
SBR(x,y) for solid body rotation? Specify it if it exists.
c) What is the pressure, pSBR(x,y), in the solid body rotation flow when pSBR(0,0) = po?
d) What is the potential function
φ
UE(x,y) for uniform expansion?
e) Is there a stream function
ψ
UE(x,y) for uniform expansion? Specify it if it exists.
f) Determine the pressure, pUE(x,y), in the uniform expansion flow when pUE(0,0) = po.
Solution 7.5. a) Start with the stream-function-based velocity definitions,
u=∂
ψ
∂y=−Ωy
and
v=−∂
ψ
∂x=Ωx
,
and integrate each second equality once to find:
ψ
=−(Ω2)y2+f1(x)
and
ψ
=−(Ω2)x2+f2(y)
.
The two equations are consistent when:
ψ
=−(Ω2)(x2+y2)+const.
, and this result makes sense;
and integrate to find:
pSBR (x,y)=
ρ
Ω2x22
( )
+f(y)
and
pSBR (x,y)=
ρ
Ω2y22
( )
+g(x)
, where
f and g are functions of integration. Combining these equations into one by appropriately
choosing f and g, and then applying the boundary condition on x = y = 0 to determine the final
constant, yields:
pSBR (x,y)=po+
ρ
Ω2
2x2+y2
( )
, or in cylindrical coordinates
p(R)=po+
ρ
Ω3
2
2R2
.
This answer makes sense because the necessary centripetal acceleration of the rotating fluid must
be provided by pressure forces. So, increasing pressure with increasing R is physically
meaningful, even when viewed from a rotating dynamics point of view. This result can be
checked with the swirling flow of a liquid in a cylindrical container (see Section 5.1). The height
of the free surface is proportional to the pressure in the fluid, and the free surface of a liquid in
solid body rotation is parabolic. This effect was utilized by astronomers to cast telescope mirror
blanks on rotating tables in the early part of the 1900’s. The radial pressure gradient found
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
above is also used to separate constituents in fluid mixtures in a centrifuge, a technique widely
employed in the production of weapons-grade uranium through the separation of isotopes of UF6
(a gas), and in the biological sciences to obtain nucleic material from cells.
d) Start with the potential-function-based velocity definitions,
u=∂
φ
∂x=Θx
and
v=∂
φ
∂y=Θy
,
and integrate each equation once to find:
φ
=(Θ2)x2+f3(y)
and
φ
=(Θ2)y2+f4(x)
. The two
equations are consistent when:
φ
=(Θ2)(x2+y2)+const.
e) There is no stream function for UE flow because it is everywhere not mass conserving in
constant density flow.
f) Use the momentum equation as in part c) or the Bernoulli equation between the origin and the
point (x,y):
pUE (x,y)+1
2
ρ
u2+v2
( )
=po+0
, or
pUE (x,y)=po−1
2
ρ
Θ2x2+y2
( )
.
This answer is, of course, unusual since the flow is everywhere non-mass-conserving.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.6. Determine u and v, and sketch streamlines for a)
ψ
= A(x2 – y2), and b)
φ
= A(x2 –
y2).
Solution 7.6. a) From the definition of the
stream function:
u=
∂ψ ∂
y=−2Ay
,
v=−
∂ψ ∂
x=−2Ax
, and the streamlines
are given by x2 – y2 = const. For large x and
y, these streamlines asymptote to the lines y
= ±x. Assuming positive A, the signs for u
and v indicate that the flow is toward the
origin along y = x in the first quadrant.
Therefore, the streamlines look like the
upper drawing to the right.
b) From the definition of the
potential function:
u=
∂φ
∂
x=2Ax
,
and
v=
∂φ
∂
y=−2Ay
. Here the
streamlines are given by
origin along x = 0 (the y-axis).
Therefore, the streamlines look like
the lower drawing to the right.
x
y
y
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.7. For the following two-dimensional stream and potential functions, find the fluid
velocity u = (u,v), and determine why these are or are not ideal flows.
a)
ψ
= A(x2 + y2)
b)
φ
= A(x2 + y2)
Solution 7.7. a) From the definition of the stream function:
u=
∂ψ ∂
y=2Ay
,
v=−
∂ψ ∂
x=−2Ax
, so ∂u/∂x + ∂v/∂y = 0 and the flow conserves mass. Here, the streamlines are
given by x2 + y2 = const, which are circles. However,
∇2
ψ
=4A=const.
, so (7.4) implies that
ω
z
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.8. Assume
ψ
= ax3 + bx2y + cxy2 + dy3 where a, b, c, and d are constants; and
determine two independent solutions to the Laplace equation. Sketch the streamlines for both
flow fields.
Solution 7.8. Determine the implications of
∇2
ψ
=0
by evaluating derivatives:
∂
2
ψ
∂
x2=6ax +2by
, and
∂
2
ψ
∂
y2=2cx +6dy
, so
∂
2
ψ
∂
x2+
∂
2
ψ
∂
y2=0=6ax +2by +2cx +6dy
.
The first non-trivial solution
ψ
1 occurs
when 6a + 2c = 0 or c = –3a, and b = d = 0:
ψ
1=a x3−3xy2
( )
=ax x +3y
( )
x– 3y
( )
.
Thus, the streamlines corresponding to
ψ
1 = 0 are
three lines specified by x = 0 and
y= ± x3
. The
velocity components determined from
ψ
1 are:
u=
∂ψ
1
∂
y=−6axy
, and
v=−
∂ψ
1
∂
x=−a(3x2−3y2)=−3a(x+y)(x−y)
.
Assuming positive a, the streamlines for this flow
field appear in the upper figure to the right.
The second non-trivial solution
ψ
2
occurs when a = c = 0, and 2b + 6d = 0 or d = –
3d:
ψ
2=d−3x2y+y3
( )
=dy y +3x
( )
y– 3x
( )
.
Thus, the streamlines corresponding to
ψ
2 = 0
are three lines specified by y = 0 and
y= ±x3
.
The velocity components determined from
ψ
2
are:
u=
∂ψ
2
∂
y=−3dx2+3dy2=−3d(x+y)(x−y)
,
and
v=−
∂ψ
2
∂
x=−d(−6xy)=6dxy
.
Assuming positive d, the streamlines for this
flow field appear in the lower figure to the
right.
The two flow fields are identical when a = d
and the streamlines are rotated by 30°.
x
y
x
y
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.9. Repeat exercise 7.8. for
ψ
= ax4 + bx3y + cx2y2 + dxy3 + ey4 where a, b, c, d, and e
are constants.
Solution 7.9. Determine the implications of
∇2
ψ
=0
by evaluating derivatives:
∂
2
ψ
∂
x2=12ax2+6bxy +2cy2
, and
∂
2
ψ
∂
y2=2cx2+6dxy +12ey2
, so
∂
2
ψ
∂
x2+
∂
2
ψ
∂
y2=0=12ax2+6bxy +2c(y2+x2)+6dxy +12ey2
.
Therefore, non-trivial solutions occur when 12a + 2c = 0 or c = –6a, 6b + 6d = 0 or b = –d, and
2c + 12e = 0 or c = –6e. Choosing a and then b as the free parameter, leads to:
ψ
1=a(x4−6x2y2+y4)=a(x2−y2)2−4x2y2
( )
=a x2−y2+2xy
( )
x2−y2−2xy
( )
=a(x+y)2−2y2
( )
(x−y)2−2y2
( )
=a x +(1+2)y
( )
x+(1−2)y
( )
x−(1−2)y
( )
x−(1+2)y
( )
,
and
ψ
2=b(x3y−xy3)=bxy(x+y)(x−y)
.
Consider each solution in turn.
The streamlines corresponding to
ψ
1 =
0 are four lines specified all possible sign
combinations of
y= ± x1±2
( )
. The
velocity components determined from
ψ
1 are:
u=
∂ψ
1
∂
y=−12ax 2y+3y3
, and
v=−
∂ψ
1
∂
x=−a(4 x3−12xy 2)
.
Assuming positive a, the streamlines for this
flow field appear in the upper figure to the
right.
The streamlines corresponding to
ψ
2 = 0 are four lines specified by x = 0, y
to the left.
x
y
y
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.10. Without using complex variables, determine:
a) The potential
φ
for an ideal vortex of strength Γ starting from (7.8)
b) The stream function for an ideal point source of strength qs starting from (7.15)
c) Is there any ambiguity in your answers to parts a) and b)? If so, does this ambiguity influence
the fluid velocity?
Solution 7.10. a) The starting point is the stream function for a two-dimensional point vortex of
strength Γ located at x = x´ = (x´, y´):
ψ
=−Γ
2
π
ln (x−&
x )2+(y−&
y )2
.
The velocity components are:
u=
∂ψ
∂
y=−Γ
2
π
y−‘
y
(x−‘
x )2+(y−‘
y )2=
∂φ
∂
x
, and
v=−
∂ψ
∂
x=Γ
2
π
x−‘
x
(x−‘
x )2+(y−‘
y )2=
∂φ
∂
y
,
where the final equality in each case comes from the definition of the potential function.
Integrate to find
φ
(x, y) using these final equalities to find
φ
(x,y)=−Γ
2
π
y−&
y
(x−&
x )2+(y−&
y )2dx +f(y)
∫
, and
φ
(x,y)=Γ
2
π
x−&
x
(x−&
x )2+(y−&
y )2dy +g(x)
∫
,
where f(y) and g(x) are single-variable functions that appear because of the integrations.
Rearrange the first integral, and create the integration variable tan
θ
= (y – y´)/(x – x´), noting that
sec2
θ
d
θ
= –[(y – y´)/(x – x´)2]dx.
φ
(x,y)=−Γ
2
π
(y−&
y ) (x−&
x )2
1+(y−&
y ) (x−&
x )
[ ]
2dx +f(y)
∫=Γ
2
π
sec2
θ
d
θ
1+tan2
θ
∫+f(y)=Γ
2
πθ
+f(y)
.
Use the same integration variable in the second integral to find:
φ
(x,y)=Γ
2
π
sec2
θ
d
θ
1+tan2
θ
∫+g(x)=Γ
2
πθ
+g(x)
.
The only way for the two results to be consistent is for f(y) = g(x) = const. Thus,
φ
(x,y)=Γ
2
π
tan−1y−&
y
x−&
x
‘
(
) *
+
, +const.
b) For this part, the starting point is the potential for a two-dimensional point source of strength
qs located at x = x´ = (x´, y´):
φ
=qs
2
π
ln (x−“
x)2+(y−“
y)2
.
The velocity components are:
u=
∂φ
∂
x=qs
2
π
x−“
x
(x−“
x)2+(y−“
y)2=
∂ψ
∂
y
, and
v=
∂φ
∂
y=qs
2
π
y−“
y
(x−“
x)2+(y−“
y)2=−
∂ψ
∂
x
,
where the final equality in each case comes from the definition of the stream function. Integrate
to find
ψ
(x, y) using these final equalities to find
ψ
(x,y)=qs
2
π
x−“
x
(x−“
x)2+(y−“
y)2dy +f(x)
∫
, and
ψ
(x,y)=−qs
2
π
y−“
y
(x−“
x)2+(y−“
y)2dx +g(y)
∫
,
where f(x) and g(y) are single-variable functions that appear because of the integrations. These
integrations are the same as in part a); thus using the same integration variable produces:
ψ
(x,y)=qs
2
πθ
+f(x)=qs
2
πθ
+g(y)
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.11. Determine the stream function of a doublet starting from (7.29) and show that the
streamlines are circles having centers on the y-axis that are tangent to x-axis at the origin.
Solution 7.11. The potential for a doublet is:
φ
=d
2
π
cos
θ
r=d
2
π
x
x2+y2
,
so the velocity field is:
u=
∂φ
∂
x=d
2
π
∂
∂
x
x
x2+y2
%
&
‘
(
)
* =d
2
π
1
x2+y2−x
(x2+y2)2(2x)
%
&
‘
(
)
* =d
2
π
y2−x2
(x2+y2)2
%
&
‘
(
)
* =
∂ψ
∂
y
, and
v=
∂φ
∂
y=d
2
π
∂
∂
y
x
x2+y2
%
&
‘
(
)
* =d
2
π
−x
(x2+y2)2(2y)
%
&
‘
(
)
* =d
2
π
−2xy
(x2+y2)2
%
&
‘
(
)
* =−
∂ψ
∂
x
,
where the final equalities for each component follow from the definition of the stream function.
Integrate the v-equation using x2 + y2 =
β
and 2xdx = d
β
to find:
ψ
(x,y)=d
2
π
2xy
(x2+y2)2dx
∫+f(y)=d
2
π
yd
β
β
2
∫+f(y)=−d
2
π
y
β
+f(y)=−d
2
π
y
x2+y2+f(y)
.
When this result is partial-differentiated with respect y, the result is:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.12.
Consider steady horizontal flow at speed U past a stationary source of strength qs located at the
origin of coordinates in two dimensions, (7.30) or (7.31). To hold it in place, an external force
per unit depth into the page, F, is applied to the source.
a) Develop a dimensionless scaling law for F = |F|.
b) Use a cylindrical control volume centered on the source with radius R and having depth B into
the page, the steady ideal-flow momentum conservation equation for a control volume,
<Begin Equation>
ρ
u u ⋅n
( )
A*
∫dA =−pndA
A*
∫+F
,
</End Equation>
and an appropriate Bernoulli equation to determine the magnitude and direction of F without
using Blasius Theorem.
c) Is the direction of F unusual in anyway? Explain it physically.
Solution 7.12. a) There are only 4 parameters: F, qs, U, and
ρ
, and these span all three
dimensions. Thus, there is only one dimensionless parameter, F/
ρ
Uqs, so it must be constant.
This implies F = (const.)
ρ
Uqs, so rest of this problem merely involves determining the constant
and the direction of F.
b) It is convenient to use x–y and r–
θ
velocities expressed in polar coordinates. Using the
potential
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.13. Repeat all three parts of Exercise 7.12 for steady ideal flow past a stationary
irrotational vortex located at the origin when the control volume is centered on the vortex. The
stream function for this flow is:
ψ
=Ur sin
θ
− Γ 2
π
( )
ln(r)
Solution 7.13. a) There are only 4 parameters: F, Γ, U, and
ρ
, and these span all three
dimensions. Thus, there is only one dimensionless parameter, F/
ρ
UΓ, so it must be constant.
This implies F = (const.)
ρ
UΓ, so rest of this problem merely involves determining the constant
and the direction of F.
b) For this problem, it is convenient to use a mixture of x–y and r–
θ
velocities expressed in polar
coordinates. The potential for this flow field is
φ
=Ur cos
θ
+Γ
θ
2
π
. Performing the requisite
differentiations produces:
u=(u,v)=U−Γ
2
π
rsin
θ
,Γ
2
π
rcos
θ
&
‘
( )
*
+
, and
u=(ur,u
θ
)=Ucos
θ
,−Usin
θ
+Γ
2
π
r
&
‘
( )
*
+
Choose the control volume to be a cylinder of radius R centered on the point vortex and having
depth B into the page. The equation for conservation of mass is identically satisfied for all
potential flows without sources.
To find the force from the given CV momentum equation, first identify unit vectors and
evaluate dot products. Here,
n=er
so that
u⋅n=ur
. Therefore:
F=
ρ
(uex+vey)urBRd
θ
θ
=0
θ
=2
π
∫+p(excos
θ
+eysin
θ
)BRd
θ
θ
=0
θ
=2
π
∫
The pressure at r can be evaluated via a Bernoulli equation:
p∞+1
2
ρ
U2=p+1
2
ρ
u2+v2
( )
=p+1
2
ρ
U2−2UΓsin
θ
2
π
r+Γ2
4
π
2r2
(
)
*
+
,
–
, so
p=p∞+
ρ
UΓsin
θ
2
π
r−
ρ
Γ2
8
π
2r2
.
Evaluate this at r = R, and place it into the x-component of the momentum equation.
Fx
B=
ρ
U−Γ
2
π
R
sin
θ
‘
(
) *
+
,
Ucos
θ
Rd
θ
θ
=0
θ
=2
π
∫+p∞+
ρ
UΓsin
θ
2
π
R−
ρ
Γ2
8
π
2R2
‘
(
)
*
+
,
cos
θ
Rd
θ
θ
=0
θ
=2
π
∫=0
In each case the various trigonometric functions produce a net zero for each term. The y–
component of the equation is:
Fy
B=
ρ
Γ
2
π
R
cos
θ
&
‘
( )
*
+
Ucos
θ
Rd
θ
θ
=0
θ
=2
π
∫+p∞+
ρ
UΓsin
θ
2
π
R−
ρ
Γ2
8
π
2R2
&
‘
(
)
*
+
sin
θ
Rd
θ
θ
=0
θ
=2
π
∫
.
Evaluate the integrals. The non-trivial ones become:
Fy
B=
ρ
UΓ
2
π
cos2
θ
d
θ
θ
=0
θ
=2
π
∫+
ρ
UΓ
2
π
sin2
θ
d
θ
θ
=0
θ
=2
π
∫=
ρ
UΓ
.
Hence, the force on control volume is directed along the positive y-axis (perpendicular to the
uniform stream) for positive Γ. When R → 0, this force remains the same. Therefore, to keep
the vortex stationary, an equal and opposite force must be produced by the hydrodynamic
interaction of the point vortex with the incoming flow. This hydrodynamic force is called the lift
(= L = –Fy), because it can support the weight of an object. The final result, which is true for all
two-dimensional lifting bodies, is:
L B =−
ρ
UΓ
. The minus sign appears here because of the
choice of the positive direction of circulation.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.14. Use the principle of conservation of mass (4.5) and an appropriate control volume
to show that maximum half thickness of the half body described by (7.30) or (7.31) is hmax =
qs/2U.
Solution 7.14. Place a control surface on the streamline that divides the fluid from the source
from the fluid that originates upstream. The velocity u is:
u=(u,v)=U+qs
2
π
rcos
θ
,qs
2
π
rsin
θ
!
“
#$
%
&
,
but this simplifies to u = (U, 0) far from the source (
r→ ∞
). Therefore, far downstream of the
source, the volume flux (per unit depth into the page) inside the CV must still be qs and its flow
speed will be U. Therefore, conservation of mass implies:
ρ
qs = 2
ρ
Uhmax, or hmax = qs/2U.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 7.15. By integrating the surface pressure, show that the drag on a plane half-body
(Figure 7.7) is zero.
Solution 7.15. For the half-body produced by a free stream of speed U and source of strength m,
the potential and velocity field are:
φ
=Ux +qs
2
π
ln x2+y2=Ur cos
θ
+qs
2
π
ln r
, and
u=(ur,u
θ
)=Ucos
θ
+qs
2
π
r,−Usin
θ
“
#
$%
&
‘
.
The stream function is given by:
where B is the spanwise dimension,
n=∇
ψ
∇
ψ
[ ]
r(
θ
)
is the outward normal from the dividing
streamline, and d
ξ
is a contour increment along the dividing streamline. Fortunately, some of the
intricacies of the geometry cancel out. First consider the dot product:
ex⋅n=ex⋅∇
ψ
∇
ψ
=
∂ψ ∂
x
ur
2+u
θ
2=−v
u
θ
1+ur
2u
θ
2
( )
=−v
u
θ
1
1+1r
( )
2dr d
θ
( )
.
Here v is the vertical velocity component, and the final equality follows from the streamline
condition,
dr ur=rd
θ
u
θ
, in r–
θ
polar coordinates (see Exercise 3.8). Now develop d
ξ
in r–
θ
polar coordinates:
d
ξ
=(dr)2+(rd
θ
)2=rd
θ
1+1r
( )
2dr d
θ
( )
.
Therefore:
ex⋅nd
ξ
=−
ν
u
θ
1
1+1r
( )
2dr d
θ
( )
rd
θ
1+1r
( )
2dr d
θ
( )
=−
ν
u
θ
rd
θ
=−qs
2
π
Ud
θ
.
where the final equality follows because
v=qs2
π
r
( )
sin
θ
, and u
θ
= –Usin
θ
. Thus, the drag
integral becomes: