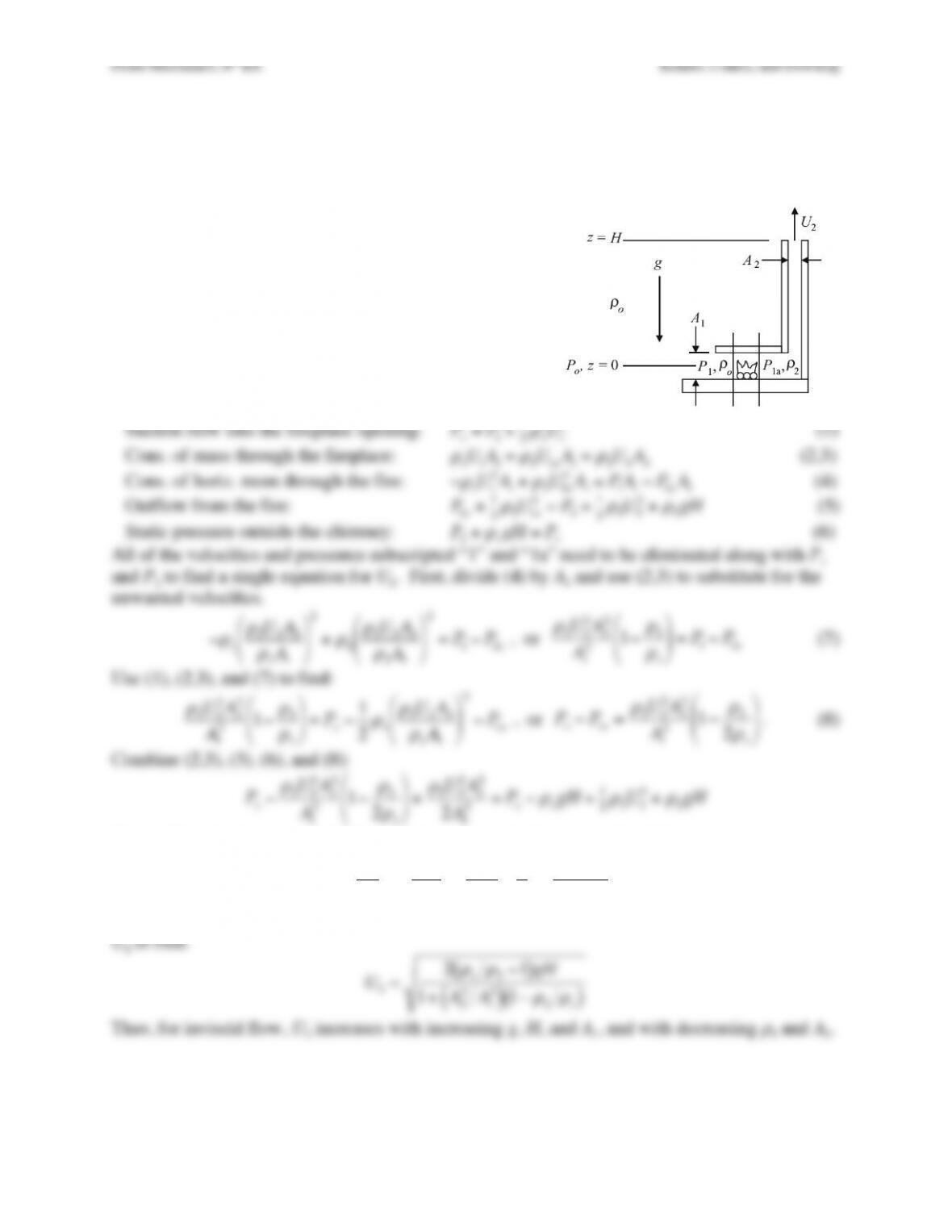
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
ρ
ui
∂
1
2uj
2
( )
∂
xi
=
ρ
giu+
∂
ujTij
( )
∂
xi
−Tij
∂
uj
∂
xi
=
ρ
giu+
∂
ujTij
( )
∂
xi
−Tij Sij =
ρ
giu+
∂
ujTij
( )
∂
xi
+pSii −
ρε
.
Note that
1
2uj
2
∂ ∂
xi
( )
ρ
ui
( )
=0
(because of the steady continuity equation) add it the left side and
integrate the two ends of this equation in the stationary control volume described in the statement
of part c) to find:
1
2
ρ
uj
2
( )
A*
∫uinidA =
ρ
gjujdV
V*
∫+ujTijnidA
A*
∫+pSii dV
V*
∫−
ρε
dV
V*
∫
.
Here Gauss’ divergence theorem has been used to covert the left side term and the second term
on the right to surface integrals. The body force term is zero because the dot product gjuj = 0 for
a horizontal flow with a vertical body force. The term involving the pressure is zero because Sii =
0 in this flow. Evaluating the remaining terms at locations x = 0 and x = L produces:
ρ
2−u(y)
[ ]
x=0
3+u(y)
[ ]
x=L
3
( )
0
b
∫dy = + up
[ ]
x=0−up
[ ]
x=L
( )
dy
0
b
∫+uT21
[ ]
y=b
0
L
∫dx −
µ
U2
b2
#
$
%&
‘
(
0
b
∫
0
L
∫dxdy
.
where the dimension of the control volume into the page, which is common to all terms, has been
divided out, and
ρε
has been evaluated with the result of part b). There is no contribution to the
surface work term from the lower plate because u = 0 on y = 0. The flow field is independent of
x, so the left-side integrand terms cancel, as do the integrand terms in the first right-side integral.
This leaves a balance of surface work and dissipation rate terms. On the upper plate at y = b, u =
U and T21 =
τ
21 =
µ
U/b; thus
0= + U
µ
U
b
“
#
$ %
&
‘
0
L
∫dx −
µ
U2
b2
“
#
$
%
&
‘
0
b
∫
0
L
∫dxdy →0=
µ
U2
bL−
µ
U2
b2Lb
= 0,
and the energy balance is verified.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.60. Determine the outlet speed, U2, of a chimney in terms of
ρ
o,
ρ
2, g, H, A1, and A2.
For simplicity, assume the fire merely decreases the density of the air from
ρ
o to
ρ
2 (
ρ
o >
ρ
2) and
does not add any mass to the airflow. (This mass flow assumption isn’t true, but it serves to keep
the algebra under control in this problem.) The relevant parameters are shown in the figure. Use
the steady Bernoulli equation into the inlet and from the
outlet of the fire, but perform a control volume analysis
across the fire. Ignore the vertical extent of A1 compared
to H, and the effects of viscosity.
Solution 4.60. Start from the stagnant air (Uo ≈ 0) on
the suction side of the chimney and use the steady
Bernoulli equation and a CV analysis to follow the flow
all the way to the chimney exit. The effects of viscosity
are ignored throughout this problem.
Suction flow into the fireplace opening:
P
o=P
1+1
2
ρ
oU1
2
(1)
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.61. A hemispherical vessel of radius R containing an inviscid constant-density liquid
has a small rounded orifice of area A at the bottom. Show that the time required to lower the
level from h1 to h2 is given by
t2−t1=2
π
A2g
2R
3h1
3 2 −h2
3 2
( )
−1
5h1
5 2 −h2
5 2
( )
$
%
& ‘
(
)
Solution 4.61. Let z denote the height of the
liquid in the vessel. Although there is slight
unsteadiness even when
π
R2 >> A, use the
steady Bernoulli equation between the free
π
r2dz dt
( )
=AUe
. (2)
Eliminate Ue from (1) and (2) to find:
2gz =
π
2r4
A2−1
$
%
&
‘
(
) dz
dt
$
%
& ‘
(
)
2
, or
−
π
2r4
A2−1
$
%
&
‘
(
)
1 2
dz
dt
$
%
& ‘
(
) =2gz
,
where the minus sign arises because z decreases as the tank empties. When
π
r2 >> A, this relationship
R
r
h1
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.62. Water flows through a pipe in a gravitational field as shown in the accompanying
figure. Neglect the effects of viscosity and surface tension. Solve the appropriate conservation
equations for the variation of the cross–sectional area of the fluid column A(z) after the water has left
the pipe at z = 0. The velocity of the fluid at z = 0 is uniform at Vo and the cross–sectional area is A0.
Solution 4.62. Using the z coordinate shown and the steady Bernoulli
equation between the pipe exit and the vertical location z:
patm +1
2
ρ
Vo
2=patm +1
2
ρ
u2(z)+
ρ
gz
, (1)
where u(z) is the flow speed at vertical location z. For an incompressible
liquid, conservation of mass reduces to conservation of volume:
VoAo=u(z)A(z)
, (2)
Eliminate u(z) from (1) and (2) to find:
Vo
2=VoAoA(z)
( )
2+2gz
.
Solve for A(z) to find:
A(z)=VoAo
Vo
2−2gz
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.63. Redo the solution for the orifice-in-a-tank problem allowing for the fact that in
Fig. 4.16, h = h(t) but ignoring fluid acceleration. Estimate how long it takes for the tank take to
empty.
Solution 4.63. Let h(t) denote the height of the liquid in the vessel. Let the tank and orifice cross
sectional areas be At and Ao, respectively. Although there is slight unsteadiness even when At >> Ao,
use the steady Bernoulli equation between the free surface and the orifice at the bottom of the tank:
patm +1
2
ρ
−dh
dt
$
%
& ‘
(
)
2
+
ρ
gh =patm +1
2
ρ
Uo
2
(1)
where Uo is the flow speed exiting the tank throught the orifice, and the minus sign appears because
the water level is decreasing. For an incompressible liquid, conservation of mass reduces to
conservation of volume:
At−dh dt
( )
=AoUo
. (2)
Eliminate Uo from (1) and (2) to find:
2gh =At
2
Ao
2−1
#
$
%
&
‘
( −dh
dt
#
$
% &
‘
(
2
, or
2gAt
2
Ao
2−1
#
$
%
&
‘
(
−1 2
=−1
h
dh
dt
,
which is a differential equation for h(t). The left side is merely a constant. Integrate this equation
from t = 0 when h = h0 to t = tf when h = 0 to find:
2gAt
2
Ao
2−1
#
$
%
&
‘
(
−1 2
tf=−h1 2
1 2
#
$
%
&
‘
(
h0
0
=2h0
1 2
,
which can be rearranged to yield:
tf=2h0
g
At
2
Ao
2−1
#
$
%
&
‘
(
1 2
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.64. Consider the planar flow of Example 3.5, u = (Ax,–Ay), but allow A = A(t) to
depend on time. Here the fluid density is
ρ
, the pressure at the origin or coordinates is po, and
there are no body forces.
a) If the fluid is inviscid, determine the pressure on the x-axis, p(x,0,t) as a function of time from
the unsteady Bernoulli equation.
b) If the fluid has viscosities
µ
and
µυ
, determine the pressure throughout the flow field, p(x,y,t),
from the x-direction and y-direction differential momentum equations.
c) Are the results for parts a) and b) consistent with each other? Explain your findings.
Solution 4.64. a) Use (4.82):
∂
u
∂
t⋅ds
1
2
∫+1
2
u2+gz +p
ρ
#
$
%&
‘
(
2
=1
2
u2+gz +p
ρ
#
$
%&
‘
(
1
, and choose the
streamline along the x-axis from the origin to the location x:
∂
u
∂
t
dx
0
x
∫+1
2
u2+p
ρ
“
#
$%
&
‘
x
=1
2
u2+p
ρ
“
#
$%
&
‘
0
or
dA
dt x dx
0
x
∫+1
2A2x2+p(x, 0, t)
ρ
“
#
$%
&
‘=0+po
ρ
.
The integral is elementary, so after some rearrangement the last equation implies:
p(x, 0, t)−po
ρ
=−dA
dt +A2
“
#
$%
&
‘x2
2
.
b) For this velocity field
∇ ⋅ u=0
, so the flow is incompressible. Thus, the relevant version of
the Navier-Stokes momentum equation is (4.39b) with g = 0:
ρ
Du
Dt =−∇p+
µ
∇2u
.
Using u = (Ax,–Ay) in this equation leads to:
ρ
dA
dt x+A2x
!
“
#$
%
&ex+−dA
dt y+A2y
!
“
#$
%
&ey
(
)
*+
,
–=−∂p
∂x
ex−∂p
∂y
ey+0
.
Separating this into two component equations and integrating yields:
p=−
ρ
dA
dt +A2
“
#
$%
&
‘x2
2+B(y,t)
and
p=−
ρ
−dA
dt +A2
“
#
$%
&
‘y2
2+C(x,t)
,
where B and C are functions of integration. These two equations are consistent and match the
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.65. A circular plate is forced down at a steady velocity Uo against a flat surface.
Frictionless incompressible fluid of density
ρ
fills the gap h(t). Assume that h << ro, the plate
radius, and that the radial velocity ur(r,t) is constant across the gap.
a) Obtain a formula for ur(r,t) in terms of r, Uo, and h.
b) Determine ∂ur(r,t)/∂t.
c) Calculate the pressure distribution under the plate assuming that p(r = ro) = 0.
Solution 4.65. a) Choose a cylindrical control volume that fits under the moving disk, and has
radius r and height h. Conservation of mass for this control volume implies:
dM
dt =0=d
dt
ρπ
r2h
( )
+
ρ
ur⋅2
π
rh
, or
0=r2dh
dt +2rhur
In this problem,
dh dt =−Uo
, so the above conservation of mass equation requires:
ur=rUo2h
.
b) Differentiate the part a) result:
∂
ur
∂
t=−rUo
2h2
dh
dt =rUo
2
2h2
c) Use this result in the unsteady Bernoulli equation. The streamlines are radial so the unsteady
term can be evaluated on paths directed through the origin from a radial distance r to the radial
distance ro.
ur
2(r)
2+p(r)
ρ
=
∂
ur
∂
tdr
r
ro
∫+ur
2(ro)
2+p(ro)
ρ
.
Now substitute p(r = ro) = 0, and the results of parts a) and b) to find:
r2Uo
2
8h2+p(r)
ρ
=Uo
2
2h2
ro
2
2−r2
2
$
%
&
‘
(
) +ro
2Uo
2
8h2
Solve for the pressure at distance r:
p(r)=3
ρ
Uo
2
8h2ro
2−r2
( )
. Such a distribution is reasonable
because it is highest at r = 0 and drops toward the edges of the disk; thus, the pressure gradient
pushes fluid out from underneath the disk.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.66. A frictionless, incompressible fluid with density
ρ
resides in a horizontal nozzle
of length L having a cross sectional area that varies smoothly between Ai and Ao via:
A(x)=Ai+Ao−Ai
( )
f x L
( )
, where f is a function that goes from 0 to 1 as x/L goes from 0 to 1.
Here the x-axis lies on the nozzle’s centerline, and x = 0 and x = L are the horizontal locations of
the nozzle’s inlet and outlet, respectively. At t = 0, the pressure at the inlet of the nozzle is raised
to pi > po, where po is the (atmospheric) outlet pressure of the nozzle, and the fluid begins to flow
horizontally through the nozzle.
a) Derive the following equation for the time-dependent volume flow rate Q(t) through the
nozzle from the unsteady Bernoulli equation and an appropriate conservation-of-mass
relationship.
˙
Q (t)
Ai
Ai
A(x)
x=0
x=L
∫dx +Q2(t)
2
1
Ao
2−1
Ai
2
$
%
&
‘
(
) =pi−po
ρ
$
%
&
‘
(
)
b) Solve the equation of part a) when
f x L
( )
=x L
.
c) If
ρ
= 103 kg/m3, L = 25 cm, Ai = 100 cm2, Ao = 30 cm2, and pi – po = 100 kPa for t ≥ 0, how
long does it take for the flow rate to reach 99% of its steady-state value?
Solution 4.66. a) A conservation of mass equation and the unsteady Bernoulli equation are
needed here. Considering inflow and outflow at the inlet and outlet of the nozzle produces:
volume flux = Q(t) = Ui(t)Ai = Uo(t)Ao, where the ‘o’ and ‘i’ subscripts stand for outlet and inlet,
respectively. Now consider the streamline that follows a horizontal path along the centerline of
the nozzle:
pi
ρ
+Ui
2
2+gzi=
∂
u
∂
t⋅ds
x=0
x=L
∫+po
ρ
+Uo
2
2+gzo
.
This streamline lies on the x-axis so that
ds=exdx
and
u(x,t)
[ ]
centerline =exUi(t)AiA(x)
. In
addition, zi = zo, so
pi−po
ρ
=
∂
∂
tUi(t)Ai
A(x)
ex
%
&
‘ (
)
*
⋅exdx
x=0
x=L
∫+Ui
2
2
Ai
2
Ao
2−1
%
&
‘
(
)
*
.
Perform the dot product, set
Q(t)=Ui(t)Ai
, and rearrange:
˙
Q (t)
Ai
Ai
A(x)
x=0
x=L
∫dx +Q2(t)
2
1
Ao
2−1
Ai
2
$
%
&
‘
(
) =pi−po
ρ
.
where the over-dot signifies a time derivative. This is a first-order nonlinear differential
equation for Q(t).
b) When
A(x)=Ai+Ao−Ai
( )
f x L
( )
=Ai1+Ao−Ai
( )
Ai
x
L
#
$
%
&
‘
(
, then
Ai
A(x)
x=0
x=L
∫dx =1+Ao−Ai
( )
Ai
x
L
$
%
&
‘
(
)
−1
x=0
x=L
∫dx =L
β
ln 1+
β
( )
where
β
=Ao−Ai
( )
Ai
. Thus, the differential equation for the volume flux becomes:
˙
Q (t)+
β
Ai
2Lln 1+
β
( )
1
Ao
2−1
Ai
2
$
%
&
‘
(
)
Q2(t)=
β
Ai
Lln 1+
β
( )
pi−po
ρ
$
%
&
‘
(
)
.
The coefficients are clumsy, but they are constants so the above equation can be rewritten:
˙
Q (t)+BQ2(t)=C
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Here the fluid starts from rest, so the initial condition Q(0) = 0 allows D to be determined:
D=−1 2Qss
( )
, thus
Q(t)=C
B1−2
1+exp 2 BCt
{ }
#
$
%
%
&
‘
(
(
where
C
B=Qss =2Ai
2Ao
2
Ai
2−Ao
2
pi−po
ρ
$
%
&
‘
(
)
, and
BC =
β
Ai
Lln 1+
β
( )
Ai
2−Ao
2
2Ai
2Ao
2
pi−po
ρ
%
&
‘
(
)
*
.
c) 99% of the steady flow rate will be reached when:
exp +2BC t
{ }
=199
. Thus for
β
=(Ao−Ai)Ai=(30cm2−100cm2) 100cm2=−0.7
, the requisite time is:
t=ln(199)
2BC =ln(199)
2
0.25mln(1−0.7)
(−0.7)(0.01m2)
2(0.01m2)2(0.003m2)2
(0.01m2)2−(0.003m2)2⋅103kg /m3
105Pa
$
%
&
‘
(
)
1 2
.
= (2.64665)(42.999)(4.4475×10–4) = 0.051 seconds.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.67. For steady constant-density inviscid flow with body force per unit mass
g=−∇Φ
, it is possible to derive the following Bernoulli equation:
p+1
2
ρ
u2+
ρ
Φ=
constant
along a streamline.
a) What is the equivalent form of the Bernoulli equation for constant-density inviscid flow that
appears steady when viewed in a frame of reference that rotates a constant rate about the z-axis,
i.e. when
Ω=(0,0,Ωz)
with Ωz constant?
b) If the extra term found in the Bernoulli equation is considered a pressure correction. Where
on the surface of the earth (i.e. at what latitude) will this pressure correction be the largest?
What is the absolute size of the maximum pressure correction when changes in R on a streamline
are 1 m, 1 km, and 103 km.
Solution 4.67. a) The steady-flow Bernoulli equation can be derived from a dot product of the
fluid velocity, u, with the momentum equation. For this problem, the flow appears steady in a
frame of reference rotating at a constant rate, so the point of departure for this problem is the
momentum equation (4.45) in a non-interial frame of reference (the primes denote quantities in
the non-inertial frame):
ρ
#
D #
u
Dt =−#
∇ p+
ρ
g+
µ
#
∇ 2#
u −
ρ
dU
dt +dΩ
dt ×#
x +2Ω×#
u +Ω×Ω×#
x
( )
(
)
*
+
,
–
.
This equation can be simplified for steady (∂/∂t = 0) inviscid (
µ
= 0) flow in a steadily rotating (
∂
Ω
∂
t=0
) co-ordinate system that does not accelerate (
dUdt =0
).
ρ
#
u ⋅ ∇
( )
#
u =−#
∇ p+
ρ
g+
µ
#
∇ 2#
u −
ρ
2Ω×#
u +Ω×Ω×#
x
( )
[ ]
.
The term on the left can be rewritten using a vector identity (B3.9), the body force term can be
replaced with the gradient of the body-force potential
g=−∇Φ
, and the last term on the right can
be evaluated when
Ω=(0,0,Ωz)
:
ρ
#
∇ 1
2#
u 2
%
&
‘ (
)
* +
ρ
#
∇ ×#
u
( )
×#
u =−#
∇ p−
ρ
#
∇ Φ −
ρ
2Ω×#
u − Ωz
2#
x #
e
x+#
y #
e
y
( )
[ ]
.
The terms containing
Ωz
2
can be combined and put under a gradient:
−Ωz
2$
x $
e
x+$
y $
e
y
( )
=−$
∇ 1
2Ωz
2$
x 2+$
y 2
( )
&
‘
( )
*
+
.
Collect all the terms involving a gradient together on the left side of the equation:
“
∇
ρ
2“
u 2+p+
ρ
Φ − 1
2
ρ
Ωz
2“
x 2+“
y 2
( )
(
)
* +
,
– =−2
ρ
Ωד
u −
ρ
“
∇ ד
u
( )
ד
u
Now take the dot product of this equation with u´ noting that this will zero the right side of the
equation because of the properties of the cross product:
“
u ⋅“
∇
ρ
2“
u 2+p+
ρ
Φ − 1
2
ρ
Ωz
2“
x 2+“
y 2
( )
)
*
+ ,
–
. =0
This implies that
ρ
2#
u 2+p+
ρ
Φ − 1
2
ρ
Ωz
2#
x 2+#
y 2
( )
= constant along a streamline in the rotating
frame. The sign of the new term can be checked by considering u´ = 0 with Φ = 0 (standard
solid body rotation viewed from the inertial frame of reference) and computing the pressure
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.68. Starting from (4.45) derive the following unsteady Bernoulli equation for inviscid
incompressible irrotational fluid flow observed in a non-rotating frame of reference undergoing
acceleration dU/dt with its z-axis vertical.
∂u
∂t⋅ds
1
2
∫ +u2
2+p
ρ
+gz +x⋅dU
dt
$
%
&
&
‘
(
)
)
2
=u2
2+p
ρ
+gz +x⋅dU
dt
$
%
&
&
‘
(
)
)
1
.
Solution 4.68. Start from (4.45) with Ω = 0, work in the accelarting frame of reference, and drop
the “primes” from the coordinates.
ρ
Du
Dt
!
“
#$
%
&=−∇p+
ρ
g−dU
dt
)
*
+,
–
.+
µ
∇2u
(1)
Here the acceleration of the coordinate system is dU/dt and fluid is frictionless so
µ
= 0. Thus,
(1) becomes:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.69. Using the small slope version of the surface curvature
1R
1≈d2
ζ
dx 2
, redo
Example 4.7 to find h and
ζ
(x) in terms of x,
σ
,
ρ
, g and
θ
. Show that the two answers are
consistent when
θ
approaches
π
/2.
Solution 4.69. Pressure matching between the air just above and the liquid just beneath the free
surface where the deflection is
ζ
(x) produces:
patm =p
( )
air =p
( )
liquid +
σ
1
R1
+1
R2
#
$
%
&
‘
( =patm −
ρ
g
ζ
+
σ
1
R1
+1
R2
#
$
%
&
‘
(
,
where the Laplace pressure must be added to the liquid pressure, and the radii of curvature are
and must be simplified for
θ
approaching
π
/2. The complexity here comes from the cosh-inverse
function which can be written in terms of a natural logarithm:
cosh−1(y)=ln y±y2−1
( )
.
When
θ
→
π
2
, then
cot
θ
→0
. Thus, in this limit h/
δ
<< 1 and
ζ
/
δ
<< 1. Therefore, the exact
solution can be simplified using:
cosh−1(2
δ ζ
)≈ln 4
δ ζ
( )
+…
and
cosh−1(2
δ
h)≈ln 4
δ
h
( )
+…
, and
(4 −
ζ
2/
δ
2)1/2 ≈2+…
, and
(4 −h2/
δ
2)1/2 ≈2+…
Substituting these replacements into the exact solution produces:
ln 4
δ ζ
( )
−2−ln 4
δ
h
( )
+2+… = x/
δ
, or
ln h
ζ
( )
+… = x/
δ
.
Exponentiating both sides of the last expression and solving for z produces
ζ
=hexp −x/
δ
{ }
,
which the same as the small-slope solution. Thus, the exact and small-slope solutions are
consistent.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.70. An spherical bubble with radius R(t), containing gas with negligible density,
creates purely radial flow, u = (ur(r,t), 0, 0), in an unbounded bath of a quiescent incompressible
liquid with density
ρ
and viscosity
µ
. Determine ur(r,t) in terms of R(t), its derivatives. Ignoring
body forces, and assuming a pressure of p∞ far from the bubble, find (and solve) an equation for
the pressure distribution, p(r,t), outside the bubble. Integrate this equation from r = R to
r→ ∞
,
and apply an appropriate boundary condition at the bubble’s surface to find the Rayleigh-Plesset
equation for the pressure pB(t) inside the bubble:
pB(t)−p∞
ρ
=Rd2R
dt2+3
2
dR
dt
#
$
%&
‘
(
2
+4
µ
ρ
R
dR
dt +2
σ
ρ
R
,
where
µ
is the fluid’s viscosity and
σ
is the surface tension.
Solution 4.70. The flow of the liquid is purely radial and incompressible so the continuity
equation and radial momentum equation in spherical coordinates are:
1
r2
∂
∂r
r2ur
( )
=0
and
∂ur
∂t+ur
∂ur
∂r=−1
ρ
∂p
∂r+
µ
ρ
1
r2
∂
∂r
r2∂ur
∂r
#
$
%&
‘
(−2ur
r2
#
$
%&
‘
(
.
The first equation is readily integrated to find: ur = A(t)/r2. The function of integration, A(t), can
be evaluated by requiring ur(R,t) = dR/dt, and this leads to:
ur(r,t)=R2(t)
dR
.
Substituting this relationship into the radial momentum equation leads to:
∂
∂t
R2(t)
r2
dR
dt
“
#
$%
&
‘+R2(t)
r2
dR
dt
“
#
$%
&
‘∂
∂r
R2(t)
r2
dR
dt
“
#
$%
&
‘=−1
ρ
∂p
∂r+
µ
ρ
1
r2
∂
∂rr2∂
∂r
R2(t)
r2
dR
dt
“
#
$%
&
‘
“
#
$%
&
‘−2
r2
R2(t)
r2
dR
dt
“
#
$%
&
‘
“
#
$
$
%
&
‘
‘
,
and this is an equation for the pressure. Carefully evaluate derivatives and simplify the various
terms:
2R(t)
r2
dR
dt
!
“
#$
%
&
2
+R2(t)
r2
d2R
dt2−2R4(t)
r5
dR
dt
!
“
#$
%
&
2
=−1
ρ
∂p
∂r+
µ
ρ
0!
( )
.
Interestingly, the viscous terms cancel out at this point. Rearrange this equation and integrate in r
from R to ∞:
dp
ρ
=
p(R)
∞
∫p∞−p(R)
ρ
=−2R(t)dR
dt
$
%
&‘
(
)
2dr
r2
R
∞
∫−R2(t)d2R
dt2
dr
r2
R
∞
∫+2R4(t)dR
dt
$
%
&‘
(
)
2dr
r5
R
∞
∫
=−2R(t)dR
dt
$
%
&‘
(
)
2
−1
r
*
+
,–
.
/
R
∞
−R2(t)d2R
dt2−1
r
*
+
,–
.
/
R
∞
+2R4(t)dR
dt
$
%
&‘
(
)
2
−1
4r4
*
+
,–
.
/
R
∞
=−2dR
dt
$
%
&‘
(
)
2
−R(t)d2R
dt2+1
2
dR
dt
$
%
&‘
(
)
2
=−R(t)d2R
dt2−3
2
dR
dt
$
%
&‘
(
)
2
.
This produces an equation for the pressure p(R) in the liquid at r = R, but the pressure pB(t) inside
the bubble is sought.
Equation (4.93) provides the means to jump from the liquid to the gas side of the bubble’s
surface. Here we assume that no mass is transfered and that “1” indicates the inside the bubble
while “2” indicates the outside of the bubble. In this case (4.93) reduces to:
0=−p2−p1
( )
+(
τ
rr )2−(
τ
rr )1
( )
−2
σ
R
,