Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
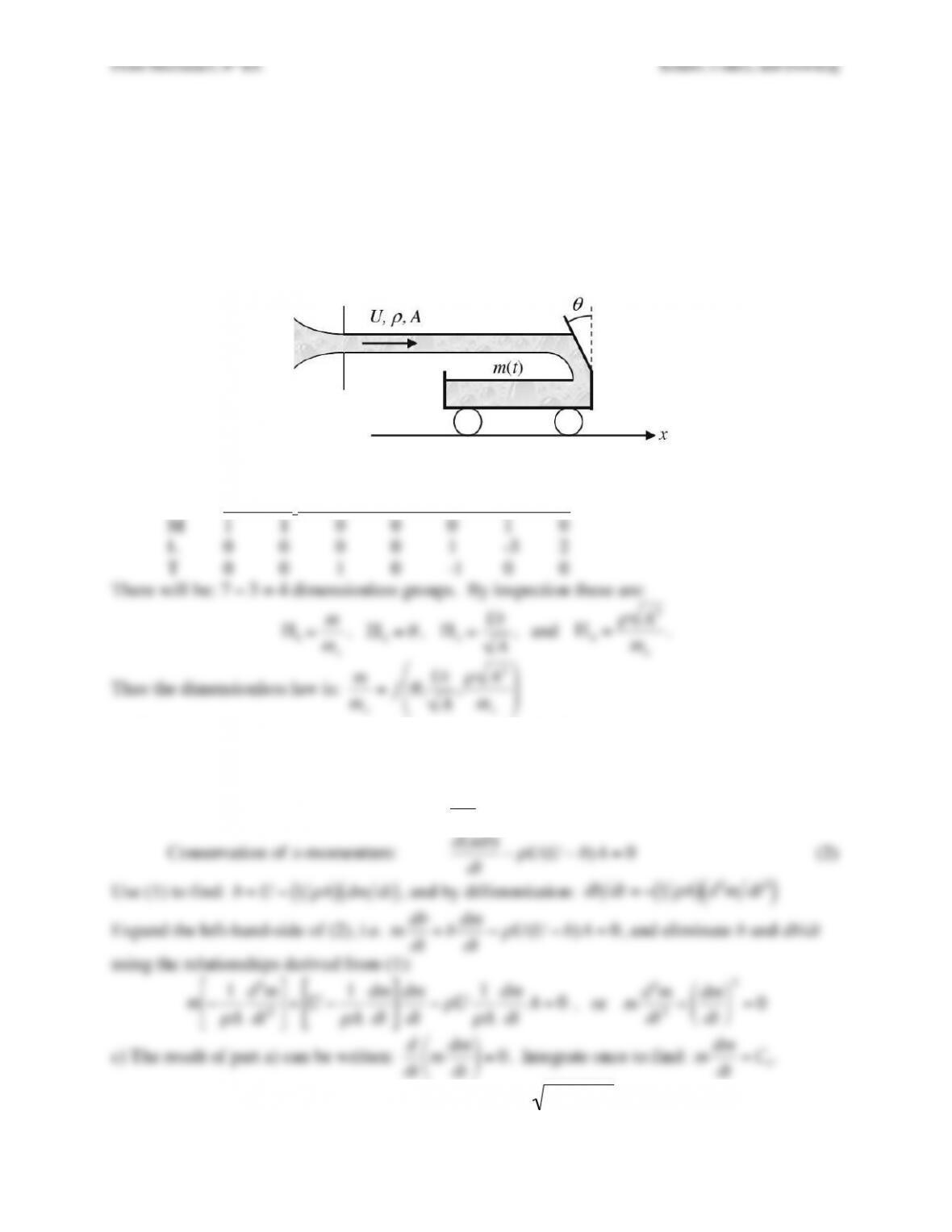
Exercise 4.29. Attach a drinking straw to a 15-cm-diameter cardboard disk with a hole at the
center using tape or glue. Loosely fold the corners of a standard piece of paper upward so that
the paper mildly cups the cardboard disk (see drawing). Place the cardboard disk in the central
section of the folded paper. Attempt to lift the loosely folded paper off a flat surface by blowing
or sucking air through the straw.
a) Experimentally determine if blowing or suction is more effective in lifting the folded paper.
b) Explain your findings with a control volume analysis.
Solution 4.29. a) It would seem that the only way to lift the paper would be to suck on the straw.
However, blowing is found to be just as successful!
b) This can be explained with a control volume analysis. Choose a control volume that encloses a
portion of the fluid between the paper and the cardboard disk. For simplicity assume that the
flow is axisymmetric, constant density, and inviscid. Also assume that the paper and the
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Wp≤2
π
−p(r)+p∞
[ ]
rdr
r=o
r=R
∫
(†)
where Wp is the weight of the paper. This pressure integral can be estimated from the vertical
momentum equation for a control volume like that shown above with radius R:
ρ
Ui
As
∫Uiez⋅ez
( )
dA =
ρ
Ui
2As=−2
π
p(r)rdr
r=rs
r=R
∫−piAs+2
π
p(r)rdr
r=0
r=R
∫
.
The first and second integrals on the right side come from the cardboard and paper surfaces,
respectively. Rearrange this, use the Bernoulli equation, and then approximate the flow under
the cardboard as purely radial for r > rs.
2
π
p(r)rdr
r=0
r=R
∫=
ρ
Ui
2As+2
π
p(r)rdr
r=rs
r=R
∫+piAs
2
π
p(r)rdr
r=0
r=R
∫=
ρ
Ui
2As+2
π
p∞+1
2
ρ
uR
2−1
2
ρ
ur
2
( )
rdr
r=rs
r=R
∫+piAs
.
Now start reconstructing the integral of the pressure difference (†):
2
π
p∞−p(r)
[ ]
rdr
r=0
r=R
∫=−
ρ
Ui
2As−2
π
1
2
ρ
uR
2−1
2
ρ
ur
2
( )
rdr
r=rs
r=R
∫−piAs+p∞As
Inserting
ur=uRR r
( )
yields:
2
π
p∞−p(r)
[ ]
rdr
r=0
r=R
∫=−
ρ
Ui
2As−1
2
πρ
uR
2R2−rs
2
( )
+
πρ
uR
2R2ln R−ln rs
( )
−piAs+p∞As
.
Collect terms and introduce Wp:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.30. A compression wave in a long gas-filled constant-area duct propagates to the left
at speed U. To the left of the wave, the gas is quiescent with uniform density
ρ
1 and uniform
pressure p1. To the right of the wave, the gas has uniform density
ρ
2 (>
ρ
1) and uniform pressure
is p2 (> p1). Ignore the effects of viscosity in this problem.
a) Formulate a dimensionless scaling law for U in terms of the pressures and densities.
b) Determine U in terms of
ρ
1,
ρ
2, p1, and p2 using a control volume.
c) Put your answer to part b) in dimensionless form and thereby determine the unknown function
from part a).
d) When the density and pressure changes are small, they are proportional:
p2−p1=c2
ρ
2−
ρ
1
( )
for
ρ
2−
ρ
1
( )
ρ
2<<1
,
where
c2=∂p∂
ρ
( )
s
. Under these conditions, U is associated with what common property of the
gas?
Solution 4.30. a) Use dimensional analysis. There are five parameters but there are only two
independent dimensions ([density] and [velocity] because [density x velocity2] = [pressure]), so
there are three dimensionless groups. Three satisfactory groups are easily found by inspection:
Π1=
ρ
1
U2p1
,
Π2=
ρ
1
ρ
2
, and
Π3=p1p2
. Thus, the scaling law is:
ρ
1
U2p1=fn
ρ
1
ρ
2,p1p2
( )
.
b) Place a moving control volume around the compression wave with vertical surfaces on the left
and on the right of the wave, similar to Example 4.6. The contents of this control volume do not
vary in time so:
Cons. of mass
−
ρ
1
UA +
ρ
2(U+u2)A=0
Cons. of x-momentum
−
ρ
1
U2A+
ρ
2(U+u2)2A=p1A−p2A
where A is the cross section of the duct, and u2 is the horizontal velocity of the gas on the right of
the wave in a stationary frame of reference. Divide both equations by A, and use the first
equation to eliminate U + u2 from the second equation to find:
−
ρ
1
U2+
ρ
2
ρ
1
U
ρ
2
( )
2=p1−p2
.
Solve this equation to find:
U=p2−p1
ρ
11−
ρ
1
ρ
2
( )
.
c) Manipulate the result of part b) to find:
ρ
1
U2
p1
=p2p1−1
1−
ρ
1
ρ
2
( )
, or
Π1=fn(Π2,Π3)=Π3
−1−1
1− Π2
.
d) Start with the result of part b) and insert the small-change approximation:
U=p2−p1
ρ
11−
ρ
1
ρ
2
( )
=c2
ρ
2−
ρ
1
( )
ρ
11−
ρ
1
ρ
2
( )
=c
ρ
2
ρ
1
→c
as
ρ
2
ρ
1
→1
Thus, in this limit, U is associated with c, the speed of sound.
U!
p1,
ρ
1!
u1 = 0!
p2,
ρ
2!
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.31. A rectangular tank is placed on wheels and is given a constant horizontal
acceleration a. Show that, at steady state, the angle made by the free surface with the horizontal
is given by tan
θ
= a/g.
Solution 4.31. Make a drawing and add some
dimensions to the tank. Place the control
volume around the water inside the rolling
accelerates. The horizontal component of the
momentum equation is:
d
dt
ρ
udV
V*(t)
∫+
ρ
u u −at
( )
ex⋅n
S*(t)
∫dA =fxdA
S*(t)
∫
where the CV velocity, b, has been set to b = atex, so that db/dt = aex is the specified steady
horizontal acceleration. The body force term does not appear in the above equation since
gravity acts in the vertical direction. At steady state, the fluid velocity will equal the velocity of
the moving tank so the flux terms will be zero, and the pressure forces will be hydrostatic:
∇p=
ρ
g
, where p is the pressure and g is the body force acceleration. In this situation, g has
both horizontal and vertical components. However, the vertical component of the hydrostatic
equation,
∂
p
∂
y=−
ρ
g
, is not changed by the horizontal acceleration. This equation integrates
to:
p=A(x)−
ρ
gy
where A(x) is the function of integration; it cannot depend on y. Here we
know that atmospheric pressure occurs on the surface of the water so the pressure on the left and
right sides of the tank are
p1(y)=po+
ρ
g(h1−y)
and
p2(y)=po+
ρ
g(h2−y)
, respectively.
Thus, the horizontal momentum conservation equation becomes:
Ma =
ρ
g(h1−y)Bdy
0
h1
∫−
ρ
g(h2−y)Bdy =
ρ
gB
2
0
h2
∫h1
2−h2
2
( )
,
where B is the dimension of the tank into the page, and the CV-surface integration of po leads (as
usual) to no net force. Now, use the two ends of this extended equality and divide by M noting
that
M=
ρ
BL (h1+h2) 2
where L is the horizontal length of the tank:
a=g
Lh1−h2
( )
=gtan
θ
, so
tan
θ
=a
g
θ
!g!
y!
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.32. Starting from rest at t = 0, an airliner of mass M accelerates at a constant rate
a=aex
into a headwind,
u=−uiex
. For the following items, assume that: i) the x-component of
the fluid velocity is –ui on the front, sides, and back upper half of the control volume (CV), ii)
the x-component of the fluid velocity is –uo on the back lower half of the CV, iii) changes in M
can be neglected, iv) changes of air momentum inside the CV can be neglected, and v)
frictionless wheels. In addition, assume constant air density
ρ
and uniform flow conditions exist
on the various control surfaces. In your work, denote the CV’s front and back area by A. (This
approximate model is appropriate for real commercial airliners that have the engines hung under
the wings).
a) Find a dimensionless scaling law for uo at t = 0 in terms of ui,
ρ
, a, M, and A.
b) Using a CV that encloses the airliner (as shown) determine a formula for uo(t), the time-
dependent air velocity on the lower half of the CV’s back surface.
c) Evaluate uo at t = 0, when M = 4 x 105 kg, a = 2.0 m/s2, ui = 5 m/s,
ρ
= 1.2 kg/m3, and A = 1200
m2. Would you be able to walk comfortably behind the airliner?
Solution 4.32. a) The units matrix is:
uo ui
ρ
a M
A
––––––––––––––––––––––––––––––––
M 0 0 1 0 1 0
b) For constant acceleration from rest, the CV’s velocity is b = atex. Conserve mass:
d
dt M+Mair
( )
+
ρ
upper back
∫+
lower back
∫+
front
∫+
sides
∫
$
%
&
‘
(
)
(u−b)⋅ndA =0
Drop the time derivative terms and evaluate the first three surface integrations:
ρ
(−ui−at)ex⋅(−ex)A
2+
ρ
(−uo−at)ex⋅(−ex)A
2+
ρ
(−ui−at)ex⋅(ex)A+
ρ
(u−b)⋅ndS
sides
∫=0
Simplify to find:
(u−b)⋅ndS
sides
∫=(ui−uo)A
2
. (*)
Note that ui must equal uo if the integral over the sides of the CV is not included. Now conserve
horizontal momentum noting that there are no external forces on the CV.
d
dt Mat +Mairuair
( )
+
ρ
upper back
∫+
lower back
∫+
front
∫+
sides
∫
$
%
&
‘
(
)
(u⋅ex)(u−b)⋅ndS =0
Drop the air term from the time derivative, set dM/dt = 0, and evaluate the first three surface
integrations
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.33. A cart that can roll freely in the x-direction deflects a horizontal water jet into its
tank with a vane inclined to the vertical at an angle
θ
. The jet issues steadily at velocity U with
density
ρ
, and has cross sectional area A. The cart is initially at rest with a mass of mo. Ignore the
effects of surface tension, and the cart’s rolling friction and wind resistance in your answers.
a) Formulate dimensionless law for the mass, m(t), in the cart at time t in terms of t,
θ
, U,
ρ
, A,
and mo.
b) Formulate a differential equation for m(t).
c) Find a solution for m(t) and put it in dimensionless form.
Solution. 4.33. a) Create the parameter matrix
m mo t
θ
U
ρ
A
M 1 1 0 0 0 1 0
b) Choose a control volume that contains: i) the cart, ii) the fluid in the cart, and iii) the tip of the
fluid jet that just hits the deflector plate. The vane is presumed to be entirely inside the CV. Let
b(t) be the cart’s velocity in the positive x-direction.
Conservation of mass:
dm
dt =
ρ
(U−b)A
(1)
Conservation of x-momentum:
d(mb)
dt −
ρ
U(U−b)A=0
(2)
Integrate a second time and take the square root:
m(t)=2C1t+C2
. Evaluate the constants
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.34. A spherical balloon of mass Mb is filled with air of density
ρ
and is initially
stationary at x = 0 with diameter Do. At t = 0, an opening of area A is created and the balloon
travels horizontally along the x-axis. The aerodynamic drag force on the balloon is given by
(1/2)
ρ
U2
π
(D/2)2CD, where: CD is a constant, U(t) is the velocity of the balloon, and D(t) is the
current diameter of the balloon. Assume incompressible air flow and that D(t) is known.
a) Find a differential equation for U that includes: Mb,
ρ
, CD, D, and A.
b) Solve the part a) equation when CD = 0, and the mass flow rate of air out of the balloon,
m
, is
constant, so that the mass of the balloon and its contents are
Mb+
ρπ
6
Do
3−
mt
at time t.
c) What is the maximum value of U under the conditions of part b)?
Solution 4.34. a) Enclose the balloon with an accelerating control volume that moves with
velocity b = U(t)ex. Denote the velocity of the air leaving the balloon by u = –Ueex + U(t)ex,
where Ue is the speed of the exiting air stream relative to the balloon and it appears with a minus
sign because it is oriented opposite to the x-direction.
For this control volume, the the total mass inside at any time is Mb +
ρ
(
π
/6)D3, and the
x!
D!
A!
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.35. For time t < 0, a rolling water tank with frictionless wheels, horizontal cross-
sectional area A, and empty mass M sits stationary while filled to a depth ho with water of density
ρ
. At t = 0, the outlet of the tank is opened and the tank starts moving to the right. The outlet tube
has cross sectional area a and contains a narrow-passage honeycomb so that the flow speed
through the tube is Ue = gh/R, where R is the specific flow resistivity of the honeycomb material,
g is the acceleration of gravity, and h(t) is the average water depth in the rolling tank for t > 0.
Here, Ue is the leftward speed of the water with respect to the outlet tube; it is independent of the
speed b(t) of the rolling tank. Assume uniform flow at the pipe outlet and use an appropriate
control volume analysis for the following items.
a) By conserving mass, develop a single equation for h(t) in terms of a, A, g, R, and t.
b) Solve the part a) equation for h(t).
c) By conserving horizontal momentum, develop a single equation for b(t) in terms of a, A, M, h,
ρ
, g, and R.
d) Determine for b(t) in terms of a, A, M, ho,
ρ
, g, R, and t. [Hint: use db/dt = (db/dh)(dh/dt)]
Solution 4.35. a) Enclose the rolling cart within a moving control volume. The mass inside the
control volume is: M +
ρ
Ah, and
b=b(t)ex
so conservation of mass implies:
d
dt
ρ
dV
V*
∫+
ρ
(u−b)⋅n
A*
∫dA =0→d
dt
M+
ρ
Ah
( )
+
ρ
aUe=0
.
Here flowing water only crosses the control surface at the tube outlet. At this location the water’s
velocity is
u=−Ueex+bex
and the outward normal is:
n=−ex
, so
(u−b)⋅n=−Ueex⋅(−ex)=Ue
.
The area of this outlet is a, so the flux integral is simply +
ρ
aUe. To find the equation for h(t),
complete the indicated differentiation and use Ue = gh/R to reach:
dh dt =−ag AR
( )
h
.
b) This equation has the exponential solution:
h(t)=hoexp −agt AR
{ }
when h(0) = ho.
c) Use the same control volume and note that only horizontal momentum needs to be conserved.
In addition assume that the mass of water in the outlet pipe is negligible compared M +
ρ
hA so
that the horizontal momentum in the control volume is simply (M +
ρ
hA)b. With frictionless
wheels, there is no horizontal force on the control volume, so horizontal conservation of
momemtum implies:
d
dt
ρ
u dV
V*
∫+
ρ
u(u−b)⋅n
A*
∫dA =0→d
dt (M+
ρ
Ah)b
[ ]
+
ρ
a(–Ue+b)Ue=0
.
Expand the derivative & flux term to find:
(M+
ρ
Ah)db
dt +bd
dt (M+
ρ
Ah)+
ρ
aUe
!
“
#$
%
&−
ρ
aUe
2=0
.
Cons. of mass requires the terms in [,]-brackets to be zero, so this equation simplifies to:
x!
y !
h(t)!
Ue!
b(t)!
g!
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.36. Prove that the stress tensor is symmetric by considering first order changes in
surface forces on a vanishingly small cube in rotational equilibrium. Work with rotation about
the no. 3 coordinate axis to show T12 = T21. Cyclic permutation of the indices will suffice for
showing the symmetry of the other two shear stresses.
Solution 4.36. Consider a small cubical volume dV = dx1dx2dx3. All of the surface tractions
lying in the x1–x2 plane act to rotate or counter rotate this cube around the x3 axis. The horizontal
and vertical moment arms from cube’s center to each side are dx1/2 or dx2/2, respectively.
Rotational equilibrium about an axis through the cube’s center parallel to the x3 axis requires:
+Y
12 +
∂
T
12
∂
x1
dx1
2
!
“
#$
%
&dx2dx3
( )
dx1
2+T
12 −
∂
T
12
∂
x1
dx1
2
!
“
#$
%
&dx2dx3
( )
dx1
2
−T21 +
∂
T21
∂
x2
dx2
2
“
#
$%
&
‘dx1dx3
( )
dx2
2−T21 −
∂
T21
∂
x2
dx2
2
“
#
$%
&
‘dx1dx3
( )
dx2
2
+ higher order terms =
ρ
12
dx1dx2dx3dx1
( )
2+dx2
( )
2
{ }
dΩ
dt
where Ω is the rotation rate. Here, the first 4 terms are written as triple products, (surface
stress)(surface area)(moment arm). The final term is the product of the moment of inertia about
the x3-axis and the rotational acceleration. Canceling terms and dividing by dV produces:
T
12 −T21 =
ρ
12
dx1
( )
2+dx2
( )
2
{ }
dΩ
dt
.
Taking dx1,dx2 → 0, produces
τ
12 =
τ
21. Cyclic permutation of the indices can be used to show
the equality of the other two shear stresses: T23 = T32, and T31 = T13.
x1!
x2!
dx1!
centroid!
axis!
dx2!
T
12 −dx1
2
∂T
12
∂x1
T
12 −dx1
2
∂T
12
∂x1
T21 +dx2
2
∂T21
∂x1
T21 −dx2
2
∂T21
∂x1
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.37. Obtain an empty plastic milk jug with a cap that seals tightly, and a frying pan.
Fill both the pan and jug with water to a depth of approximately 1 cm. Place the jug in the pan
with the cap off. Place the pan on a stove and turn up the heat until the water in the frying pan
boils vigorously for a few minutes. Turn the stove off, and quickly put the cap tightly on the jug.
Avoid spilling or splashing hot water on yourself. Remove the capped jug from the frying pan
and let it cool to room temperature. Does anything interesting happen? If something does
happen, explain your observations in terms of surface forces. What is the origin of these surface
forces? Can you make any quantitative predictions about what happens?
Solution 4.37. When the sealed milk jug is allowed to cool, its volume shrinks. This occurs
because the water vapor inside the jug provides an outward-pushing surface pressure of only 3
kPa at room temperature, while the atmosphere in your kitchen provides an inward-pushing
surface force of ~1 atm (101.3 kPa). The plastic of the milk jug is not strong enough to support
the inward-pushing load, so the jug ends up being “crushed” by atmospheric pressure. This
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.38. In cylindrical coordinates (R,
ϕ
,z), two components of a steady incompressible
viscous flow field are known:
u
ϕ
=0
, and
uz=−Az
where A is a constant, and body force is zero.
a) Determine uR so that the flow field is smooth and conserves mass.
b) If the pressure, p, at the origin of coordinates is Po, determine p(R,
ϕ
,z) when the density is
constant.
Solution 4.38. a) Incompressible flow implies:
∇ ⋅ u=0=1R
( )
∂
RuR
∂
R
( )
+1R
( )
∂
u
ϕ∂ϕ
( )
+
∂
uz
∂
z
(in cylindrical coords).
Substitute in the given u
ϕ
and uz to find:
∂
(RuR)
∂
R−RA =0
. Integrate in R to get:
uR=AR 2+B R
.
The constant B must be zero for the flow field to be smooth as
R→0
, so
uR=AR 2
.
b) Convert to Cartesian coordinates. With
u
ϕ
=0
:
u=uRcos
ϕ
=Ax 2
and
v=uRsin
ϕ
=Ay 2
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.39. Consider solid-body rotation of an isothermal perfect gas (with constant R) at
temperature T in (r,
θ
)-plane-polar coordinates: ur = 0, and
u
θ
=Ωzr
, where Ωz is a constant
rotation rate and the body force is zero. What is the pressure distribution p(r) if p(0) = patm? If the
gas is air at 295 K and the container has a radius of ro = 10 cm, what Ωz is needed to produce
p(ro) = 2patm? Ignore gravity.
Solution 4.39. For steady solid body rotation, the unsteady fluid acceleration will be zero and the
viscous stress in the fluid will be zero. In plane polar coordinates, the radial momentum equation
is under these conditions is
−u
θ
2
r=−1
ρ
∂
p
∂
r
.
(see Appendix B.6). Here, both
ρ
and p can vary, but the flow is presumed isothermal so
ρ
=
p/RT. Thus, with
u
θ
=Ωzr
, the steady radial momentum equation is:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.40. Solid body rotation with a constant angular velocity, Ω, is described by the
following Cartesian velocity field:
u=Ω×x
. For this velocity field:
a) Compute the components of:
Tij =−p
δ
ij +
µ∂
ui
∂
xj
+
∂
uj
∂
xi
“
#
$
$
%
&
‘
‘−2
3
δ
ij
∂
uk
∂
xk
(
)
*
*
+
,
–
–+
µυδ
ij
∂
uk
∂
xk
.
b) Consider the case of
Ω1=Ω2=0
,
Ω3≠0
, with p = po at x1 = x2 = 0. Use the differential
momentum equation in Cartesian coordinates to determine p(r), where
r2=x1
2+x2
2
, when there
is no body force and
ρ
= constant. Does your answer make sense? Can you check it with a
simple experiment?
Solution 4.40. a) The components of the velocity field
u=Ω×x
are
u1=Ω2x3− Ω3x2
,
u2=Ω3x1− Ω1x3
, and
u3=Ω1x2− Ω2x1
. The strain rate tensor is for this velocity field is:
Sij =1
2
∂
ui
∂
xj
+
∂
uj
∂
xi
#
$
%
%
&
‘
(
( =1
2
2
∂
u1
∂
x1
∂
u1
∂
x2
+
∂
u2
∂
x1
∂
u1
∂
x3
+
∂
u3
∂
x1
∂
u2
∂
x1
+
∂
u1
∂
x2
2
∂
u2
∂
x2
∂
u2
∂
x3
+
∂
u3
∂
x2
∂
u3
∂
x1
+
∂
u1
∂
x3
∂
u3
∂
x2
+
∂
u2
∂
x3
2
∂
u3
∂
x3
)
*
+
+
+
,
+
+
+
–
.
+
+
+
/
+
+
+
=
0+Ω3+Ω2− Ω2
+Ω3− Ω30−Ω1+Ω1
−Ω2+Ω2+Ω1− Ω10
)
*
+
,
+
–
.
+
/
+
=
0 0 0
0 0 0
0 0 0
“
#
$
%
$
&
‘
$
(
$
,
and this also shows that ∂uk/∂xk = 0. Thus, all the viscous stress components of Tij are zero, so
Tij = –p
δ
ij.
b) When
Ω1=Ω2=0
, with
Ω3
= const., the fluid velocity is
u=Ω3e
ϕ
in cylindrical coordinates
with x3 = z. In spite of this simplification, it’s even simpler to continue with Cartesian
coordinates. The switch to cylindrical coordinates can be made at the end. Use Cauchy’s form
of the momentum equation (4.24)
∂
uj
∂
t+ui
∂
uj
∂
xi
=gj+1
ρ
∂
Tij
∂
xi
.
The flow is steady,
∂
uj
∂
t=0
, and there’s no body force, gj = 0. Combine these facts with the
result of part a) to find:
ui
∂
uj
∂
xi
=−1
ρ
∂
p
∂
xj
.
Evaluate the left side of this equation using the given velocity field:
ρ
−Ω3x2e1+Ω3xe2
( )
⋅e1
∂
∂
x1
+e2
∂
∂
x2
‘
(
)
*
+
, −Ω3x2e1+Ω3x1e2
( )
=−e1
∂
p
∂
x1
−e2
∂
p
∂
x2
.
This reduces to:
−
ρ
Ω3
2x1e1−
ρ
Ω3
2x2e2=e1
∂
p
∂
x1
−e2
∂
p
∂
x2
−e3
∂
p
∂
x3
.
Considering each component separately along with the boundary condition on x1 = x2 = 0, yields:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.41. Using only (4.7), (4.22), (4.36), and (3.12) show that
ρ
Du
Dt +∇p=
ρ
g+
µ
∇2u+
µυ
+1
3
µ
( )
∇ ∇ ⋅ u
( )
when the dynamic (
µ
) and bulk (
µυ
) viscosities are constants.
Solution 4.41. Start by expanding the left side of the momentum equation (4.22), then regroup
the terms.
∂
∂
t
ρ
uj
( )
+
∂
∂
xi
ρ
uiuj
( )
=
ρ∂
uj
∂
t+uj
∂ρ
∂
t+
ρ
ui
∂
uj
∂
xi
+uj
∂
∂
xi
ρ
ui
( )
,
=
ρ∂
uj
∂
t+
ρ
ui
∂
uj
∂
xi
+uj
∂ρ
∂
t+
∂
∂
xi
ρ
ui
( )
$
&
‘
) =
ρ∂
uj
∂
t+
ρ
ui
∂
uj
∂
xi
With the summation convention and the definition of the Kronecker
δ
-function,
∂
∂
xi
( )
δ
ij
[ ]
=
δ
ij
∂
( )
∂
xi
=
∂
( )
∂
xj
where ( ) represents any variable or combination of variables. Using this and distributing
∂ ∂
xi
over the terms in [,]-braces yields:
∂τ
ij
∂
xi
=−
∂
p
∂
xj
+
∂
∂
xi
µ∂
ui
∂
xj
+
∂
uj
∂
xi
%
&
‘
‘
(
)
*
*
+
,
–
–
.
/
0
0
+
∂
∂
xj
µυ
−2
3
µ
( )
∂
uk
∂
xk
+
,
–
.
/
0
.
Combining the modified forms of the right and left sides of (4.22) leads to:
ρ∂
uj
∂
t+
ρ
ui
∂
uj
∂
xi
=
ρ
gj−
∂
p
∂
xj
+
∂
∂
xi
µ∂
ui
∂
xj
+
∂
uj
∂
xi
%
&
‘
‘
(
)
*
*
+
,
–
–
.
/
0
0
+
∂
∂
xj
µυ
−2
3
µ
( )
∂
uk
∂
xk
+
,
–
.
/
0
.
When the dynamic viscosity µ and the bulk viscosity
µυ
are constants; they can be brought
outside the
∂ ∂
xi
-differentiations, i.e.
To convert from index to vector notation, the following definitions and replacements apply:
uj=u
,
gj=g
,
∂
∂
xj
=∇
,
∂
∂
xi
∂
∂
xi
#
$
%
&
‘
( =∇2
,
∂
∂
xj
∂
∂
xi
#
$
%
&
‘
( =
∂
∂
xi
∂
∂
xj
#
$
%
%
&
‘
(
( =∇∇
(no dot product), and
∂
∂
t+ui
∂
∂
xi
=D
Dt
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling