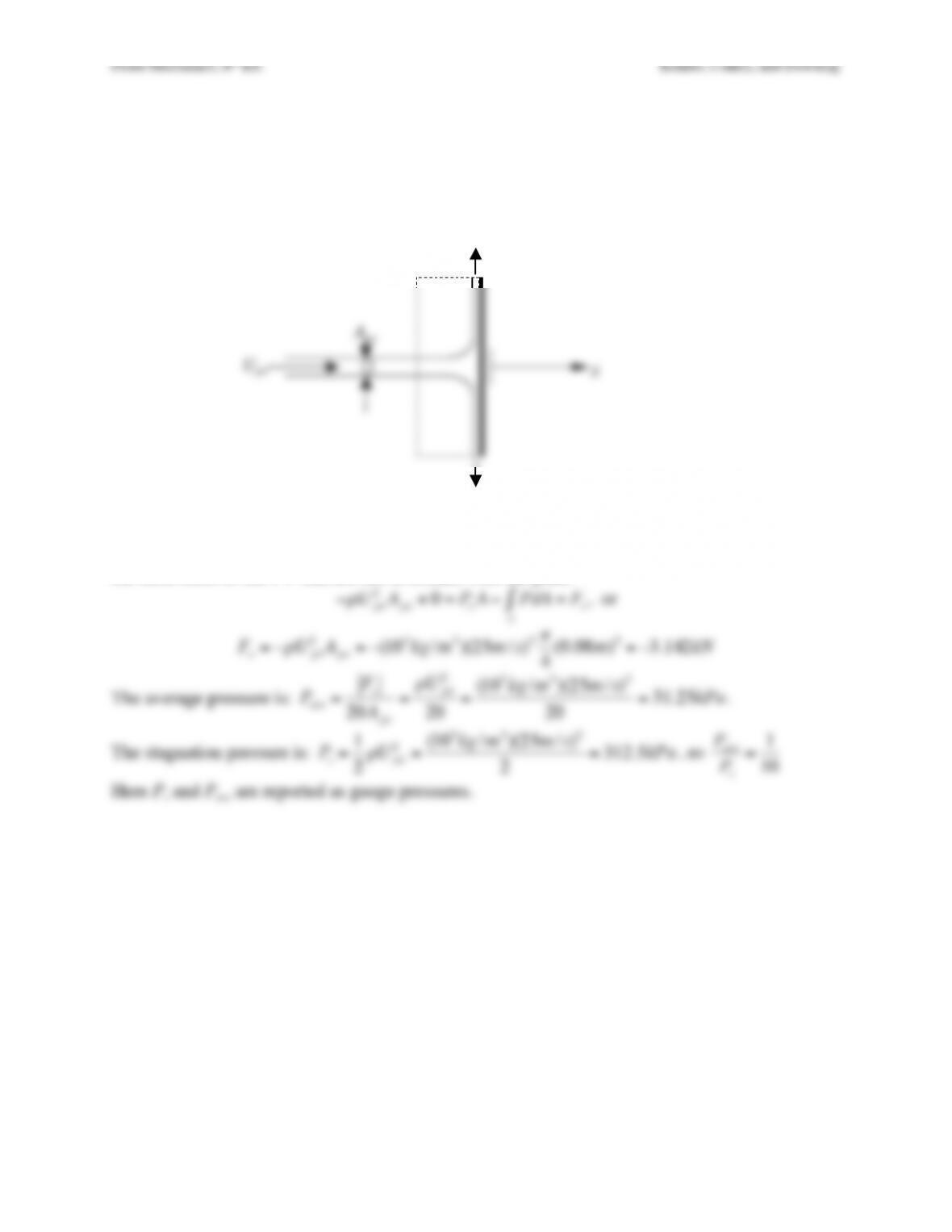
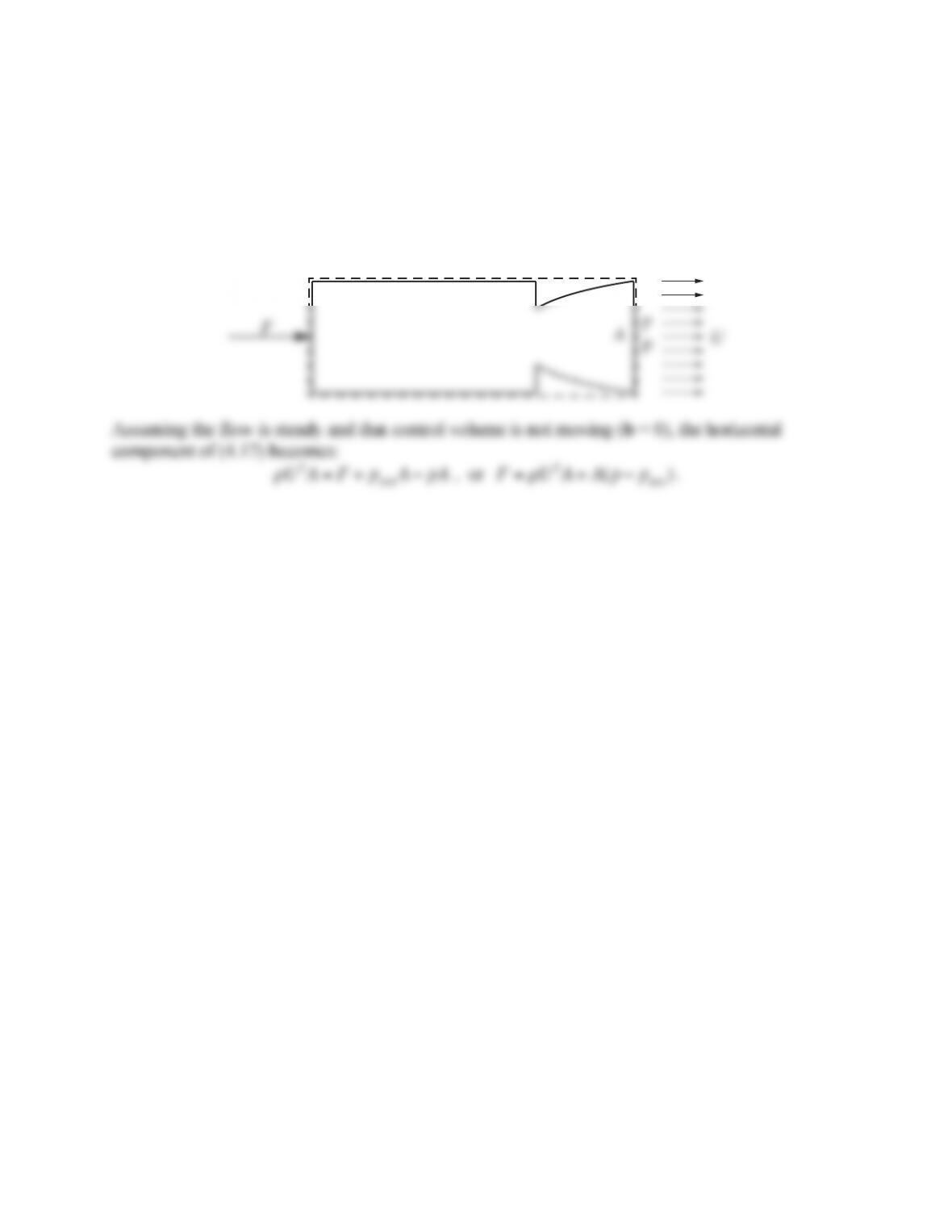
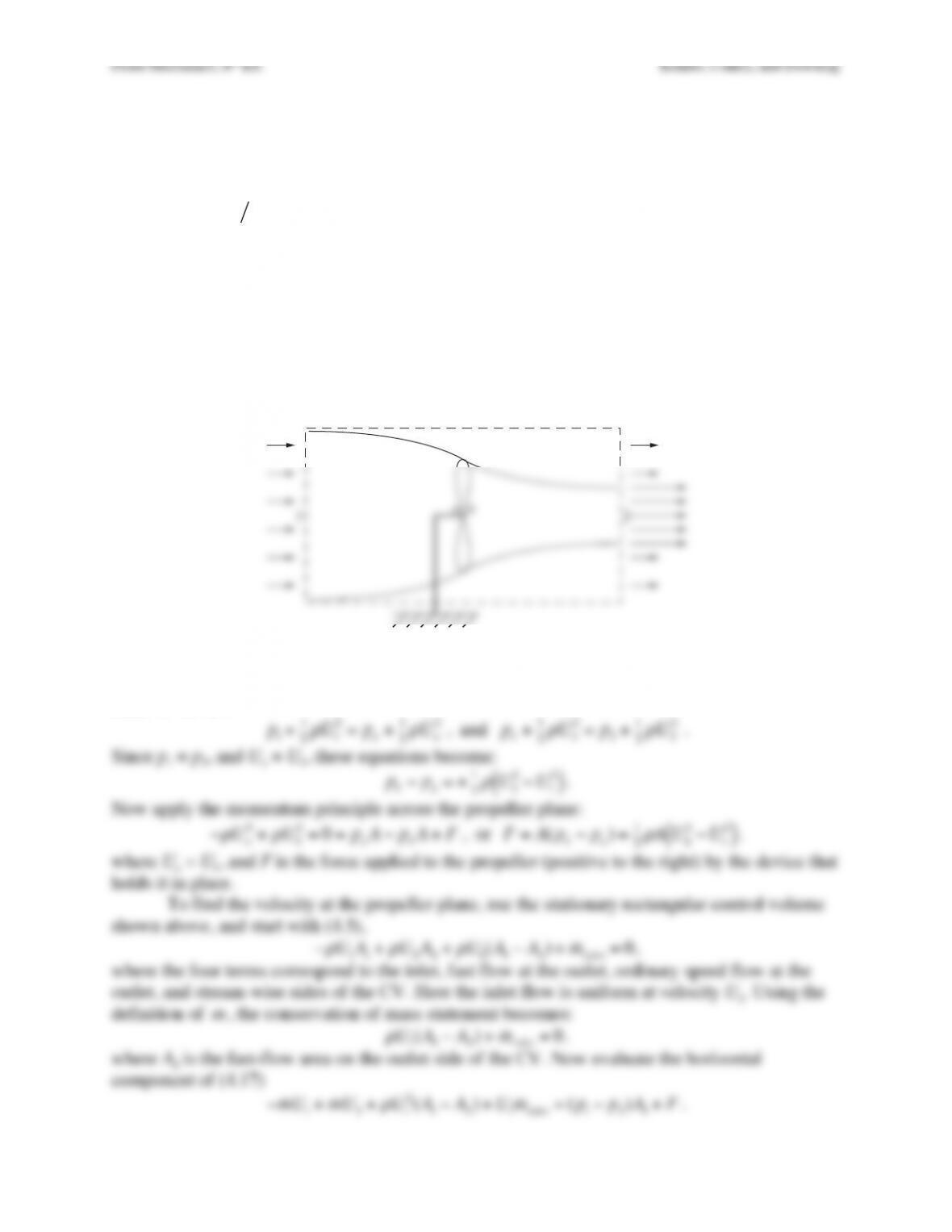
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.1. Let a one-dimensional velocity field be u = u(x, t), with v = 0 and w = 0. The
density varies as
ρ
=
ρ
0(2 − cos
ω
t). Find an expression for u(x, t) if u(0, t) = U.
Solution 4.1. Here u = u(x,t)ex, and the density field is given, so a solution for u(x,t) might be
found from the continuity equation:
∂ρ
∂
t+∇ ⋅
ρ
u
( )
=0
, or specifically for this problem:
∂ρ
∂
t+u
∂ρ
∂
x+
ρ∂
u
∂
x=0
.
The given density field only depends on time so ∂
ρ
/∂x = 0, and this leads to:
∂
u
∂
x=−1
ρ
∂ρ
∂
t=−
ρ
0sin(
ω
t)
ρ
02−cos(
ω
t)
( )
→u=−sin(
ω
t)
2−cos(
ω
t)
‘
(
) *
+
,
x+C(y,z,t)
.
where C is function of integration that does not depend on x. The initial condition requires:
u(0, t) = U = C(y,z,t),
so the final answer for u(x, t) is
u=U−sin(
ω
t)
2−cos(
ω
t)
$
%
& ‘
(
)
x
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.2. Consider the one-dimensional Cartesian velocity field:
u=
α
x t, 0, 0
( )
where
α
is a
constant.
a) Find a spatially uniform, time-dependent density field,
ρ
=
ρ
(t), that renders this flow field
mass conserving when
ρ
=
ρ
o at t = to.
b) What are the unsteady (∂u/∂t), advective (
[u⋅ ∇]u
), and particle (Du/Dt) accelerations in this
flow field? What does
α
= 1 imply?
Solution 4.2. a) Use the continuity equation and the given velocity field with
ρ
=
ρ
(t):
∂ρ
∂
t+∇ ⋅
ρ
u
( )
=0
implies
d
ρ
dt +
ρα
t=0
.
Separate variables and integrate:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.3. Find a non-zero density field
ρ
(x,y,z,t) that renders the following Cartesian velocity
fields mass conserving. Comment on the physical significance and uniqueness of your solutions.
a)
u=Usin(
ω
t−kx),0,0
( )
where U,
ω
, k are positive constants.
[Hint: exchange the independent variables x,t for a single independent variable
ξ
=
ω
t–kx]
b)
u=(−Ωy,+Ωx,0)
with Ω = constant. [Hint: switch to cylindrical coordinates]
c)
u=A x,B y ,C z
( )
where A, B, C are constants.
Solution 4.3. a)
u=Usin(
ω
t−kx),0,0
( )
where U,
ω
, k are positive constants. Use the expanded
form of the continuity equation for a unidirectional velocity,
u=u,0,0
( )
:
∂ρ
∂
t+
ρ∂
u
∂
x+u
∂ρ
∂
x=0
,
and plug in the given velocity field to find:
∂ρ
∂
t−
ρ
kU cos(
ω
t−kx)+Usin(
ω
t−kx)
∂ρ
∂
x=0
.
Now follow the hint and change from independent variables (x, t) to
ξ
, where
ξ
=
ω
t–kx:
∂
∂
t=
∂ξ
∂
t
d
d
ξ
=
ω
d
d
ξ
, and
∂
∂
x=
∂ξ
∂
x
d
d
ξ
=−kd
d
ξ
,
to find a first-order differential equation:
ω
d
ρ
d
ξ
−kU sin
ξ
d
ρ
d
ξ
=
ρ
kU cos
ξ
that can be
separated and integrated:
d
ρ
ρ
∫=kU cos
ξ
ω
−kU sin
ξ
∫d
ξ
–>
ln
ρ
=−ln
ω
−kU sin
ξ
( )
+C(y,z)
.
Here, the constant C must be used to make the solution dimensionally sound. Noting that
ω
/k
has units of velocity, define M = kU/
ω
. Since the original equation did not contain any y or z
dependence the constant of integration might depend on these variables. So, the final solution
can be obtained by exponentiating the last equation:
ρ
(x,y,z,t)=
ρ
o(y,z)
1−Msin
ξ
=
ρ
o(y,z)
1−kU
ω
( )
sin(
ω
t−kx)
,
where
ρ
o(y,z) is an undetermined function. Thus, this solution is not fully determined; it is not
unique. This is the density field that corresponds to a traveling-wave disturbance in a stationary
medium. In the limit as M → 0 with
ρ
o = constant, this wave becomes an acoustic plane wave
with
ω
/k = the speed of sound and M = the Mach number of the fluid particle motions.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Comparing this equation with the one above shows that the characteristic paths are defined by
dR dt =0
,
d
ϕ
dt =Ω
, and
dz dt =0
. Thus, after a single time integration of each equation, the
characteristic paths are determined to be:
R=Ro
,
ϕ
=Ωt+
ϕ
o
, and
z=zo
.
where the quantities with subscript zero are constants. Along these paths the original equation
for
ρ
becomes d
ρ
/dt = 0, which implies the density is constant along these paths. If the density
at Ro,
ϕ
o, zo and t = 0 is
ρ
o(Ro,
ϕ
o,zo)
, then the density at all later times can be obtained by
substituting for ro,
θ
o, zo from the three equations that specify the characteristic path. Hence, the
solution for the density is:
ρ
=
ρ
o(R,
ϕ
− Ωt,z)
.
Here again
ρ
o is an undetermined function so this solution is not fully determined; it is
not unique. In this case, the velocity field corresponds to solid-body rotation about the z-axis at
angular rate Ω, so conservation of mass implies that the density variations must revolve around
the z-axis at the angular rate Ω as well.
c)
u=A x,B y ,C z
( )
where A, B, C are constants.
Use the expanded form of the continuity equation:
∂ρ
∂
t+
ρ
∇ ⋅ u+u⋅ ∇
ρ
=0
, and plug in the given
velocity field to find:
∂ρ
ρ
A
“
$%
‘+A
∂ρ
∂ρ
∂ρ
. This equation is linear in
where ( )´ denotes derivative of ( ) with respect to is argument. Because each group of terms
depends on only one of the independent coordinates, this equation can only be satisfied if each
term is equal to a constant, and the 4 constants sum to zero. This means setting:
“
T
T=d
,
A
x
“
X
X−A
x2=a
,
B
y
“
Y
Y−B
y2=b
, and
C
z
“
Z
Z−C
z2=c
where a + b + c + d = 0
The solution of the first equation is:
T(t)=Toedt
where To is a constant, while that of the second
can be found from:
“
X
X=ax
A+1
x→ln X=ax 2
2A+ln x+const.
, or
X(x)=Xoxexp ax 2
2A
“
#
$
%
&
‘
,
where Xo is a constant. The solutions of the third and fourth equations are similar to that of the
second. Combining the solutions of these equations and condensing the leading product of
constants to
C1=ToXoYoZo
produces:
ρ
(x,y,z,t)=C1xyzexp ax2
2A+by2
2B+cz2
2C−(a+b+c)t
$
%
&
‘
(
)
where C1, a, b, c are undetermined constants, and the above restriction on a, b, c, and d has been
used to eliminate d. Any particular version of this solution is acceptable as long as C1 ≠ 0. Here,
the density field is zero everywhere that
∇ ⋅ u→ ∞
. The constants C1, a, b, and c are not
determined so again this solution is not fully determined; it is not unique.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.4. A proposed conservation law for
ξ
, a new fluid property, takes the following form:
d
dt
ρξ
dV
V(t)
∫+Q⋅ndS
A(t)
∫=0
, where V(t) is a material volume that moves with the fluid velocity
u, A(t) is the surface of V(t),
ρ
is the fluid density, and
Q=−
ργ
∇
ξ
.
a) What partial differential equation is implied by the above conservation statement?
b) Use the part-a) result and the continuity equation to show:
∂ξ
∂
t+u⋅ ∇
ξ
=1
ρ
∇ ⋅
ργ
∇
ξ
( )
.
Solution 4.4. a) Start with the given material CV equation, and apply Reynolds Transport Thm.
to the first term on the left side and Gauss’s Divergence Thm. to the other term:
d
dt
ρξ
dV
V(t)
∫+Q⋅ndS
S(t)
∫=0→
∂
∂
t
ρξ
( )
dV
V(t)
∫+
ρξ
u⋅n
( )
dV
A(t)
∫+∇ ⋅ QdV
V(t)
∫=0
.
Now apply Gauss’s divergence theorem to the remaining surface integral, subsitute in the
specified relationship
Q=−
ργ
∇
ξ
, and combine all the terms into one volume integral to find:
∂
∂
t
ρξ
( )
+∇⋅
ρξ
u
( )
− ∇⋅
ργ
∇
ξ
( )
$
%
&
‘
(
)dV
V(t)
∫=0
.
Here V(t) is an arbitrary material volume, so the integrand must be zero. Thus, the partial
differential equation implied by the given CV equation is:
∂
∂
t
ρξ
( )
+∇ ⋅
ρξ
u
( )
=∇ ⋅
ργ
∇
ξ
( )
.
b) Expand the left side of the part a) result and group the terms that have
ξ
as a coefficient:
ρ∂ξ
∂
t+
ξ∂ρ
∂
t+
ξ
∇⋅
ρ
u
( )
+
ρ
u⋅ ∇
ξ
=
ρ∂ξ
∂
t+
ρ
u⋅ ∇
ξ
+
ξ∂ρ
∂
t+∇⋅
ρ
u
( )
#
$
%&
‘
(
.
The contents of the parentheses is zero because of the continuity equation, so dividing by
ρ
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.5. The components of a mass flow vector
ρ
u are
ρ
u = 4x2y,
ρ
v = xyz,
ρ
w = yz2.
a) Compute the net mass outflow through the closed surface formed by the planes x = 0, x = 1, y
= 0, y = 1, z = 0, z = 1.
b) Compute
∇ ⋅ (
ρ
u)
and integrate over the volume bounded by the surface defined in part a)
c) Explain why the results for parts a) and b) should be equal or unequal.
Solution 4.5. a) The specified volume is a cube, and the mass outflow will have six contributions
(one for each side):
ρ
u⋅ndA =
cube surface
∫∫ 4x2y
[ ]
x=1dydz −
z=0
1
∫
y=0
1
∫4x2y
[ ]
x=0dydz
z=0
1
∫
y=0
1
∫
+ xyz
[ ]
y=1dxdz −
z=0
1
∫
x=0
1
∫xyz
[ ]
y=0dxdz
z=0
1
∫
x=0
1
∫
+ yz2
[ ]
z=1dxdy −
y=0
1
∫
x=0
1
∫yz2
[ ]
z=0dxdy
y=0
1
∫
x=0
1
∫
The integral evaluations are straightforward and unremarkable, and lead to:
ρ
u⋅ndA =
cube surface
∫∫ 2+0+1
4+0+1
2+0=11
4
.
b) First compute the divergence of the mass flow:
∇ ⋅ (
ρ
u)=
∂
∂
x
ρ
u
( )
+
∂
∂
y
ρ
v
( )
+
∂
∂
z
ρ
w
( )
=8xy +xz +2yz
,
then integrate it in the cubical volume:
∇ ⋅ (
ρ
u)
cube
∫∫∫ dV =8xy +xz +2yz
( )
dxdydz
z=0
1
∫
y=0
1
∫
x=0
1
∫=81
2
&
‘
( )
*
+ 1
2
&
‘
( )
*
+ +1
2
&
‘
( )
*
+ 1
2
&
‘
( )
*
+ +21
2
&
‘
( )
*
+ 1
2
&
‘
( )
*
+ =11
4
.
c) The two answers should be the same because of Gauss’ Divergence Theorem.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.6. Consider a simple fluid mechanical model for the atmosphere of an ideal spherical
star that has a surface gas density of
ρ
o and a radius ro. The escape velocity from the surface of
the star is ve. Assume that a tenuous gas leaves the star’s surface radially at speed vo uniformly
over the star’s surface. Use the steady continuity equation for the gas density
ρ
and fluid velocity
u
=(ur,u
θ
,u
ϕ
)
in spherical coordinates
1
r2
∂
∂
r
r2
ρ
ur
( )
+1
rsin
θ
∂
∂θ ρ
u
θ
sin
θ
( )
+1
rsin
θ
∂
∂ϕ ρ
u
ϕ
( )
=0
for the following items
a) Determine
ρ
when
vo≥ve
so that u
=(ur,u
θ
,u
ϕ
)=vo1−ve
2vo
2
( )
1−r
or
( )
( )
, 0, 0
( )
.
b) Simplify the result from part a) when
vo>> ve
so that: u
=(ur,u
θ
,u
ϕ
)=(vo, 0, 0)
.
c) Simplify the result from part a) when
vo=ve
.
d) Use words, sketches, or equations to describe what happens when
vo<ve
. State any
assumptions that you make.
Solution 4.6. a) ur is the only non-zero velocity component so only the continuity equation
simplifies to
1
r2
∂
∂
r
r2
ρ
ur
( )
=0
,
multiply by r2 and integrate to find:
r2
ρ
ur=C(
θ
,
ϕ
)
, where C is the function of integration that
cannot depend on r. Thus,
ρ
=C(
θ
,
ϕ
)
r
2
u
r
=
ρ
o
r
o
2
r
2
1−v
e
2
v
o
2
( )
1−r
o
r
( )
( )
[ ]
−1 2
,
where
C(
θ
,
ϕ
)=
ρ
or
o
2vo
= const. is determined from the boundary conditions:
ρ
=
ρ
o & v = vo at r
= ro.
b) When
vo>> ve
, the square root factor in the result of part a) becomes unity, so
ρ
=
ρ
or
o
2r2
.
c) When
vo=ve
, the square root factor simplifies to
r
or
( )
−1 2
, so
ρ
=
ρ
or
or
[ ]
3 2
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.7. Consider the three-dimensional flow field
ui=
β
xi
or equivalently u =
β
rer, where
β
is a constant with units of inverse time, xi is the position vector from the origin, r is the
distance from the origin, and
ˆ
er
is the radial unit vector. Find a density field
ρ
that conserves
mass when:
a)
ρ
(t) depends only on time t and
ρ
=
ρ
o at t = 0, and
b)
ρ
(r) depends only on the distance r and
ρ
=
ρ
1 at r = 1 m.
c) Does the sum
ρ
(t) +
ρ
(r) also conserve mass in this flow field? Explain your answer.
Solution 4.7. The full continuity equation is:
∂
ρ
∂t+(u⋅ ∇)
ρ
+
ρ
∇ ⋅ u=0
.
a) When
ρ
depends only on t,
(u⋅ ∇)
ρ
=0
so the cont. equation reduces to:
d
ρ
dt +
ρ
∇ ⋅ u=0
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.8. The definition of the stream function for two-dimensional constant-density flow in
the x–y plane is:
u=−ez× ∇
ψ
, where ez is the unit vector perpendicular to the x–y plane that
determines a right-handed coordinate system.
a) Verify that this vector definition is equivalent to
u=
∂ψ ∂
y
, and
v=−
∂ψ ∂
x
in Cartesian
coordinates.
b) Determine the velocity components in r–
θ
polar coordinates in terms of r–
θ
derivatives of
ψ
.
c) Determine an equation for the z-component of the vorticity in terms of
ψ
.
Solution 4.8. a) Start from the definition of the cross product:
u=−ez× ∇
ψ
=−
exeyez
0 0 1
∂ψ ∂
x
∂ψ ∂
y0
=−ex−
∂ψ
∂
y
‘
(
)
*
+
, −ey
∂ψ
∂
x
‘
(
) *
+
, =ex
∂ψ
∂
y
‘
(
)
*
+
, +ey−
∂ψ
∂
x
‘
(
) *
+
,
.
Setting components equal from the extreme ends of this extended equality produces:
u=
∂ψ ∂
y
, and
v=−
∂ψ ∂
x
.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.9. A curve of
ψ
(x,y)=C1
(= a constant) specifies a streamline in steady two-
dimensional constant-density flow. If a neighboring streamline is specified by
ψ
(x,y)=C2
,
show that the volume flux per unit depth into the page between the streamlines equals C2 – C1
when C2 > C1.
Solution 4.9. Start with a picture of the two streamlines and let Q = volume flow rate (per unit
depth) between them.
By definition,
Q=|u|d
1
2
∫
where
d
is an increment of length that lies perpendicular to the flow
in the stream tube. Again by definition,
∇
ψ
lies in the direction perpendicular to the lines of
constant
ψ
. Thus:
ψ
= C1
ψ
= C2
∇
ψ
d
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.10. Consider steady two-dimensional incompressible flow in r–
θ
polar coordinates
where
u=(ur,u
θ
)
,
ur= + Λr2
( )
cos
θ
, and Λ is positive constant. Ignore gravity.
a) Determine the simplest possible
u
θ
.
b) Show that the simplest stream function for this flow is
ψ
=Λr
( )
sin
θ
.
c) Sketch the streamline pattern. Include arrowheads to show stream direction(s).
d) If the flow is frictionless and the pressure far from the origin is p∞, evaluate the pressure p(r,
θ
) on
θ
= 0 for r > 0 when the fluid density is
ρ
. Does the pressure increase or decrease as r
increases?
Solution 4.10. a) Place the given
ur= + Λr2
( )
cos
θ
into
∇ ⋅ u=1
r
∂
∂r
rur
( )
+1
r
∂u
θ
∂
θ
=0
, to find:
1
r
∂
∂r
rur
( )
=1
r
∂
∂r+Λ
r
cos
θ
#
$
%&
‘
(=−Λ
r3cos
θ
=−1
r
∂u
θ
∂
θ
, or
∂u
θ
∂
θ
= + Λ
r2cos
θ
→u
θ
= + Λ
r2sin
θ
,
where the final constant of integration has been dropped to produce the simplest possible
u
θ
.
b) By definition:
ur=1r
( )
∂
ψ
∂
θ
( )
, and
u
θ
=−∂
ψ
∂r
. Thus,
1
r
∂
ψ
∂
θ
= + Λ
r2cos
θ
so
∂
ψ
∂
θ
= + Λ
rcos
θ
→
ψ
=Λ
rsin
θ
+f(r)
, and
−∂
ψ
∂r= + Λ
r2sin
θ
so
ψ
=Λ
rsin
θ
+g(
θ
)
.
The simplest stream function is recovered when f = g = 0:
ψ
=Λr
( )
sin
θ
.
c) Start from the result of b) and switch to Cartesian coordinates:
positive when
θ
is near zero.
d) Use the ordinary Bernoulli-equation to find:
p∞=p(r,
θ
)
θ
=0+1
2
ρ
vr
2+v
θ
2
( )
θ
=0=p(r, 0) +1
2
ρ
Λ2
r4cos2(0) +Λ2
r4sin2(0)
#
$
%&
‘
(
, or
p(r, 0) =p∞−1
2
ρ
Λ2
r4
.
The pressure increases as r increases.
y!
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.11. The well-known undergraduate fluid mechanics textbook by Fox et al. (2009)
provides the following statement of conservation of momentum for a constant-shape (non-
rotating) control volume moving at a non-constant velocity U = U(t).
d
dt
ρ
urel dV
V*(t)
∫+
ρ
urel urel ⋅n
( )
A*(t)
∫dA =
ρ
gdV
V*(t)
∫+fdA
A*(t)
∫−
ρ
dU
dt
dV
V*(t)
∫
.
Here
urel =u−U(t)
is the fluid velocity observed in a frame of reference moving with the
control volume while u and U are observed in a non-moving frame. Meanwhile, (4.17) states
this law as
d
dt
ρ
udV
V*(t)
∫+
ρ
u u −U
( )
⋅n
A*(t)
∫dA =
ρ
gdV
V*(t)
∫+fdA
A*(t)
∫
where the replacement b = U has been made for the velocity of the accelerating control surface
A*(t). Given that the two equations above are not identical, determine if these two statements of
conservation of fluid momentum are contradictory or consistent.
Solution 4.11. The goal here is to derive one result from the other. Start with the equation from
the undergraduate textbook, substitute using
urel =u−U
, and put the forces on the left and move
the extra acceleration term to the other side of the equation.
ρ
gdV
V*(t)
∫+fdA
A*(t)
∫=
ρ
dU
dt
dV
V*(t)
∫+d
dt
ρ
u−U
( )
dV
V*(t)
∫+
ρ
u−U
( )
u−U
( )
⋅n
A*(t)
∫dA
Now expand the terms on the terms on the right side.
ρ
gdV
V*(t)
∫+fdA
A*(t)
∫=
ρ
dU
dt
dV
V*(t)
∫+d
dt
ρ
udV
V*(t)
∫−dU
dt
ρ
dV
V*(t)
∫−Ud
dt
ρ
dV
V*(t)
∫+
ρ
u u −U
( )
⋅n
A*(t)
∫dA −U
ρ
u−U
( )
⋅n
A*(t)
∫dA
Here, U and dU/dt depend on time only – that is, they are uniform over V*(t) and A*(t) – so they
can move inside or outside of the volume and surface integrals over V*(t) and A*(t), but not
through time differentiations. Thus, the first and third terms on the right side are equal and
opposite. Rearrange the other terms to find:
ρ
gdV
V*(t)
∫+fdA
A*(t)
∫=d
dt
ρ
udV
V*(t)
∫+
ρ
u u −U
( )
⋅n
A*(t)
∫dA −Ud
dt
ρ
dV
V*(t)
∫+
ρ
u−U
( )
⋅n
A*(t)
∫dA
&
‘
(
)
*
+
.
Conservation of mass requires the contents of the [,]-brackets to equal zero. After removing
these terms, the remaining simplified equation,
d
dt
ρ
udV
V*(t)
∫+
ρ
u u −U
( )
⋅n
A*(t)
∫dA =
ρ
gdV
V*(t)
∫+fdA
A*(t)
∫
,
is the same as (4.17). The two statements of conservation of momentum are consistent. The
essential difference between the two CV formulations of conservation of momentum amounts to
a transformation between an inertial and an accelerating frame of reference when mass is also
conserved.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.12. A jet of water with a diameter of 8 cm and a speed of 25 m/s impinges normally
on a large stationary flat plate. Find the force required to hold the plate stationary. Compare the
average pressure on the plate with the stagnation pressure if the plate is 20 times the area of the
jet.
Solution 4.12. Choose a rectangular CV with one side that covers and the flat stationary plate.
Denote the plate area by A, and the jet velocity & area by Ujet and Ajet, respectively. In this
problem only the x-component of the integral momentum equation is required, and x-direction
fluid momentum only enters the CV of its left side. Assume that atmospheric pressure Po acts on
the three sides of the CV that are not in contact with the plate.
−
ρ
Ujet
2Ajet +0=PoA−PdA
A
∫=Fx
, or
Fx=−
ρ
Ujet
2Ajet =−(103kg /m3)(25m/s)2
π
4(0.08m)2=−3.142kN
The average pressure is:
Pave =Fx
20Ajet
=
ρ
Ujet
2
20 =(103kg /m3)(25m/s)2
20 =31.25kPa
.
The stagnation pressure is:
Ps=1
2
ρ
Ujet
2=(103kg /m3)(25m/s)2
2=312.5kPa
, so
P
ave
P
s
=1
10
Here Ps and Pave are reported as gauge pressures.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.13. Show that the thrust developed by a stationary rocket motor is F =
ρ
AU2 + A(p −
patm), where patm is the atmospheric pressure, and p,
ρ
, A, and U are, respectively, the pressure,
density, area, and velocity of the fluid at the nozzle exit.
Solution 4.13. Use the control volume shown, where F is the force that holds the rocket motor in
place.
F
patm
U
p
!
A
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.14. Consider the propeller of an airplane moving with a velocity U1. Take a reference
frame in which the air is moving and the propeller [disk] is stationary. Then the effect of the
propeller is to accelerate the fluid from the upstream value U1 to the downstream value U2 > U1.
Assuming incompressibility, show that the thrust developed by the propeller is given by
F=
ρ
A(U2
2−U1
2) 2,
where A is the projected area of the propeller and
ρ
is the density (assumed
constant). Show also that the velocity of the fluid at the plane of the propeller is the average
value U = (U1 + U2)/2. [Hint: The flow can be idealized by a pressure jump of magnitude Δp =
F/A right at the location of the propeller. Also apply Bernoulli’s equation between a section far
upstream and a section immediately upstream of the propeller. Also apply the Bernoulli equation
between a section immediately downstream of the propeller and a section far downstream. This
will show that
Δp=
ρ
(U2
2−U1
2) / 2
.]
Solution 4.14. The CV and nominal propeller-edge streamlines are:
where the mass flux within the curved propeller-edge streamlines is constant and equal to
˙
m =
ρ
U1A
1=
ρ
U2A2
. Apply the steady constant density Bernoulli equation from “1” to “a”, and
from “b” to “2”:
p1+1
2
ρ
U1
2=pa+1
2
ρ
Ua
2
, and
pb+1
2
ρ
Ub
2=p2+1
2
ρ
U2
2
.
Since p1 = p2, and Ua = Ub, these equations become:
pb−pa= + 1
2
ρ
U2
2−U1
2
( )
.
Now apply the momentum principle across the propeller plane:
−
ρ
Ua
2+
ρ
Ub
2=0=paA−pbA+F
, or
F=A(pb−pa)=1
2
ρ
A U2
2−U1
2
( )
.
where Ua = Ub, and F is the force applied to the propeller (positive to the right) by the device that
holds it in place.
To find the velocity at the propeller plane, use the stationary rectangular control volume
shown above, and start with (4.5),
−
ρ
U1A
1+
ρ
U2A2+
ρ
U1(A
1−A2)+˙
m
sides =0
,
where the four terms correspond to the inlet, fast flow at the outlet, ordinary speed flow at the
outlet, and stream-wise sides of the CV. Here the inlet flow is uniform at velocity U1. Using the
definition of
˙
m
, the conservation of mass statement becomes:
ρ
U1(A
1−A2)+˙
m
sides =0
.
where A2 is the fast-flow area on the outlet side of the CV. Now evaluate the horizontal
component of (4.17)
−˙
m U1+˙
m U2+
ρ
U1
2(A1−A2)+U1˙
m
sides =(p1−p2)A1+F
.
1 2
ab
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.15. Generalize the control volume analysis of Example 4.1 by considering the control
volume geometry shown for steady two-dimensional flow past an arbitrary body in the absence
of body forces. Show that the force the fluid exerts on the body is given by the Euler momentum
integral,
Fj=−
ρ
uiuj−
τ
ij
( )
A1
∫nidA
, and that
0=
ρ
ui
A1
∫nidA
.
Solution 4.15. For steady flow through a stationary control volume (b = 0) and no body force,
(4.5) and (4.17) simplify to:
where the second equality in each case is the same as first after conversion to index notation, and
(2.15) or (4.20b) has been used to write
the surface force f in terms of the stress
tensor
τ
ij.
normal on the combined surface. Thus, n points outward on A1 (shown as n1 in the figure) and
points into the body on A2 (shown as n2 in the figure). Thus, the conservation laws require:
ρ
uini
A1+A2+A3
∫dA =0
and
ρ
ujui−
τ
ij
( )
ni
A1+A2+A3
∫dA =0
.
Now consider the contribution of each surface individually. In general, there are no
simplifications to be made on A1 and all the integrand terms must be retained. The surface A2
coincides with the surface of the solid body, thus
u⋅n
= uini = 0 on A2. This fact eliminated the
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 4.16. The pressure rise
Δp=p2−p1
that occurs for flow through a sudden pipe-cross-
sectional-area expansion can depend on the average upstream flow speed Uave, the upstream pipe
diameter d1, the downstream pipe diameter d2, and the fluid density
ρ
and viscosity
µ
. Here p2 is
the pressure downstream of the expansion where the flow is first fully adjusted to the larger pipe
diameter.
a) Find a dimensionless scaling law for Δp in terms of Uave, d1, d2,
ρ
and
µ
.
b) Simplify the result of part a) for high-Reynolds-number turbulent flow where
µ
does not
matter.
c) Use a control volume analysis to determine Δp in terms of Uave, d1, d2, and
ρ
for the high
Reynolds number limit. [Hints: i) a streamline drawing might help in determining or estimating
the pressure on the vertical surfaces of the area transition, and ii) assume uniform flow profiles
wherever possible.]
d) Compute the ideal flow value for Δp and compare this to the result from part d) for a diameter
ratio of d1/d2 = ½. What fraction of the maximum possible pressure rise does the sudden
expansion achieve?
Solution 4.16. a) This is a dimensional analysis task. Construct the units matrix.
Δp Uave d1 d2
ρ
µ
M 1 0 0 0 1 1
The matrix has rank three so there are 6 – 3 = 3 dimensionless groups. These can be found by
inspection; thus:
Δp
ρ
Uave
2
( )
=f d1d2,
ρ
Uave d1
µ
( )
where f is an undetermined function.
Uave!
d1 !
d2!
p2!
p1!
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Here, the cross-stream momentum equation suggests that p1 acts on the flat surfaces of the area
transition because the flow separates at the area junction and is parallel to the x-direction and this