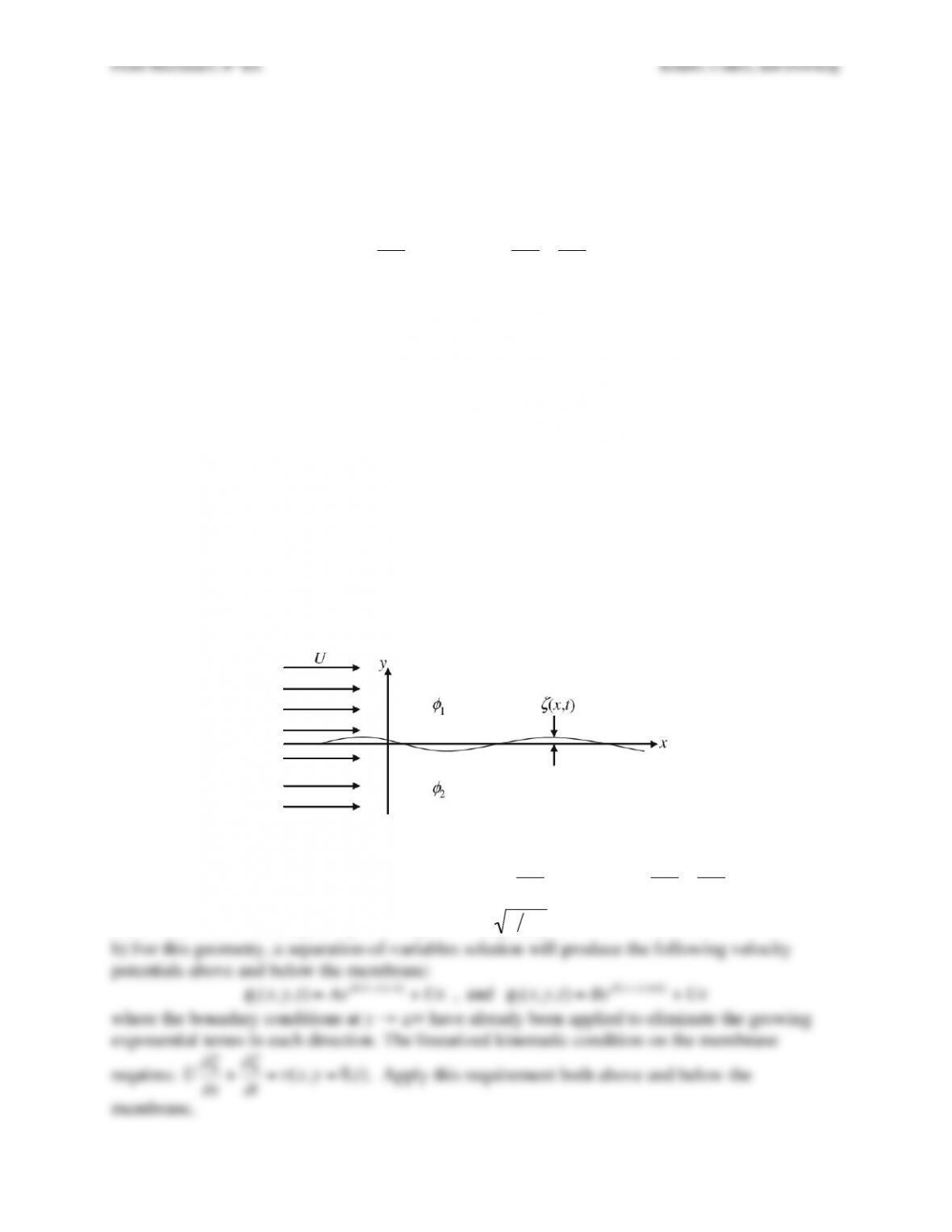
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 11.1. A perturbed vortex sheet nominally located at y = 0 separates flows of differing
density in the presence of gravity with downward acceleration g. The upper stream is semi-
infinite and has density
ρ
1 and horizontal velocity U1. The lower stream has thickness h density
ρ
2, and horizontal velocity U2. A smooth flat impenetrable surface located at y = –h lies below
the second layer. The interfacial tension between the two fluids is
σ
. Assume a disturbance
occurs on the vortex sheet with wave number k = 2π/
λ
, and complex wave speed c, i.e.
y
[ ]
sheet =f(x,t)=foRe eik(x−ct )
{ }
. The four boundary conditions are:
1) u1, v1 → 0 as y → +∞
2) v2 = 0 on y = –h.
3)
u1⋅n
=
u2⋅n
= normal velocity of the vortex sheet on both sides of the vortex sheet.
4)
p1−p2=
σ∂
2f
∂
x2
on the vortex sheet (
σ
= interfacial surface tension)
a) Following the development in Section 11.3, show that:
c=
ρ
1
U1+
ρ
2U2coth(kh)
ρ
1+
ρ
2coth(kh)±(g/k)(
ρ
2−
ρ
1)+
σ
k
ρ
1+
ρ
2coth(kh)−
ρ
1
ρ
2U1−U2
( )
2coth(kh)
ρ
1+
ρ
2coth(kh)
( )
2
%
&
‘
‘
(
)
*
*
1 2
.
b) Use the result of part a) to show that the vortex sheet is unstable when:
tanh(kh)+
ρ
2
ρ
1
#
$
%
&
‘
( g
k
(
ρ
2−
ρ
1)
ρ
2
+
σ
k
ρ
2
#
$
%
&
‘
( <U1−U2
( )
2
c) Will the sheet be stable or unstable to long wavelength disturbances (
k→0
) when
ρ
2 >
ρ
1 for
a fixed velocity difference?
d) Will the sheet be stable or unstable to short wavelength disturbances (
) for a fixed
velocity difference?
e) Will the sheet ever be unstable when U1 = U2?
f) Under what conditions will the thickness h matter?
Solution 11.1. Set up the problem with U1, U2 mean horizontal velocities and u1, u2, v1, and v2,
perturbation velocities due to the small disturbances on the vortex sheet. Above the vortex sheet:
∇2
φ
1=0
and below the vortex sheet:
∇2
φ
2=0
. By inspection or separation of variables:
φ
1=Ae+ky +Be−ky
( )
eik(x−ct )+U1x
(above the vortex sheet)
φ
2=Ce+ky +De−ky
( )
eik(x−ct )+U2x
(below the vortex sheet)
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Po1−
ρ
1
∂φ
1
∂
t−
ρ
1
2U1
2+2U1u1
[ ]
−
ρ
1gf (x,t)=Po2−
ρ
2
∂φ
2
∂
t−
ρ
2
2U2
2+2U2u2
[ ]
−
ρ
2gf (x,t)−k2
σ
f(x,t)
When there is no disturbance on the vortex sheet, the flow is steady and u2 = u1 = 0. Therefore:
P
o1−
ρ
1
2
U1
2=P
o2−
ρ
2
2
U2
2
Subtract this from the full linearized pressure boundary condition and multiply by –1 to get:
ρ
1
∂φ
1
∂
t+
ρ
1
U1u1+
ρ
1gf (x,t)=
ρ
2
∂φ
2
∂
t+
ρ
2U2u2+
ρ
2gf (x,t)+k2
σ
f(x,t)
Now plug in the known forms for f(x,t) and the potentials, evaluate on y = 0 as appropriate, and
cancel common terms:
ρ
1[B(−ikc +ikU1)+gfo]=
ρ
2[2Ce−kh cosh(kh)(−ikc +ikU2)+gfo]+k2
σ
fo
Insert B and C from above, and divide by fo:
ρ
1[k(U1−c)2+g]=
ρ
2[−kcoth(kh)(U2−c)2+g]+k2
σ
.
This is a quadratic for c. Divide by k and put the equation in a more standard form:
ρ
1+
ρ
2coth(kh)
( )
c2−2
ρ
1
U1+
ρ
2U2coth(kh)
( )
c−
σ
k+
ρ
1
U1
2+
ρ
2U2
2coth(kh)+g k
( )
(
ρ
1−
ρ
2)=0
.
Solving for c using the quadratic formula eventually produces:
c=cr+ici=
ρ
1
U1+
ρ
2U2coth(kh)
ρ
1+
ρ
2coth(kh)±g k
( )
(
ρ
2−
ρ
1)+
σ
k
ρ
1+
ρ
2coth(kh)−
ρ
1
ρ
2U1−U2
( )
2coth(kh)
ρ
1+
ρ
2coth(kh)
( )
2
%
&
‘
‘
(
)
*
*
1 2
b) Here we see that ci = 0 (i.e. the right side has no imaginary part) unless the terms inside the
square root together are negative. This means that the vortex sheet is unstable when the terms
inside the [,]-brackets together are negative:
g k
( )
(
ρ
2−
ρ
1)+
σ
k
ρ
1+
ρ
2coth(kh)−
ρ
1
ρ
2U1−U2
( )
2coth(kh)
ρ
1+
ρ
2coth(kh)
( )
2
< 0, or
tanh(kh)+
ρ
2
ρ
1
#
$
%
&
‘
( g
k
(
ρ
2−
ρ
1)
ρ
2
+
σ
k
ρ
2
#
$
%
&
‘
( <U1−U2
( )
2
c) When
ρ
2 >
ρ
1 and
k→0
, the factor with g in it will be positive and unbounded. Thus, the
inequality will be not be satisfied and the sheet will be neutrally stable.
d) When
, the factor with
σ
in it will be positive and unbounded. Thus, the inequality will
be not be satisfied and the sheet will be neutrally stable.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
e) When U1 = U2 = U,
c=U±g k
( )
(
ρ
2−
ρ
1)+
σ
k
ρ
1+
ρ
2coth(kh)
%
&
‘
(
)
*
1 2
, which specifies neutrally stable flow when
g k
( )
(
ρ
2−
ρ
1)+
σ
k>0
, and unstable flow when
g k
( )
(
ρ
2−
ρ
1)+
σ
k<0
. Thus, even when the
fluid densities are not stably arranged, non-zero surface tension prevents high wave number
fluctuations from being unstable. In addition, note that when U1 = U2 =
ρ
1 = 0, we recover the
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 11.2. Consider a fluid layer of depth h and density
ρ
2 lying under a lighter infinitely-
deep fluid of density
ρ
1 <
ρ
2. By setting U1 = U2 = 0, in the results of the Exercise 11.1, the
following formula for the phase speed is found:
c= ± (g/k)(
ρ
2−
ρ
1)+
σ
k
ρ
1+
ρ
2coth(kh)
%
&
‘
(
)
*
1 2
Now invert the sign of gravity and consider why drops form when a liquid is splashed on the
underside of a flat surface. Are long or short waves more unstable? Does a professional painter
want interior ceiling paint with high or low surface tension? For a smooth finish should the
painter apply thin or thick coats of paint? Assuming the liquid has the properties of water
(surface tension ≈ 72 mN/m, density ≈ 103 kg/m) and that the lighter fluid is air, what is the
longest neutrally stable wavelength on the underside of a horizontal surface? [This is the
Rayleigh–Taylor instability and it occurs when density and pressure gradients point in opposite
directions. It may be readily observed by accelerating rapidly downward an upward-open cup of
water.]
Solution 11.2. Changing the sign of gravity in the given formula produces:
c= ± −(g/k)(
ρ
2−
ρ
1)+
σ
k
ρ
1+
ρ
2coth(kh)
%
&
‘
(
)
*
1 2
,
where the symbol “g” is presumed to specify a positive acceleration magnitude. Here, the heavy
liquid with density
ρ
2 is accelerated into the lighter liquid with density
ρ
1, so
ρ
2 –
ρ
1 > 0. Thus,
the first term in the numerator of the fraction under the square root is negative and while the
second is positive. For the wave speed to have a non-zero imaginary component and thereby
indicate instability, the first term, which is negative, must dominate. When k is small (long
In a painting scenario,
ρ
2 corresponds to the paint and
ρ
1 corresponds to the air, so
ρ
2 >>
ρ
1.
Thus, the small-kh formula for c is smaller than the large-kh formula for c, by a factor of [kh]1/2.
This means that instability in a layer of paint on a ceiling develops more slowly when the paint
layer is thin. Thus, a smooth finish is more likely to be obtained via multiple thin coats than one
thick one.
For the given parameters, the longest neutrally stable wavelength will be:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 11.3. Inviscid horizontal flow in the half space y > 0 moves at speed U over a porous
surface located at y = 0. Here the fluid density
ρ
is constant and gravity plays no roll. A weak
vertical velocity fluctuation occurs at the porous surface:
v
[ ]
surface =voRe eik(x−ct )
{ }
, where vo <<
U.
a) The velocity potential for the flow may be written
˜
φ
=Ux +
φ
, where
φ
leads to [v]surface at y = 0
and
φ
vanishes as y → +∞. Determine the perturbation potential
φ
in terms of vo, U,
ρ
, k, c, and
the independent variables (x,y,t).
b) The porous surface responds to pressure fluctuations in the fluid via:
p−ps
[ ]
y=0=−
γ
v
[ ]
surface
,
where p is the pressure in the fluid, ps is the steady static pressure that is felt on the surface when
the vertical velocity fluctuations are absent, and
γ
is a real material parameter that defines the
porous surface’s flow resistance. Determine a formula for c in terms of U,
γ
,
ρ
, and k.
c) What is the propagation velocity, Re{c}, of the surface velocity fluctuation?
d) What sign should
γ
have for the flow to be stable? Interpret your answer.
Solution 11.3. a) To match the porous wall boundary condition, the potential must be of the
following form:
˜
φ
=Ux +
φ
=Ux +F(y)eik(x−ct )
. Setting
∇2
φ
=0
and canceling common factors
produces:
−k2F+# #
F =0
, so that
F=Ae+ky +Be−ky
. Here, A must be zero for |
φ
| = F(y) to vanish
as y → +∞. The second boundary condition implies:
∂φ ∂
y
( )
y=0=v
[ ]
surface =voeik(x−ct )
, or
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 11.4. Repeat exercise 11.3 for a compliant surface nominally lying at y = 0 that is
perturbed from equilibrium by a small surface wave:
y
[ ]
surface =
ζ
(x,t)=
ζ
oRe eik (x−ct )
{ }
.
a) Determine the perturbation potential
φ
in terms of U,
ρ
, k, and c by assuming that
φ
vanishes
as y → +∞, and that there is no flow through the compliant surface. Ignore gravity.
b) The compliant surface responds to pressure fluctuations in the fluid via:
p−ps
[ ]
y=0=−
γζ
(x,t)
,
where p is the pressure in the fluid, ps is the steady pressure that is felt on the surface when the
surface wave is absent, and
γ
is a real material parameter that defines the surface’s compliance.
Determine a formula for c in terms of U,
γ
,
ρ
, and k.
c) What is the propagation velocity, Re{c}, of the surface waves?
d) If
γ
is positive, is the flow stable? Interpret your answer.
Solution 11.4. a) Start with
˜
φ
=Ux +
φ
. The x– and t– dependence in the problem will have to
match that of the surface wave, therefore we must have
˜
φ
=Ux +F(y)eik(x−ct )
, where F(y) must be
determined. Plugging this form for
φ
into
∇2
φ
=0
, produces
−k2F+# #
F =0
after common
factors have been cancelled. This equation has solutions of growing and decaying exponentials
as y increases. The growing exponential is discarded because it is not meaningful as y → +∞.
Thus:
φ
=Ae−kyeik(x−ct )
where A is a constant that can be determined from the linearized kinematic
boundary condition on the surface:
U
∂ζ
∂
x+
∂ζ
∂
t=
∂φ
∂
y
%
&
‘
(
)
*
y=0
→
ikU
ζ
o+−ikc
( )
ζ
o=−kA
, or
A=i c −U
( )
ζ
o
Thus:
φ
=i c −U
( )
ζ
oe−kyeik(x−ct )
b) Without gravity, the Bernoulli equation for this flow is:
ρ∂φ
∂
t+
ρ
2
u2+p=Po
.
Linearize this equation with the perturbation potential to get:
ρ∂φ
∂
t+
ρ
2U2+2U
∂φ
∂
x
%
&
‘ (
)
* +p=Po
.
For flow without the surface perturbation this perturbation Bernoulli equation is:
ρ
2U2+ps=Po
.
Subtract these two Bernoulli equations to find:
p−ps=−
ρ∂φ
∂
t+U
∂φ
∂
x
&
‘
( )
*
+
.
Thus, using the surface’s constitutive relationship, the linearized dynamic boundary condition is:
−
ρ∂φ
∂
t+U
∂φ
∂
x
&
‘
( )
*
+
y=0
=−
γζ
(x,t)
.
Substitute in the result of part a) for
φ
:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
−
ρ
i c −U
( )
−ikc +ikU
( )
ζ
o=−
γζ
o
.
where the common factor of
eik(x−ct )
has been divided out. Reduce this equation to a quadratic
form and solve for c.
c−U
( )
2=
γ
k
ρ
or
c=U±
γ
k
ρ
.
c) When
γ
is positive, the disturbance phase velocity can have two values:
cr=U±
γ
k
ρ
When
γ
is negative, the phase velocity = U.
d) At this point all that can be said is that the flow is neutrally stable when
γ
is positive. When
γ
is positive, a suction pressure on the surface lifts it up, while an elevated pressure pushes it
down. This behavior matches that of gravity. In fact, replacing
γ
in the surface’s constitutive
equation (specified in the statement of part b) by
ρ
g produces:
p−ps=−
ρ
g
ζ
,
which describes a hydrostatic balance, and
cr=U±g
k
,
which are the possible phase speeds of surface waves in deep water that is moving at speed U.
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 11.5. As a simplified version of flag waving, consider the stability of a simple
membrane in a uniform flow. Here, the undisturbed membrane lies in the x–z plane at y = 0, the
flow is parallel to the x-axis at speed U, and the fluid has density
ρ
. The membrane has mass per
unit area =
ρ
m and uniform tension per unit length = T. The membrane satisfies a dynamic
equation based on pressure forces and internal tension combined with its local surface curvature:
ρ
m
∂
2
ζ
∂
t2=p2−p1+T
∂
2
ζ
∂
x2+
∂
2
ζ
∂
z2
&
‘
(
)
*
+
.
Here, the vertical membrane displacement is given by y =
ζ
(x,z,t)
, and p1 and p2 are the
pressures acting on the membrane from above and below, respectively. The velocity potentials
for the undisturbed flow above (1) and below (2) the membrane are
φ
1=
φ
2=Ux
. For the
following items, assume a small amplitude wave is present on the membrane
ζ
(x,t)=
ζ
oRe eik(x−ct )
{ }
with k a real parameter, and assume that all deflections and other
fluctuations are uniform in the z–direction and small enough for the usual linear simplifications.
In addition, assume the static pressures above and below the membrane, in the absence of
membrane motion, are matched.
a) Using the membrane equation, determine the propagation speed of the membrane waves,
Re{c}, in the absence of fluid loading (i.e. when p2 – p1 =
ρ
= 0).
b) Assuming inviscid flow above and below the membrane, determine a formula for c in terms of
T,
ρ
m,
ρ
, U, and k.
c) Is the membrane more or less unstable if U, T,
ρ
, and
ρ
m are individually increased with the
others held constant?
d) What is the propagation speed of the membrane waves when U = 0? Compare this to your
answer for part a) and explain any differences.
Solution 11.5. a) Plug
ζ
(x,t)=
ζ
oRe eik(x−ct
{ }
into
ρ
m
∂
2
ζ
∂
t2=p2−p1+T
∂
2
ζ
∂
x2+
∂
2
ζ
∂
z2
&
‘
(
)
*
+
with p2 – p1
=
ρ
= 0) to find:
−(kc)2
ρ
m
ζ
=T−k2
ζ
+0
( )
, or
c= ± T
ρ
m
.
b) For this geometry, a separation-of-variables solution will produce the following velocity
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
ikU
ζ
o−ikc
ζ
o=−kA
, and
ikU
ζ
o−ikc
ζ
o= +kB
,
to find:
A=−B=i c −U
( )
ζ
o
.
The pressure fluctuation on the membrane is found by linearizing the Bernoulli equation and
subtracting out the part that persists when there is no disturbance on the membrane. This leaves:
“
p
1=p1−ps=−
ρ∂φ
1
∂
t+U“
u
1
‘
(
) *
+
,
, and
“
p
2=p2−ps=−
ρ∂φ
2
∂
t+U“
u
2
‘
(
) *
+
,
,
where ps is the steady pressure on both sides of the membrane when there is no disturbance and
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 11.6. Prove that
σ
r > 0 for the thermal instability discussed in Section 11.4 via the
following steps that include integration by parts and use of the boundary conditions (11.38).
a) Multiply (11.36) by
ˆ
T
*
and integrate the result from z = –1/2 to z = +1/2, where z is the
dimensionless vertical coordinate) to find:
σ
I1+I2=ˆ
T
*Wdz
∫
where
I1≡ˆ
T
2
dz
∫
,
I2≡dˆ
T /dz
2
+K2ˆ
T
2
#
$
%
&
‘
(
dz
∫
, and the limits of the integrations have been suppressed for clarity.
b) Multiply (11.37) by W* and integrate from z = –1/2 to z = +1/2 to find:
σ
Pr
J1+J2=RaK2W*ˆ
T dz
∫
where
J1≡dW /dz 2+K2W2
[ ]
dz
∫
,
J2≡d2W/dz22+2K2dW /dz 2+K4W2
[ ]
dz
∫
, and again the limits of the integrations have been
suppressed.
c) Combine the results of a) and b) to eliminate the mixed integral of W &
ˆ
T
, and use the result
of this combination to show that
σ
i = 0 for Ra > 0. [Note: the integrals I1, I2, J1, and J2 are all
positive definite].
Solution 11.6. a) Equation (11.36) is
σ
+K2−d2
dz2
$
%
&
‘
(
) ˆ
T =W
. As directed, multiply by
ˆ
T
*
and
integrate through the thickness of the layer. The term-by-term results on the left side are:
σ
+K2
( )
ˆ
T
*ˆ
T
∫dz =
σ
+K2
( )
ˆ
T
2
∫dz
, and
−ˆ
T
*d2ˆ
T
dz2
∫dz =−ˆ
T
*dˆ
T
dz
$
%
&
‘
(
)
−1 2
1 2
+dˆ
T
*
dz
dˆ
T
dz
∫dz =dˆ
T
dz
2
∫dz
,
where the boundary terms in the integration by parts are zero because of the boundary conditions
on
ˆ
T
, and all the integrals are from –1/2 to +1/2. Thus, the remnant of (11.36) is:
*Wdz
∫
T
2
dz
T /dz
2
2
#
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
σ
Pr +2K2
#
$
% &
‘
( W*d2W
dz2
∫dz =
σ
Pr +2K2
#
$
% &
‘
( W*dW
dz
*
+
,
–
.
/
−1 2
1 2
−dW *
dz
dW
dz
∫dz
1
2
3
4
5
6
=−
σ
Pr +2K2
#
$
% &
‘
( dW
dz
2
∫dz
,
and
where
J1≡dW /dz 2+K2W2
[ ]
dz
∫
, and
J2≡d2W/dz22+2K2dW /dz 2+K4W2
[ ]
dz
∫
.
c) The complex conjugate of the part a) result is:
σ
*I1+I2=ˆ
T W*dz
∫
,
Use this to eliminate the mixed integral in the result of part b) to find:
σ
Pr
J1+J2=RaK2
σ
*I1+I2
( )
.
The imaginary part of this equation is:
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 11.7. Consider the thermal instability of a fluid confined between two rigid plates, as
discussed in Section 11.4. It was stated there without proof that the minimum critical Rayleigh
number of Racr = 1708 is obtained for the gravest even mode. To verify this, consider the gravest
odd mode for which
W=Asin(q0z)+Bsinh(qz)+Csinh(q*z)
. (1)
(Compare this with the gravest even mode structure: W = Acosq0z + Bcoshqz + Ccoshq*z.)
Following Chandrasekhar (1961, p. 39), show that the minimum Rayleigh number is now
17,610, reached at the wave number Kcr = 5.365.
Solution 11.7. From Section 12.4 the six roots for the vertical wave number q are:
±iqo= ±iK(s−1)1 2
,
±q= ±K1+s1+i3
( )
2
[ ]
1 2
, and
±q*= ±K1+s1−i3
( )
2
[ ]
1 2
, (2)
with
s≡Ra K4
( )
1 3
. The boundary conditions on W are:
W=dW
dz =d2
dz2−K2
#
$
%
&
‘
(
2
W=0
at z = ± 1/2. (3)
From the mode shape specification, equation (1), the first, second, and fourth derivatives of the
mode shape are:
dW
dz =Aq0cos(q0z)+Bqcosh(qz)+Cq*cosh(q*z)
, (4)
d2W
dz2=−Aq0
2sin(q0z)+Bq2sinh(qz)+Cq*2 sinh(q*z)
, and (5)
d4W
dz4=Aq0
4sin(q0z)+Bq4sinh(qz)+Cq*4 sinh(q*z)
. (6)
From (5) and (6),
d2
dz2−K2
#
$
%
&
‘
(
2
W=d4
dz4−2K2d2
dz2+K4
#
$
%
&
‘
(
W
=A q0
2+K2
( )
2sin(q0z)+B q2−K2
( )
2sinh(qz)+C q*2 −K2
( )
2sinh(q*z).
Application of all three boundary conditions (3) at either z = +1/2 or z = –1/2 leads to:
sin(q0/2) sinh(q/z) sinh(q*/2)
q0cos(q0/2) qcosh(q/2) q*cosh(q*/2)
q0
2+K2
( )
2sin(q0/2) q2−K2
( )
2sinh(q/2) q*2 −K2
( )
2sinh(q*/2)
#
$
%
%
%
&
‘
(
(
(
A
B
C
#
$
%
%
%
&
‘
(
(
(
=0
.
For nontrivial solutions, the determinant of the 3×3 matrix has to be zero. Dividing by the first
row of the matrix produces:
1 1 1
q0cot(q0/2) qcoth(q/2) q*coth(q*/2)
q0
2+K2
( )
2q2−K2
( )
2q*2 −K2
( )
2
#
$
%
%
%
&
‘
(
(
(
A
B
C
#
$
%
%
%
&
‘
(
(
(
=0
. (7)
From (2) and definition of s given above:
q0
2=K2(s−1)
,
q0
2+K2
( )
2=K2(s−1) −K2
( )
2=K4s2
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Fluid Mechanics, 6th Ed. Kundu, Cohen, and Dowling
Exercise 11.8. Consider the centrifugal instability problem of Section 11.6. Making the narrow-
gap approximation, work out the algebra of going from (11.50) to (11.51).
Solution 11.8. The perturbation equations (11.50) are:
∂
uR
∂
t−2U
ϕ
u
ϕ
R=−1
ρ
∂
p
∂
R+
ν
∇2uR−uR
R2
(
)
* +
,
–
,
∂
u
ϕ
∂
t+
dU
ϕ
dR +
U
ϕ
R
$
%
&
‘
(
)
uR=
ν
∇2u
ϕ
−u
ϕ
R2
$
%
&
‘
(
)
,
∂
uz
∂
t=−1
ρ
∂
p
∂
z+
ν
∇2uz
, and
∂
∂
R
RuR
( )
+
∂
uz
∂
z=0
. (1)
Substituting in
Eliminating w between the third and fourth equations of set (2) produces:
ν
k2
d
dR +1
R
#
$
% &
‘
( d
dR −k2−
σ
ν
#
$
%
&
‘
( d
dR +1
R
#
$
% &
‘
(
u=ˆ
p
.
Inserting this equation for
ˆ
p
into the first equation of set (2), and working on the algebra
eventually leads to:
where U
ϕ
= Ar + B/r. It is convenient to make the transformation
2AR2
2
ν
u→u
,
so that the equations take the more convenient forms: