Unlock document.
This document is partially blurred.
Unlock all pages and 1 million more documents.
Get Access
PROBLEM 18.141* (Continued)
Given data:
217
011
g
a
φ
= ⋅
Substituting into Eq. (6),
2
2
17 10 cos cos 1.7sin
gg
βββ
max
PROBLEM 18.142*
A homogeneous sphere of mass m and radius a is welded to a rod AB of negligible
mass, which is held by a ball-and-socket support at A. The sphere is released in the
position
0
β
=
with a rate of precession
0
φφ
=
with no spin or nutation. Knowing
that the largest value of
β
in the ensuing motion is 30°, determine (a) the rate of
precession
0
φ
of the sphere in its initial position, (b) the rates of precession and spin
when
30 .
β
= °
0
PROBLEM 18.142* (Continued)
We now write
constant:
z
H=
2
2( sin ) constant
5
z
H ma
ψφ β
= −=
222
22 2 2 2 2 2
1()
2
1 22 22 2
cos ( sin )
25 5 5
xx yy zz
TI I I
T ma ma ma
ωωω
φ β β ψφ β
= ++
= + +−
22 2 2 2 2 2
constant: cos ( sin ) 2 sin constant
25 5 5
T V ma ma ma mga
φ β β ψφ β β
+= + + − − =
From the initial conditions
0
, 0, 0,
φφψ β β
= = = =
we find that the constant is
22
11
0
5
.ma
φ
Thus,
22 2 2 2
g
0
Eq. (3):
22 2 2
0
11 cos 11 10 sin 11
g
a
φ ββ βφ
+− =
(3 )
′
Solving
(1 )
′
for
,
φ
2
2
02
1 10
1 sin
11
cos
g
a
φβ
θ
−=
2
2
10 cos
11 sin
g
a
β
PROBLEM 18.142* (Continued)
(a) Making
30
β
= °
in Eq. (6), we have
2
10 0.75 15
gg
15
g
PROBLEM 18.143*
Consider a rigid body of arbitrary shape which is attached at its mass center O
and subjected to no force other than its weight and the reaction of the support at
O.
(a) Prove that the angular momentum
O
H
of the body about the fixed Point O is
constant in magnitude and direction, that the kinetic energy T of the body is
constant, and that the projection along
O
H
of the angular velocity
ω
of the body
is constant.
(b) Show that the tip of the vector
ω
describes a curve on a fixed plane in space
(called the invariable plane), which is perpendicular to HO and at a distance
2/
O
TH
from O.
(c) Show that with respect to a frame of reference attached to the body and
coinciding with its principal axes of inertia, the tip of the vector
ω
appears to
describe a curve on an ellipsoid of equation
222
2 constant
xx yy zz
III T
ωωω
++==
The ellipsoid (called the Poinsot ellipsoid ) is rigidly attached to the body and is
of the same shape as the ellipsoid of inertia, but of a different size.
OO
Since
0, 0.
OO
Σ= =MH
constant (1)
O
=H
Conservation of energy:
constantTV+=
For a rigid body rotating about Point O,
222
1
O
cos
OO
H
ωβ
⋅=Hω
The projection of
ω
along
is cos
O
ωβ
H
2
cos constant
O
T
H
ωβ
= =
cos constant (3)
ωβ
=
PROBLEM 18.143* (Continued)
(b)
cos
ωβ
is the perpendicular distance from the invariable plane. This distance is equal to
2
.
O
T
H
(c) For a frame of reference attached to the body, the moments of inertia with respect of orthogonal axes
PROBLEM 18.144*
Referring to Problem 18.143, (a) prove that the Poinsot ellipsoid is tangent to the
invariable plane, (b) show that the motion of the rigid body must be such that the
Poinsot ellipsoid appears to roll on the invariable plane. [Hint: In part a, show that
the normal to the Poinsot ellipsoid at the tip of
ω
is parallel to
.
O
H
It is recalled
that the direction of the normal to a surface of equation
( , , ) constantFx y z =
at a
Point P is the same as that of grad F at Point P.]
PROBLEM 18.145*
Using the results obtained in Problems 18.143 and 18.144, show that for an
axisymmetrical body attached at its mass center O and under no force other than
its weight and the reaction at O, the Poinsot ellipsoid is an ellipsoid of revolution
and the space and body cones are both circular and are tangent to each other.
Further show that (a) the two cones are tangent externally, and the precession is
direct, when
,II
′
<
where
I
and
I′
denote, respectively, the axial and transverse
moment of inertia of the body, (b) the space cone is inside the body cone, and the
precession is retrograde, when
.II
′
>
PROBLEM 18.146*
Refer to Problems 18.143 and 18.144.
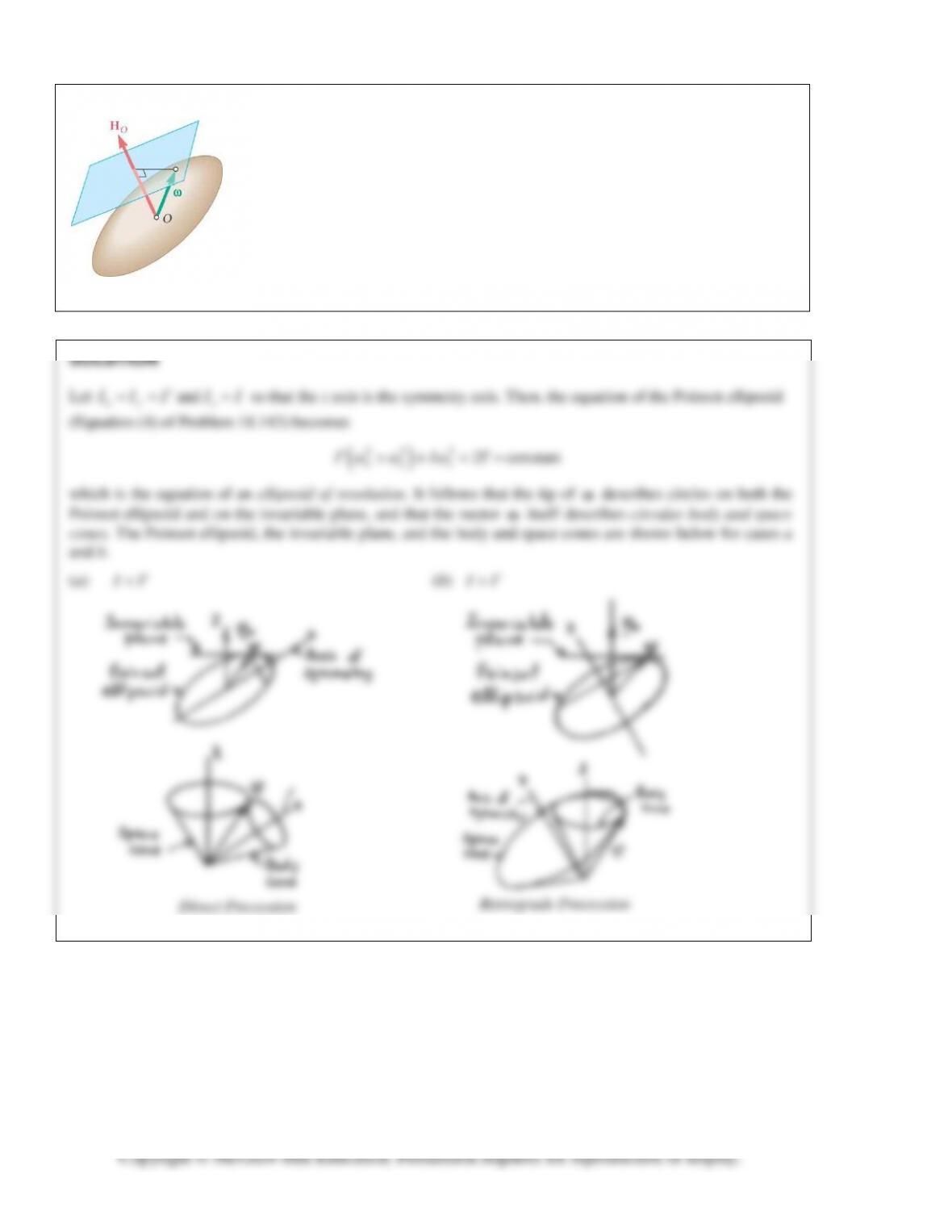
(a) Show that the curve (called polhode) described by the tip of the vector
ω
with respect to a frame of reference coinciding with the principal axes of
inertia of the rigid body is defined by the equations
222
2 constant
xx yy zz
III T
ωωω
++==
(1)
22 22 22 2
constant
xx yy zz O
IIIH
ωωω
++==
(2)
and that this curve can, therefore, be obtained by intersecting the Poinsot
ellipsoid with the ellipsoid defined by Eq. (2).
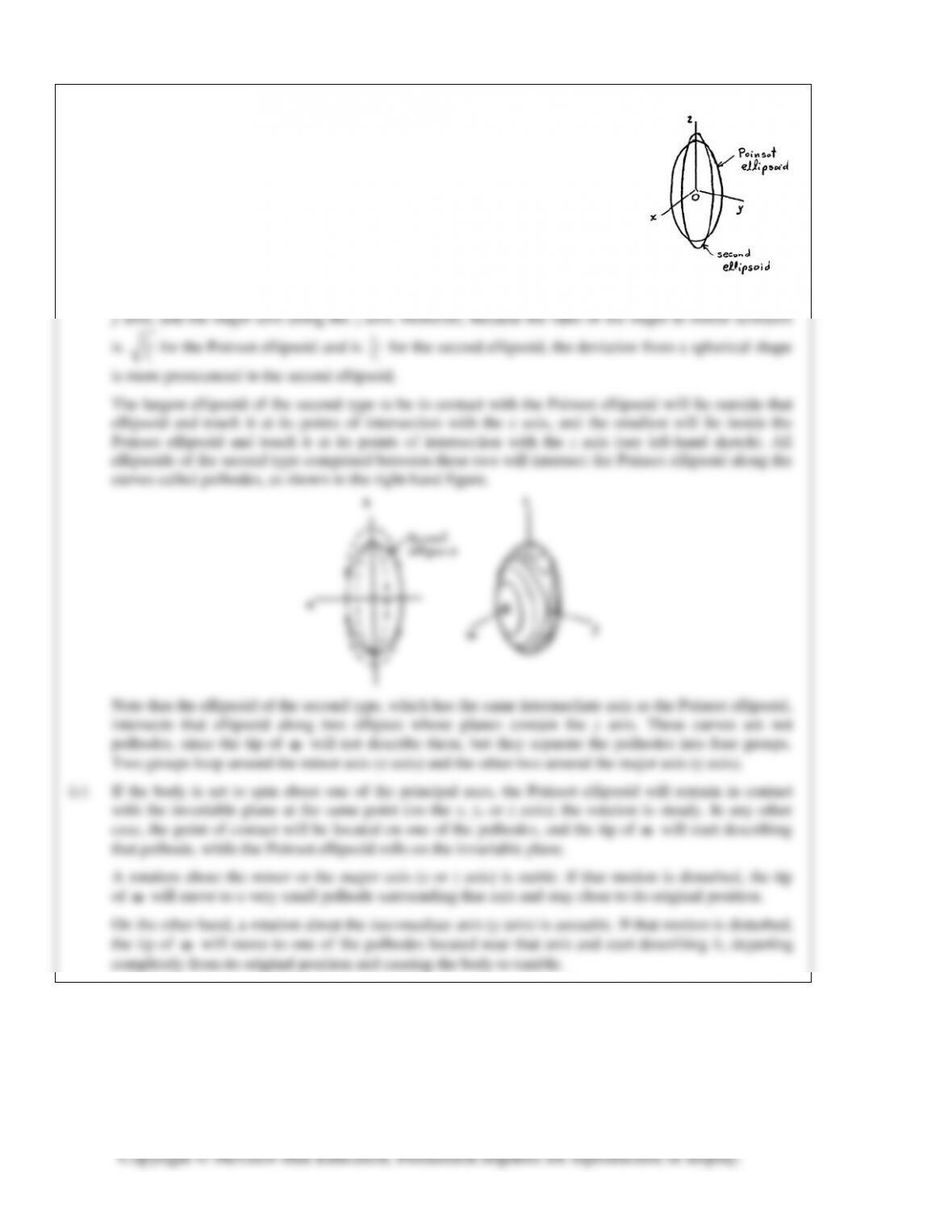
(b) Further show, assuming
,
xyz
III>>
that the polhodes obtained for
various values of
O
H
have the shapes indicated in the figure.
(c) Using the result obtained in part b, show that a rigid body under no force
can rotate about a fixed centroidal axis if, and only if, that axis coincides
with one of the principal axes of inertia of the body, and that the motion will
be stable if the axis of rotation coincides with the major or minor axis of the
Poinsot ellipsoid (z or x axis in the figure) and unstable if it coincides with
the intermediate axis (y axis).
PROBLEM 18.146* (Continued)
(b) Assume
.
xyz
III>>
Then
111 2 2 2
and .abc a b c<< <<
Thus, for both ellipsoids, the minor axis is directed along the x axis, the intermediate axis along the
PROBLEM 18.147
Three 25-lb rotor disks are attached to a shaft which rotates
at 720 rpm. Disk A is attached eccentrically so that its mass
center is
1
4
in.
from the axis of rotation, while disks B and C
are attached so that their mass centers coincide with the axis
of rotation. Where should 2-lb weights be bolted to disks B
and C to balance the system dynamically?
PROBLEM 18.147 (Continued)
48
PROBLEM 18.148
A homogeneous disk of mass
5 kgm=
rotates at the constant
rate
18 rad/s
ω
=
with respect to the bent axle ABC, which
itself rotates at the constant rate
2
3 rad/s
ω
=
about the y axis.
Determine the angular momentum
C
H
of the disk about its
center C.
SOLUTION
Using frame
:Cxyz
′′′
2
2
21
2
21
1
4
1
1( 2)
4
xy
z
Cy z
I I mr
I mr
II
mr
ωω
ωω
′′
′
′′
= =
=
= +
= +
H jk
jk
2
1(5 kg)(0.25 m) [(3 rad/s) 2(8 rad/s) ]
4
C
= +H jk
22
(0.234 kg m /s) (1.250 kg m /s)
C
= ⋅+ ⋅H jk
PROBLEM 18.149
A rod of uniform cross section is used to form the shaft shown.
Denoting by m the total mass of the shaft and knowing that the
shaft rotates with a constant angular velocity
ω
, determine
(a) the angular momentum
G
H
of the shaft about its mass
center G, (b) the angle formed by HG and the axis AB, (c) the
angular momentum of the shaft about Point A.
G
PROBLEM 18.149 (Continued)
(b)
Angle formed by and the axis .
G
ABH
2
PROBLEM 18.150
A uniform rod of mass m and length 5a is bent into the shape shown and is
suspended from a wire attached at Point B. Knowing that the rod is hit at Point
A in the negative y direction and denoting the corresponding impulse by
( ),Ft−∆j
determine immediately after the impact (a) the velocity of the mass
center G, (b) the angular velocity of the rod.
5m
( )
222
1 11
12 4 4
aaa× ++
( )
22 2
11
12 4
aa a× ++
54
52
m
2
0.35ma
2
0.66667ma
2
0.75ma
2
0.3ma−
2 22
11 11 0.2
52 52
xz
I ma ma ma
=+=
2 22
11 11 0.1
54 54
yz
I ma ma ma
= −+ −=−
Angular momentum about the mass center.
222
( ) 0.35 0.3 0.2
G x x x xy y xz z x y z
H I I I ma ma ma
ωωω ω ω ω
=−−= + −
2 22
( ) 0.3 0.66667 0.1
G y xy x y y yz z x y z
H I I I ma ma ma
ωω ω ω ω ω
=−+− = + +
22 2
( ) 0.2 0.1 0.75
Gz xzx yzz zz x y z
H I I I ma ma ma
ω ωω ω ω ω
=−− +=− + +
Σ
PROBLEM 18.150 (Continued)
Constraint of the supporting cable:
0
y
v=
Impulse-momentum principle: Before impact,
0, 0.
G
= =vH
(a) Linear momentum:
()t Tt m∆+∆=F jv
Resolve into components.
0 , 0, 0
xz
mv F t T t mv= −∆+∆= =j
0,
x
v=
,Tt Ft∆= ∆
0
z
v=
0=v
(b) Angular momentum, moments about G:
/AG G
t× ∆=rFH
[( )]( ) () () ()
2Gx Gy Gz
aa Ft aFt H H H
− ×− ∆ = ∆ = + +
ji j k i j k
Using expressions for
( ) , ( ) ,
Gx Gy
HH
and
()
Gz
H
and resolving into components,
222
: 0 0.35 0.3 0.2
xyz
ma ma ma
ωωω
= +−i
2 22
: 0 0.3 0.66667 0.1
x yz
ma ma ma
ω ωω
=++j
22 2
: 0.2 0.1 0.75
xy z
aF t ma ma ma
ωω ω
∆=− + +k
Solving,
() () ()
2.5 , 1.454 , 2.19
xy z
Ft Ft Ft
ma ma ma
ωω ω
∆ ∆∆
==−=
Ft
ma
∆
i jk
