Stewart_Calc_7ET ch09sec04
MULTIPLE CHOICE
1. Suppose that a population develops according to the logistic equation
,
where t is measured in weeks. What is the carrying capacity?
a.
b.
c.
d.
e.
2. Suppose that a population grows according to a logistic model with carrying capacity
and per year. Choose the logistic differential equation for these data.
a.
b.
c.
d.
e.
3. One model for the spread of an epidemic is that the rate of spread is jointly proportional to
the number of infected people and the number of uninfected people. In an isolated town of
inhabitants, people have a disease at the beginning of the week and have it
at the end of the week. How long does it take for of the population to be infected?
a.
b.
c.
d.
e.
4. A sum of is invested at interest. If is the amount of the investment at
time t for the case of continuous compounding, write a differential equation and an initial
condition satisfied by .
a.
b.
c.
d.
e.
5. Let c be a positive number. A differential equation of the form
where k is a positive constant is called a doomsday equation because the exponent in the
expression is larger than the exponent 1 for natural growth. An especially prolific
breed of rabbits has the growth term . If such rabbits breed initially and the warren
has rabbits after months, then when is doomsday?
a.
b.
c.
d.
e.
6. The population of the world was about 5.3 billion in 1990. Birth rates in the 1990s range
from 35 to 40 million per year and death rates range from 15 to 20 million per year. Let’s
assume that the carrying capacity for world population is 100 billion. Use the logistic model
to predict the world population in the 2,450 year. Calculate your answer in billions to one
decimal place. (Because the initial population is small compared to the carrying capacity,
you can take k to be an estimate of the initial relative growth rate.)
a.
78.3 billion
b.
24.1 billion
c.
17.1 billion
d.
59.2 billion
e.
32.9 billion
7. A common inhabitant of human intestines is the bacterium Escherichia coli. A cell of this
bacterium in a nutrient-broth medium divides into two cells every . The initial
population of a culture is cells. Find the number of cells after hours.
a.
b.
c.
d.
e.
8. Consider a population with constant relative birth and death rates and ,
respectively, and a constant emigration rate m, where , and . Then
the rate of change of the population at time t is modeled by the differential equation
where
.
Find the solution of this equation with the rate of change of the population at time that
satisfies the initial condition .
a.
b.
c.
d.
e.
f.
none of these
9. The population of the world was about 5.3 billion in 1990. Birth rates in the 1990s range
from 35 to 40 million per year and death rates range from 15 to 20 million per year. Let’s
assume that the carrying capacity for world population is 100 billion. Use the logistic model
to predict the world population in the 2,450 year. Calculate your answer in billions to one
decimal place. (Because the initial population is small compared to the carrying capacity,
you can take k to be an estimate of the initial relative growth rate.)
a.
24.1 billion
b.
78.3 billion
c.
59.2 billion
d.
17.1 billion
e.
32.9 billion
NUMERIC RESPONSE
1. The Pacific halibut fishery has been modeled by the differential equation
where is the biomass (the total mass of the members of the population) in kilograms at
time t (measured in years), the carrying capacity is estimated to be and
per year. If , find the biomass a year later.
2. One model for the spread of an epidemic is that the rate of spread is jointly proportional to
the number of infected people and the number of uninfected people. In an isolated town of
inhabitants, people have a disease at the beginning of the week and have it
at the end of the week. How long does it take for of the population to be infected?
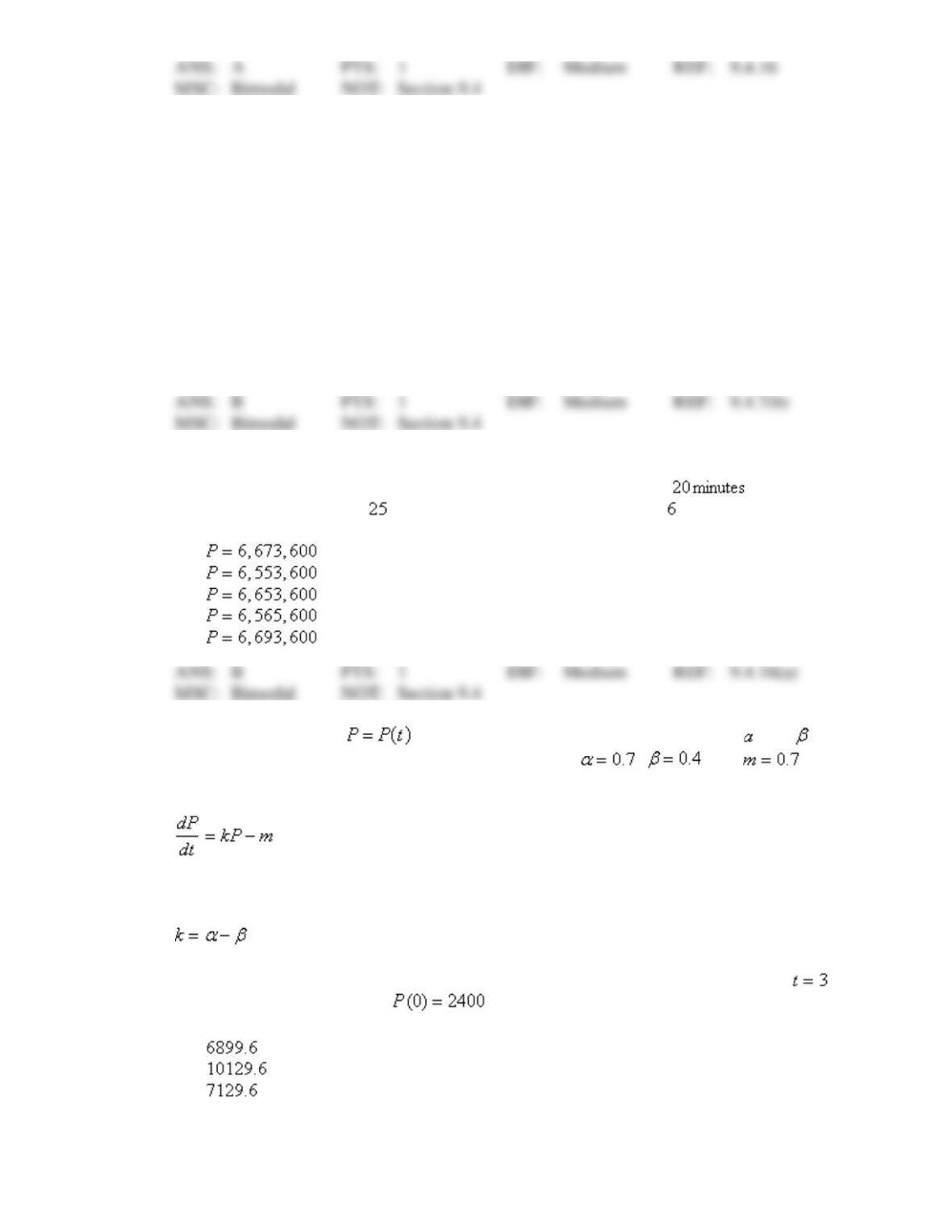
3. Let .
What are the equilibrium solutions?
4. Consider the differential equation
as a model for a fish population, where t is measured in weeks and c is a constant. For what
values of c does the fish population always die out?
5. The rate of change of atmospheric pressure P with respect to altitude h is proportional to P
provided that the temperature is constant. At the pressure is at sea level
and at . What is the pressure at an altitude of ?
6. One model for the spread of a rumor is that the rate of spread is proportional to the product
of the fraction of the population who have heard the rumor and the fraction who have not
heard the rumor. Let’s assume that the constant of proportionality is . Write a
differential equation that is satisfied by y.
7. Biologists stocked a lake with fish and estimated the carrying capacity (the maximal
population for the fish of that species in that lake) to be . The number of fish tripled in
the first year. Assuming that the size of the fish population satisfies the logistic equation,
find an expression for the size of the population after t years.
8. Suppose that a population grows according to a logistic model with carrying capacity
and per year. Write the logistic differential equation for these data.
9. Let c be a positive number. A differential equation of the form
where k is a positive constant, is called a doomsday equation because the exponent in the
expression is larger than the exponent 1for natural growth. An especially prolific
breed of rabbits has the growth term . If such rabbits breed initially and the warren
has rabbits after months, then when is doomsday?