CHAPTER 12
Introduction to Binomial Trees
Practice Questions
Problem 12.8.
Consider the situation in which stock price movements during the life of a European option
are governed by a two-step binomial tree. Explain why it is not possible to set up a position
in the stock and the option that remains riskless for the whole of the life of the option.
The riskless portfolio consists of a short position in the option and a long position in
shares. Because
changes during the life of the option, this riskless portfolio must also
change.
Problem 12.9.
A stock price is currently $50. It is known that at the end of two months it will be either $53
or $48. The risk-free interest rate is 10% per annum with continuous compounding. What is
the value of a two-month European call option with a strikeprice of $49? Use no-arbitrage
arguments.
At the end of two months the value of the option will be either $4 (if the stock price is $53) or
$0 (if the stock price is $48). Consider a portfolio consisting of:
shares
1 option
+
−
The value of the portfolio is either
48
or
53 4−
in two months. If
48 53 4 = −
i.e.,
08 =
the value of the portfolio is certain to be 38.4. For this value of
the portfolio is therefore
riskless. The current value of the portfolio is:
0 8 50 f −
where
f
is the value of the option. Since the portfolio must earn the risk-free rate of interest
0 10 2 12
(0 8 50 ) 38 4fe
− =
i.e.,
2 23f=
The value of the option is therefore $2.23.
This can also be calculated directly from equations (12.2) and (12.3).
1 06u=
,
0 96d=
so
that
0 10 2 12 0 96 0 5681
1 06 0 96
e
p −
= =
−
and
0 10 2 12 0 5681 4 2 23fe
−
= =
Problem 12.10.
A stock price is currently $80. It is known that at the end of four months it will be either $75
or $85. The risk-free interest rate is 5% per annum with continuous compounding. What is
the value of a four-month European put option with a strikeprice of $80? Use no-arbitrage
arguments.
At the end of four months the value of the option will be either $5 (if the stock price is $75)
or $0 (if the stock price is $85). Consider a portfolio consisting of:
shares
1 option
−
+
(Note: The delta,
of a put option is negative. We have constructed the portfolio so that it is
+1 option and
−
shares rather than
1−
option and
+
shares so that the initial investment
is positive.)
The value of the portfolio is either
85−
or
75 5− +
in four months. If
85 75 5− = − +
i.e.,
05 = −
the value of the portfolio is certain to be 42.5. For this value of
the portfolio is therefore
riskless. The current value of the portfolio is:
0 5 80 f +
where
f
is the value of the option. Since the portfolio is riskless
0 05 4 12
(0 5 80 ) 42 5fe
+ =
i.e.,
1 80f=
The value of the option is therefore $1.80.
This can also be calculated directly from equations (12.2) and (12.3).
1 0625u=
,
0 9375d=
so that
0 05 4 12 0 9375 0 6345
1 0625 0 9375
e
p −
= =
−
1 0 3655p− =
and
0 05 4 12 0 3655 5 1 80fe
−
= =
Problem 12.11.
A stock price is currently $40. It is known that at the end of three months it will be either $45
or $35. The risk-free rate of interest with quarterly compounding is 8% per annum. Calculate
the value of a three-month European put option on the stock with an exercise price of $40.
Verify that no-arbitrage arguments and risk-neutral valuation arguments give the same
answers.
At the end of three months the value of the option is either $5 (if the stock price is $35) or $0
(if the stock price is $45).
Consider a portfolio consisting of:
shares
1 option
−
+
(Note: The delta,
, of a put option is negative. We have constructed the portfolio so that it
is +1 option and
−
shares rather than
1−
option and
+
shares so that the initial
investment is positive.)
The value of the portfolio is either
35 5− +
or
45−
. If:
35 5 45− + = −
i.e.,
05 = −
the value of the portfolio is certain to be 22.5. For this value of
the portfolio is therefore
riskless. The current value of the portfolio is
40 f− +
where f is the value of the option. Since the portfolio must earn the risk-free rate of interest
(40 0 5 ) 1 02 22 5f + =
Hence
2 06f=
i.e., the value of the option is $2.06.
This can also be calculated using risk-neutral valuation. Suppose that
p
is the probability of
an upward stock price movement in a risk-neutral world. We must have
45 35(1 ) 40 1 02pp+ − =
i.e.,
10 5 8p=
or:
0 58p=
The expected value of the option in a risk-neutral world is:
0 0 58 5 0 42 2 10 + =
This has a present value of
2 10 2 06
1 02
=
This is consistent with the no-arbitrage answer.
Problem 12.12.
A stock price is currently $50. Over each of the next two three-month periods it is expected to
go up by 6% or down by 5%. The risk-free interest rate is 5% per annum with continuous
compounding. What is the value of a six-month European call option with a strike price of
$51?
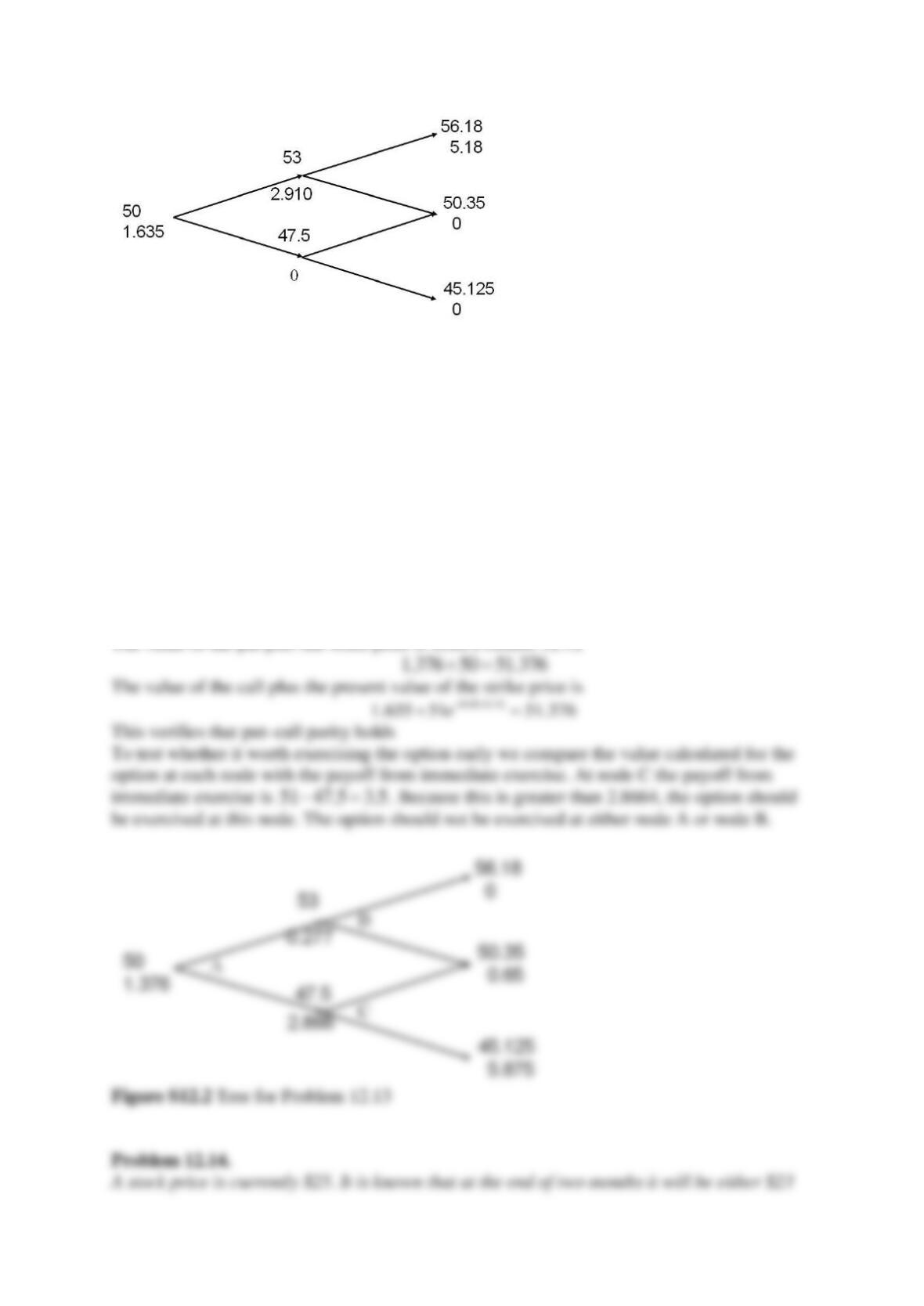
A tree describing the behavior of the stock price is shown in Figure S12.1. The risk-neutral
probability of an up move, p, is given by
0 05 3 12 0 95 0 5689
1 06 0 95
e
p −
= =
−
There is a payoff from the option of
56 18 51 5 18 − =
for the highest final node (which
corresponds to two up moves) zero in all other cases. The value of the option is therefore
2 0 05 6 12
5 18 0 5689 1 635e−
=
This can also be calculated by working back through the tree as indicated in Figure S12.1.
The value of the call option is the lower number at each node in the figure.
Figure S12.1 Tree for Problem 12.12
Problem 12.13.
For the situation considered in Problem 12.12, what is the value of a six-month European put
option with a strike price of $51? Verify that the European call and European put prices
satisfy put–call parity. If the put option were American, would it ever be optimal to exercise it
early at any of the nodes on the tree?
The tree for valuing the put option is shown in Figure S12.2. We get a payoff of
51 50 35 0 65− =
if the middle final node is reached and a payoff of
51 45 125 5 875− =
if
the lowest final node is reached. The value of the option is therefore
2 0 05 6 12
(0 65 2 0 5689 0 4311 5 875 0 4311 ) 1 376e−
+ =
This can also be calculated by working back through the tree as indicated in Figure S12.2.
